Radius of a capillary is . A liquid of weight may remain in the capillary, then the surface tension of liquid will be:
5N/m
50 N/m
The correct Answer is:B
To find the surface tension of the liquid in the capillary, we can use the formula that relates the weight of the liquid to the surface tension and the radius of the capillary.
1. Identify the given values:
- Radius of the capillary, r=2×10−3m
- Weight of the liquid, F=6.28×10−4N
2. Recall the formula for the force due to surface tension:
The force due to surface tension can be expressed as:
F=T⋅2πr
where T is the surface tension and r is the radius of the capillary.
3. Rearrange the formula to solve for surface tension T:
T=F2πr
4. Substitute the known values into the equation:
T=6.28×10−42π(2×10−3)
5. Calculate 2π:
Using π≈3.14:
2π≈2×3.14=6.28
6. Substitute 2π back into the equation:
T=6.28×10−46.28×(2×10−3)
7. Simplify the equation:
T=6.28×10−46.28×2×10−3=12×10−1
8. Calculate the final value of surface tension:
T=0.5×10−1=5×10−2N/m
Final Answer:
The surface tension of the liquid is:
T=5×10−2N/m
- Radius of the capillary, r=2×10−3m
- Weight of the liquid, F=6.28×10−4N
The force due to surface tension can be expressed as:
F=T⋅2πr
where T is the surface tension and r is the radius of the capillary.
T=F2πr
T=6.28×10−42π(2×10−3)
Using π≈3.14:
2π≈2×3.14=6.28
T=6.28×10−46.28×(2×10−3)
T=6.28×10−46.28×2×10−3=12×10−1
T=0.5×10−1=5×10−2N/m
The surface tension of the liquid is:
T=5×10−2N/m
Topper's Solved these Questions
- FLUID MECHANICSBook:RESONANCE ENGLISHChapter:FLUID MECHANICSExercise:Examples
Explore 22 Videos
- FLUID MECHANICSBook:RESONANCE ENGLISHChapter:FLUID MECHANICSExercise:Board Level Exercise
Explore 18 Videos
- ELECTROSTATICSBook:RESONANCE ENGLISHChapter:ELECTROSTATICSExercise:Exercise
Explore 52 Videos
- FULL TEST 1Book:RESONANCE ENGLISHChapter:FULL TEST 1Exercise:Exercise
Explore 30 Videos
Similar Questions
It is said that a liquid rises or is depressed in a capillary due to the surface tension. If a liquid neither rises nor depresses in a capillary, can we conclude that the surface tension of the liquid is zero ?
View SolutionA glass capillary sealed at the upper end is of length 0.11m and internal diameter 2×105m. The tube is immersed vertically into a liquid of surface tension 5.06×10−2N/m. To what length the capillary has to be immersed so that the liquid level inside and outside the capillary becomes the same. What will happen to water level inside the capillary if the seal is now broken?
View SolutionThe ring of radius 1 m is lying on the surface of liquid. It is lifted. It is lifted from the liquid surface by a force of 4 newtons in such a way that the liquid film in it remains intact. The surface tension of liquid will be
View SolutionA liquid can rise to a height of h in a capillary tube of length l (l>h). The tube filled with the liquid is kept horizontal with its two ends open. Now the tube is kept vertical with the liquid in it. Some liquid flows out. Find the height of the liquid remaining in the tube if the surface tension of the liquid is σ.
View SolutionLower end of a capillary tube of radius 10−3 m is dipped vertically into a liquid. Surface tension of liquid is 0.5 N/m and specific gravity of liquid is 5. Contact angle between liquid and material of capillary tube is 120∘. Choose the correct options (use g = 10m/s2)
View Solution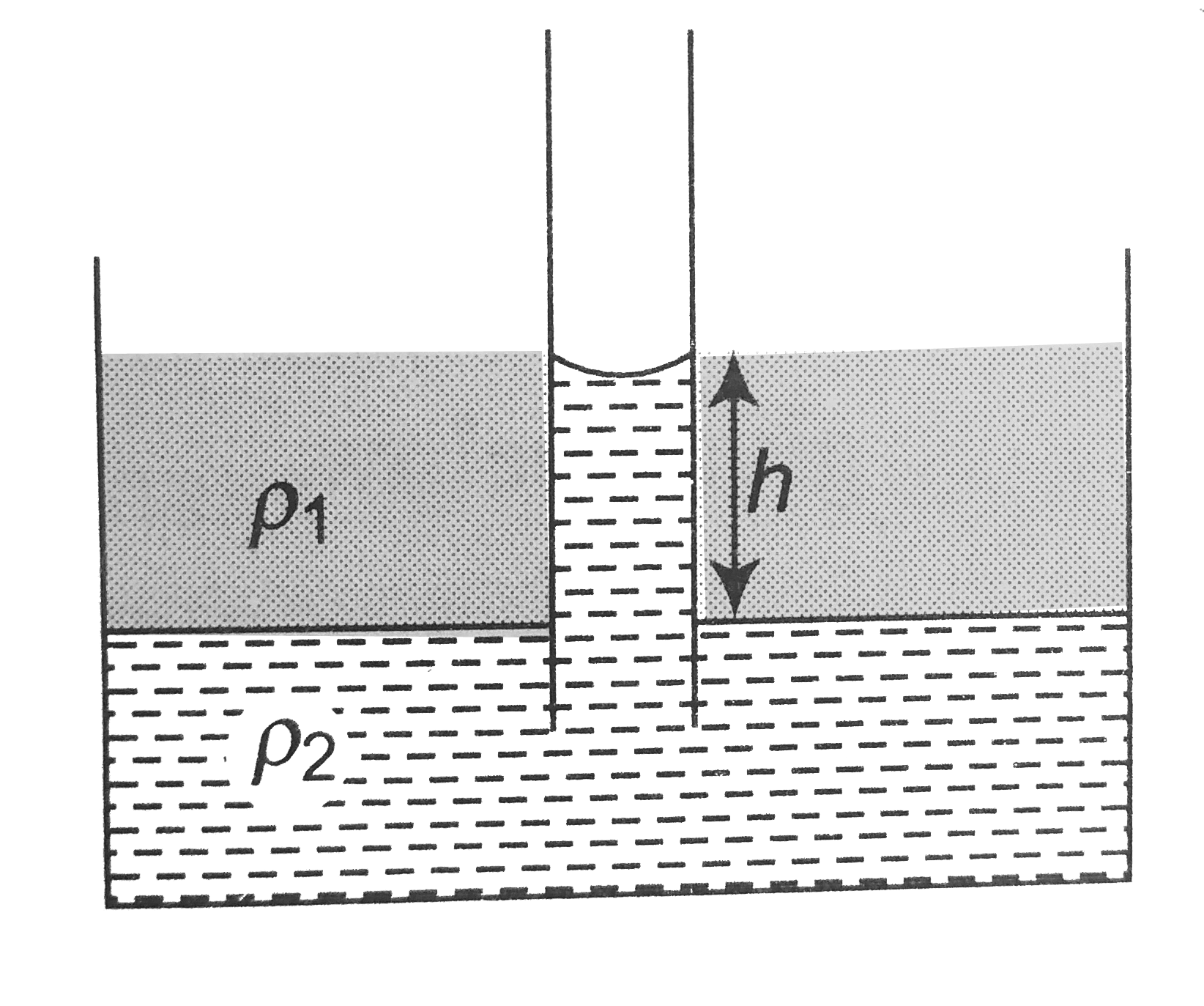
A container is partially filled with a liquid of density ρ2 A capillary tube of radius r is vertically inserted in this liquid. Now another liquid of density ρ1(ρ1<ρ2) is slowly poured in the container to a height h as shown. There is only denser liquid in the capillary tube. The rise of denser liquid in the capillary tube is also h. Assuming zero contact angle, the surface tension of heavier liquid is
View SolutionDerive an expression for the height of capillary rise between two parrallel plates dipping in a liquid of density σ separated by a distance d. The surface tension of the liquid is T.
View SolutionA student writes the equation for the capillary rise of a liquid in a tube as h=ρg2Tcosθ, where r is the radius of the capillary tube, ρ is the density of liquid, T is the surface tension and θ is the angle of contact. Check the correctness of the equation using dimensional analysis?
View SolutionIn a device designed by Academician Rebinder the surface tension is determnined from the pressure difference required to form a bubble of air at the end of a capillary immersed in the liquid being investigated (fig). Calculated the surface tension if the radius of the capillary is r=1mm and the difference in the pressures during bubble formation is ΔP=14mm of water column. The end of the capillary is near the surface of the liquid.
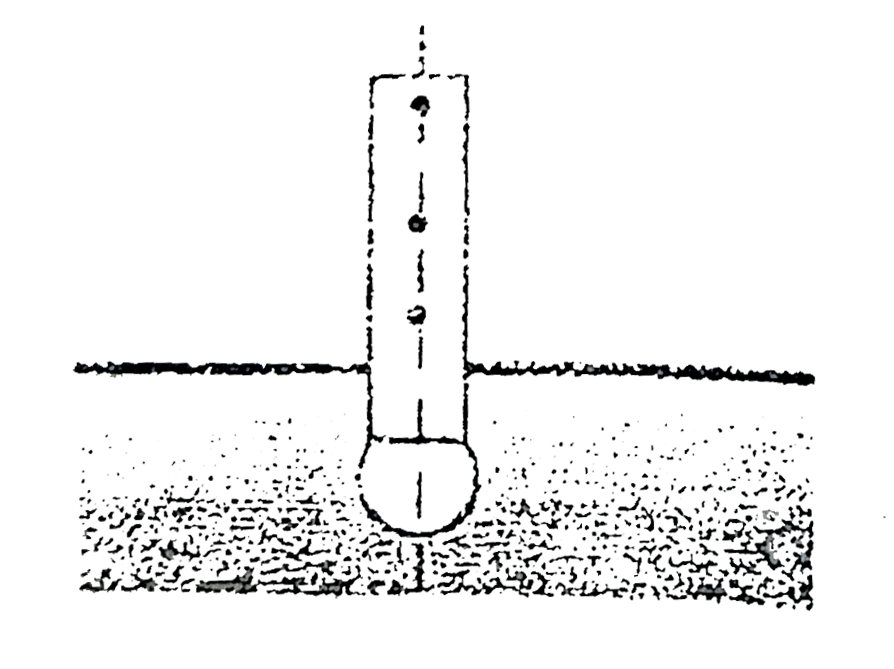
View SolutionThe sap in trees, which consists mainly of water in summer, rises in a system of capillaries of radius r=2.5×10−5m. The surface tension of sap is T=7.28×10−2Nm−1 and the angle of contact is 0∘. Does surface tension alone ac count for the supply of water to the top of all trees ?
View Solution
