An insulated conductor initiallly free from charge is charged by repeated contacts with a plate which after each contact is replenished to a charge Q. If q is the charge on the conductor after first operation prove that the maximum charge which can be given to the conductor in this way is QqQ−q
To solve the problem, we need to analyze the charging process of the insulated conductor when it comes into contact with a charged plate. Let's break down the steps:
Step 1: Initial Setup
Let the insulated conductor have a capacitance C1 and the plate have a capacitance C2. Initially, the conductor is uncharged.
Step 2: First Contact
When the charged plate, which has a charge Q, comes into contact with the insulated conductor, a charge q is transferred to the conductor. After the first operation, the charge on the conductor is q.
Step 3: Potential Equilibrium
At the moment of contact, the potential of the conductor V1 and the potential of the plate V2 must be equal for charge to stop flowing. The potential of the conductor can be expressed as:
V1=qC1
The potential of the plate after losing charge q becomes:
V2=Q−qC2
Setting V1=V2 gives us:
qC1=Q−qC2
Step 4: Rearranging the Equation
From the equation above, we can rearrange it to find the relationship between q, Q, C1, and C2:
q⋅C2=(Q−q)⋅C1
This simplifies to:
q⋅C2+q⋅C1=Q⋅C1
q(C1+C2)=Q⋅C1
Thus, we can express q in terms of Q:
q=Q⋅C1C1+C2
Step 5: Repeated Contacts
After the first contact, the conductor regains its charge q and the process can be repeated. Each time the plate has a charge Q and the conductor can take on more charge until it reaches a maximum charge Qm.
Step 6: Maximum Charge Calculation
Let Qm be the maximum charge on the conductor after multiple contacts. The potential must again be equal:
QmC1=Q−QmC2
Rearranging gives:
Qm⋅C2=(Q−Qm)⋅C1
This simplifies to:
Qm⋅C2+Qm⋅C1=Q⋅C1
Qm(C1+C2)=Q⋅C1
Thus, we can express Qm:
Qm=Q⋅C1C1+C2
Step 7: Substitute for Capacitance Ratio
From our earlier relationship, we know that:
C1C2=qQ−q
Substituting this back into our equation for Qm:
Qm=Q⋅qQ−q
Conclusion
The maximum charge that can be given to the conductor in this way is:
Qm=QqQ−q
---
Let the insulated conductor have a capacitance C1 and the plate have a capacitance C2. Initially, the conductor is uncharged.
When the charged plate, which has a charge Q, comes into contact with the insulated conductor, a charge q is transferred to the conductor. After the first operation, the charge on the conductor is q.
At the moment of contact, the potential of the conductor V1 and the potential of the plate V2 must be equal for charge to stop flowing. The potential of the conductor can be expressed as:
V1=qC1
The potential of the plate after losing charge q becomes:
V2=Q−qC2
Setting V1=V2 gives us:
qC1=Q−qC2
From the equation above, we can rearrange it to find the relationship between q, Q, C1, and C2:
q⋅C2=(Q−q)⋅C1
This simplifies to:
q⋅C2+q⋅C1=Q⋅C1
q(C1+C2)=Q⋅C1
Thus, we can express q in terms of Q:
q=Q⋅C1C1+C2
After the first contact, the conductor regains its charge q and the process can be repeated. Each time the plate has a charge Q and the conductor can take on more charge until it reaches a maximum charge Qm.
Let Qm be the maximum charge on the conductor after multiple contacts. The potential must again be equal:
QmC1=Q−QmC2
Rearranging gives:
Qm⋅C2=(Q−Qm)⋅C1
This simplifies to:
Qm⋅C2+Qm⋅C1=Q⋅C1
Qm(C1+C2)=Q⋅C1
Thus, we can express Qm:
Qm=Q⋅C1C1+C2
From our earlier relationship, we know that:
C1C2=qQ−q
Substituting this back into our equation for Qm:
Qm=Q⋅qQ−q
The maximum charge that can be given to the conductor in this way is:
Qm=QqQ−q
Topper's Solved these Questions
- CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - CAPACITORSEXERCISE - Exercise
155 Videos
- CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - CAPACITORSEXERCISE - OBJECTIVE_TYPE
1 Videos
- ATOMSBOOK - DC PANDEY ENGLISHCHAPTER - ATOMSEXERCISE - MEDICAL ENTRANCES GALLERY
42 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Subjective
11 Videos
Similar Questions
An isolated coductor, initially free from charge, is charge by repeated conacts with a plate, which afrer each contact has a charge Q due to some mechanism.If q is the charge on the conductor after the first operation, prove that the maximum charge that can be give to the conductor in this way is Qq/Q−q.
View SolutionSuppose a charge +Q1 is given to the positive plates and a charge −Q2 to the negative plate of a capacitor. What is the "charge on the capacitor"?
View Solution
Knowledge Check
A method for charging a conductor without bringing a charged body in contact with it is called
AMagnetizationBElectrificationCElectrostatic inductionDElectromagnetic induction
In a parallel plate capacitor with plate area A and charge Q, the force on one plate because of the charge on the other is equal to
View SolutionThe charge q flowing in a conductor of uniform cross section, varies with time as q=αt−bt2. Then, the current
View SolutionA dielectric slab is inserted between the plates of a capacitor. The charge on the capacitor is Q and the magnitude of the induced charge on each surface of the dielectric is Q'.
View SolutionWork done in moving a charge q coulomb on the surface of given charged conductor of potential V is
View SolutionA point charge q1 is placed inside cavity 1 and another point charge q2 is inside cavity 2. A point charge q is placed outside the conductor. For the situation described above, answer the following questions.
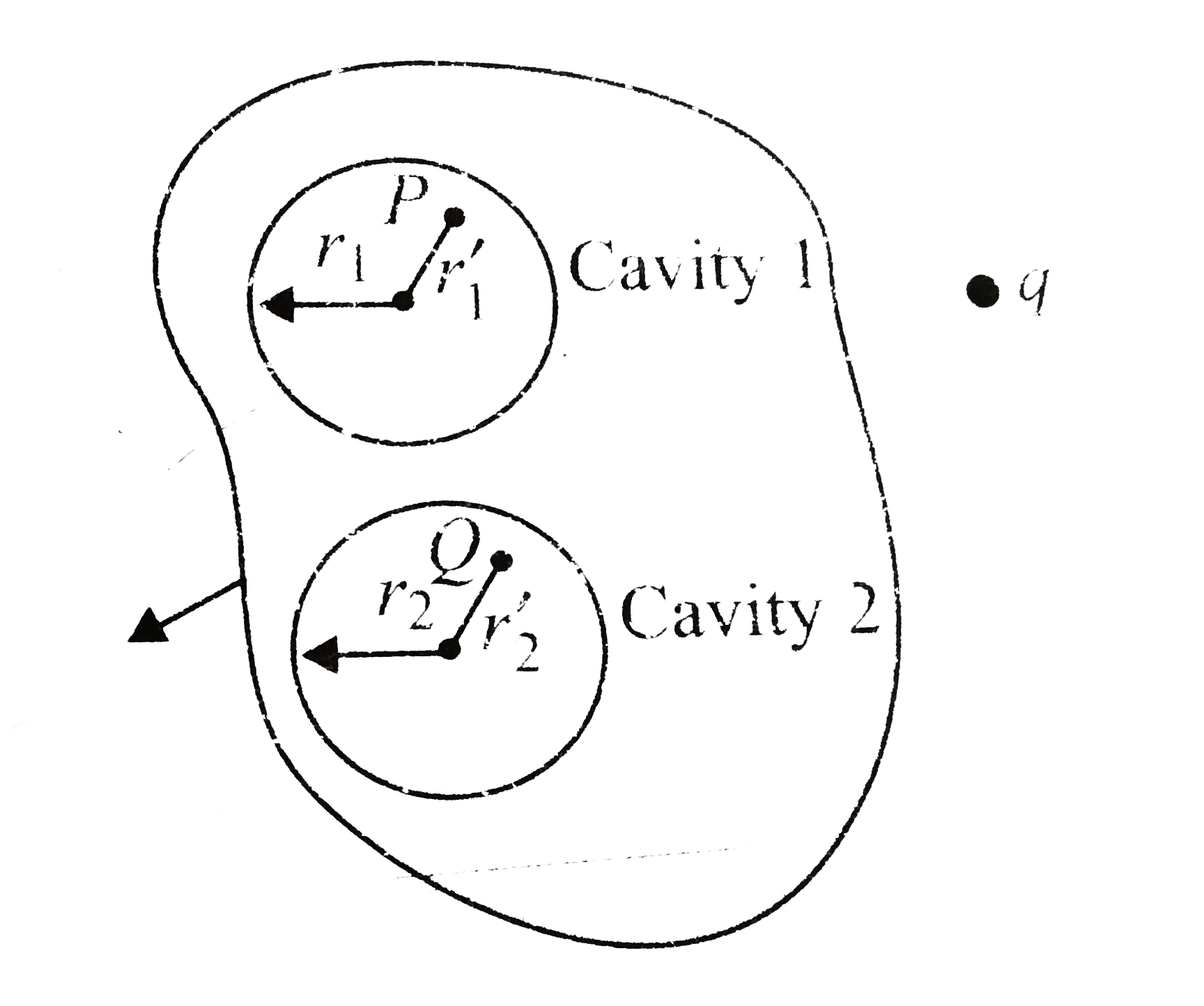
The charge on outer surface of the conductor would be
View SolutionThe charge flowing in a conductor varies with times as Q=at−bt2. Then, the current
View SolutionThe charge flowing in a conductor varies with times as Q=at−bt2. Then, the current
View Solution
