A parallel air capacitance 245μF has a charge of magnitude 0.48μC on each plate. The plates are 0.328mm apart.
(a) What is the potential difference between the plates?
(b) What is the area of each plate?
(c) What is the surface charge density on each plate?
To solve the problem step by step, we will address each part of the question systematically.
Given Data:
- Capacitance, C=245μF=245×10−6F
- Charge, Q=0.48μC=0.48×10−6C
- Distance between plates, d=0.328mm=0.328×10−3m
(a) Finding the Potential Difference Between the Plates
The potential difference V between the plates of a capacitor can be calculated using the formula:
V=QC
Substituting the known values:
V=0.48×10−6245×10−6
Calculating this gives:
V=0.48245V=0.00196V=1.96mV
(b) Finding the Area of Each Plate
The capacitance C of a parallel plate capacitor is also given by the formula:
C=ϵ0Ad
Where:
- ϵ0=8.85×10−12F/m (permittivity of free space)
- A is the area of one plate
- d is the distance between the plates
Rearranging the formula to find the area A:
A=C⋅dϵ0
Substituting the known values:
A=(245×10−6)⋅(0.328×10−3)8.85×10−12
Calculating this gives:
A=(245×0.328)×10−98.85×10−12≈9.08×10−2m2
(c) Finding the Surface Charge Density on Each Plate
The surface charge density σ is defined as the charge per unit area:
σ=QA
Substituting the known values:
σ=0.48×10−69.08×10−2
Calculating this gives:
σ≈5.28×10−6C/m2
Summary of Answers:
(a) The potential difference between the plates is approximately 1.96mV.
(b) The area of each plate is approximately 9.08×10−2m2.
(c) The surface charge density on each plate is approximately 5.28×10−6C/m2.
- Capacitance, C=245μF=245×10−6F
- Charge, Q=0.48μC=0.48×10−6C
- Distance between plates, d=0.328mm=0.328×10−3m
The potential difference V between the plates of a capacitor can be calculated using the formula:
V=QC
V=0.48×10−6245×10−6
Calculating this gives:
V=0.48245V=0.00196V=1.96mV
The capacitance C of a parallel plate capacitor is also given by the formula:
C=ϵ0Ad
Where:
- ϵ0=8.85×10−12F/m (permittivity of free space)
- A is the area of one plate
- d is the distance between the plates
A=C⋅dϵ0
A=(245×10−6)⋅(0.328×10−3)8.85×10−12
Calculating this gives:
A=(245×0.328)×10−98.85×10−12≈9.08×10−2m2
The surface charge density σ is defined as the charge per unit area:
σ=QA
σ=0.48×10−69.08×10−2
Calculating this gives:
σ≈5.28×10−6C/m2
(a) The potential difference between the plates is approximately 1.96mV.
(b) The area of each plate is approximately 9.08×10−2m2.
(c) The surface charge density on each plate is approximately 5.28×10−6C/m2.
Topper's Solved these Questions
- CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - CAPACITORSEXERCISE - OBJECTIVE_TYPE
1 Videos
- CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - CAPACITORSEXERCISE - OBJECTIVE_TYPE
1 Videos
- ATOMSBOOK - DC PANDEY ENGLISHCHAPTER - ATOMSEXERCISE - MEDICAL ENTRANCES GALLERY
42 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Subjective
11 Videos
Similar Questions
One plate of a capacitor of capacitance 2μF has total charge +10μC and its other plate has total charge +40μC. The potential difference between the plates is (in Volt).
View SolutionThe plates of a parallel-plate capacior are given equal positive charges .What will be the potential difference between the plates ? What will be the charges on the facing surfaces and on the outer surfaces ?
View SolutionIn a parallel plate capacitor of capacitor 1μF two plates are given charges 2μC and 4μC the potential difference between the plates of the capacitor is
_2019_CBT2_E01_002_Q01.png)
View SolutionA capacitor has a capacitance of 7.28μF. What amount of charge must be placed on each of its plates to make the potential difference between its plates equAl to 25.0V?
View SolutionThe plates of a parallel plate capacitor are not exactly parallel. The surface charge density is therefore,
View SolutionA parallel plate air capacitor has capcity C distance of separtion between plates is d and potential difference V is applied between the plates force of attraction between the plates of the parallel plate air capacitor is
View SolutionFind the equivalent capacitance between A and B.A: area of each plate, d: separation between the plates.
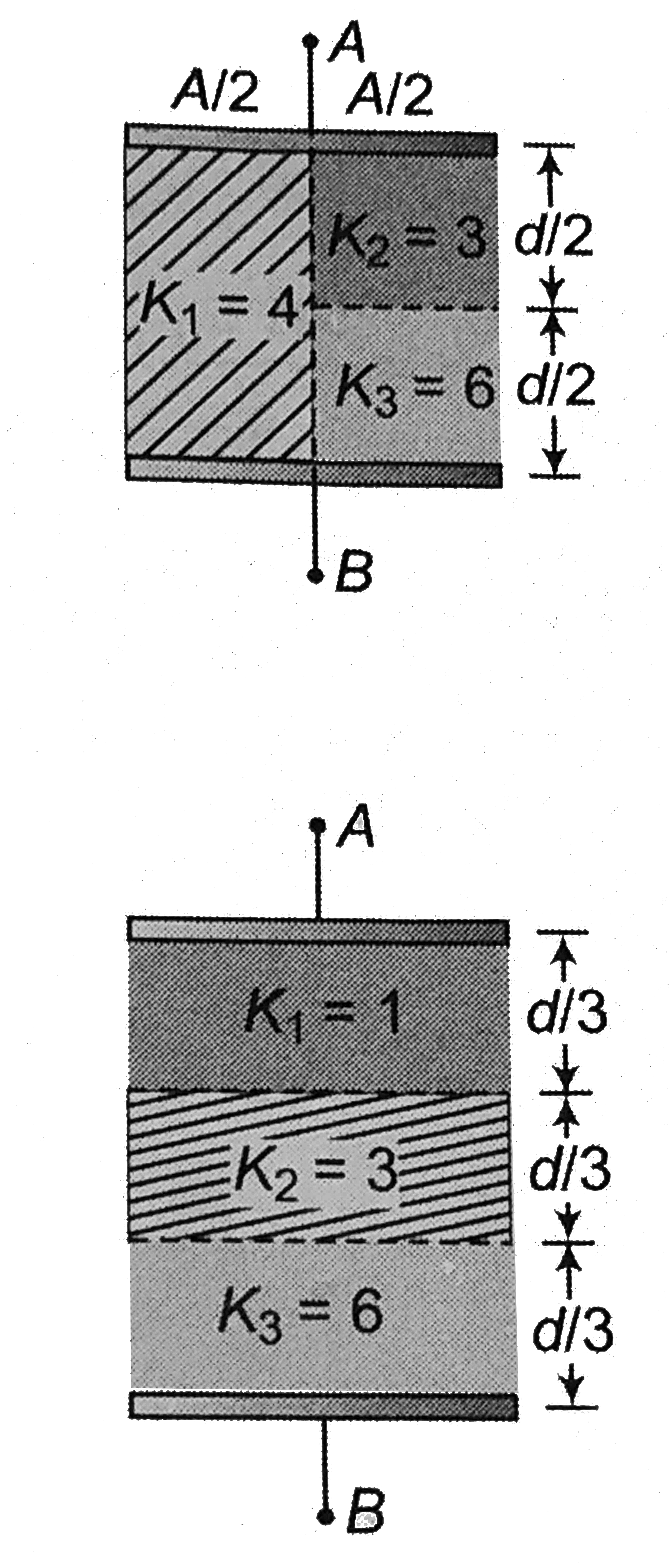
View SolutionA charge of 1μC is given to one plate of a parallel - plate capacitor of capacitance 0.1μF and a charge of 2μC is given to the other plate . Find the potential difference developed between the plate .
View SolutionA parallel plate capacitor of capacitance 3μF has total charge +15μC on one plate and total charge −15μC on the other plate. The separation between the plates is 1mm. The electric field between the plates has magnitude: (in N/C)
View SolutionFind potential difference across the plates AB if capacitance between the plates is C and area of each plate is equal and Q1,Q2(<Q1) are charges given to the plates
View Solution
