Assertion: Two capacitors are connected in series with a battery. Energy stored across them is in inverse ratio of their capacity.
Reason: U=12qV or U∝qV
If both Assertion and Reason are true and the Reason is correct explanation of the Assertion.
If both Assertion and Reason are true but Reason is not the correct explanation of Assertion.
If Assertion is true, but the Reason is false.
If Assertion is false but the Reason is true.
The correct Answer is:B
To solve the question, we need to analyze both the assertion and the reason provided.
Step 1: Understand the Assertion
The assertion states that "Two capacitors are connected in series with a battery. Energy stored across them is in inverse ratio of their capacity."
When capacitors are connected in series, they share the same charge (Q). The energy stored in a capacitor is given by the formula:
U=12QV
where V is the voltage across the capacitor.
Step 2: Energy Stored in Each Capacitor
For two capacitors C1 and C2 connected in series, the total voltage V is divided between the two capacitors. The voltage across each capacitor can be expressed as:
V1=QC1
V2=QC2
The energy stored in each capacitor can be calculated as follows:
- For capacitor C1:
U1=12QV1=12Q(QC1)=Q22C1
- For capacitor C2:
U2=12QV2=12Q(QC2)=Q22C2
Step 3: Ratio of Energies
Now, we can find the ratio of the energies stored in the two capacitors:
U1U2=Q22C1Q22C2=C2C1
This shows that the energy stored in the capacitors is indeed in the inverse ratio of their capacitances, confirming the assertion.
Step 4: Analyze the Reason
The reason states: "U = 1/2 qV or U is proportional to qV."
This is a true statement as the energy stored in a capacitor is given by U=12QV. However, while this statement is true, it does not directly explain why the energy stored in the capacitors is in inverse ratio to their capacitance when connected in series.
Conclusion
Both the assertion and the reason are true, but the reason does not correctly explain the assertion. Therefore, the correct answer to the question is:
B: Both assertion and reason are true, but the reason is not a correct explanation for the assertion.
---
The assertion states that "Two capacitors are connected in series with a battery. Energy stored across them is in inverse ratio of their capacity."
U=12QV
where V is the voltage across the capacitor.
For two capacitors C1 and C2 connected in series, the total voltage V is divided between the two capacitors. The voltage across each capacitor can be expressed as:
V1=QC1
V2=QC2
- For capacitor C1:
U1=12QV1=12Q(QC1)=Q22C1
U2=12QV2=12Q(QC2)=Q22C2
Now, we can find the ratio of the energies stored in the two capacitors:
U1U2=Q22C1Q22C2=C2C1
The reason states: "U = 1/2 qV or U is proportional to qV."
Both the assertion and the reason are true, but the reason does not correctly explain the assertion. Therefore, the correct answer to the question is:
B: Both assertion and reason are true, but the reason is not a correct explanation for the assertion.
Topper's Solved these Questions
- CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - CAPACITORSEXERCISE - OBJECTIVE_TYPE
1 Videos
- CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - CAPACITORSEXERCISE - OBJECTIVE_TYPE
1 Videos
- ATOMSBOOK - DC PANDEY ENGLISHCHAPTER - ATOMSEXERCISE - MEDICAL ENTRANCES GALLERY
42 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Subjective
11 Videos
Similar Questions
Two capacitors of 10μF and 20μF are connected in series with a 30V battery. The charge on the capacitors will be, respectively
View SolutionTwo identical capacitors are first connected in series and then in parallel. The ratio of equivalent capacitance is
View SolutionTwo capacitors A and B are connected in series with a battery as shown in the figure. When the switch S is closed and the two capacitors get charged fully, then
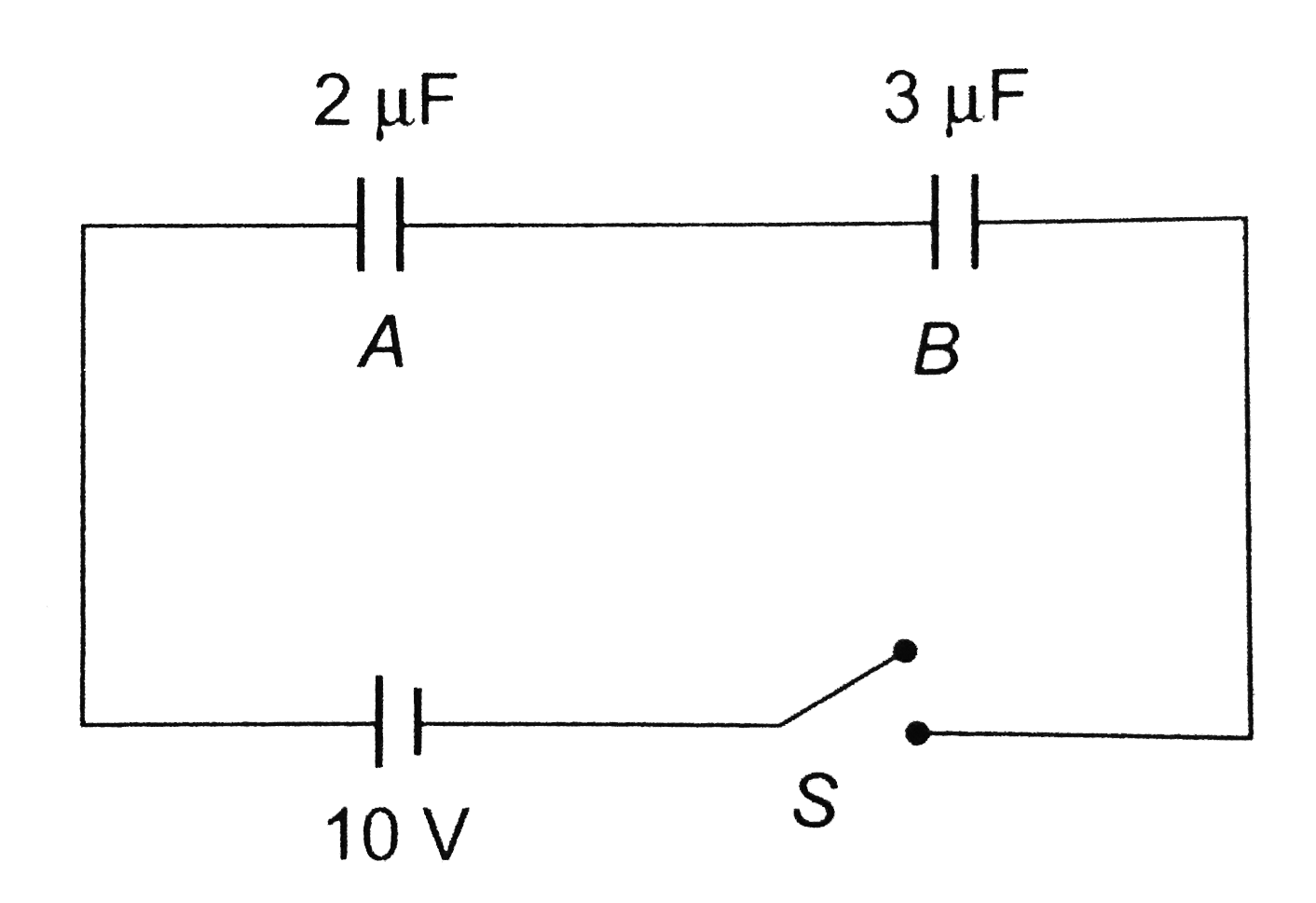
View SolutionTwo capacitors A and B are connected in series with a battery as shown in the figure. When the switch S is closed and the two capacitors get charged fully, then

View SolutionTwo capacitors A and B are connected in series with a battery as shown in the figure. When the switch S is closed and the two capacitors get charged fully, then

View SolutionAn uncharged capacitor is connected in series with a resistor and a battery. The charging of the capacitor starts at t=0. The rate at which energy stored in the capacitor:-
View SolutionA capacitor of capacitance C1 is charged to a potential V1 while another capacitor of capacitance C2 is charged to a potential difference V2 . The capacitors are now disconnected from their respective charging batteries and connected in parallel to each other .
(a) Find the total energy stored in the two capacitors before they are connected.
(b) Find the total energy stored in the parallel combination of the two capacitors.
(c ) Explain the reason for the difference of energy in parallel combination in comparison to the total energy before they are connected.
View SolutionTwo capacitors of capacitance of 6μFand12μF are connected in series with a battery. The voltage across the 6μF capacitor is 2V. Compute the total battery voltage.
View SolutionTwo identical capacitor C1 and C2 are connected in series with a battery. They are fully charged. Now a dielectric slab is inserted between the plates of C2. The potential across C1 will :
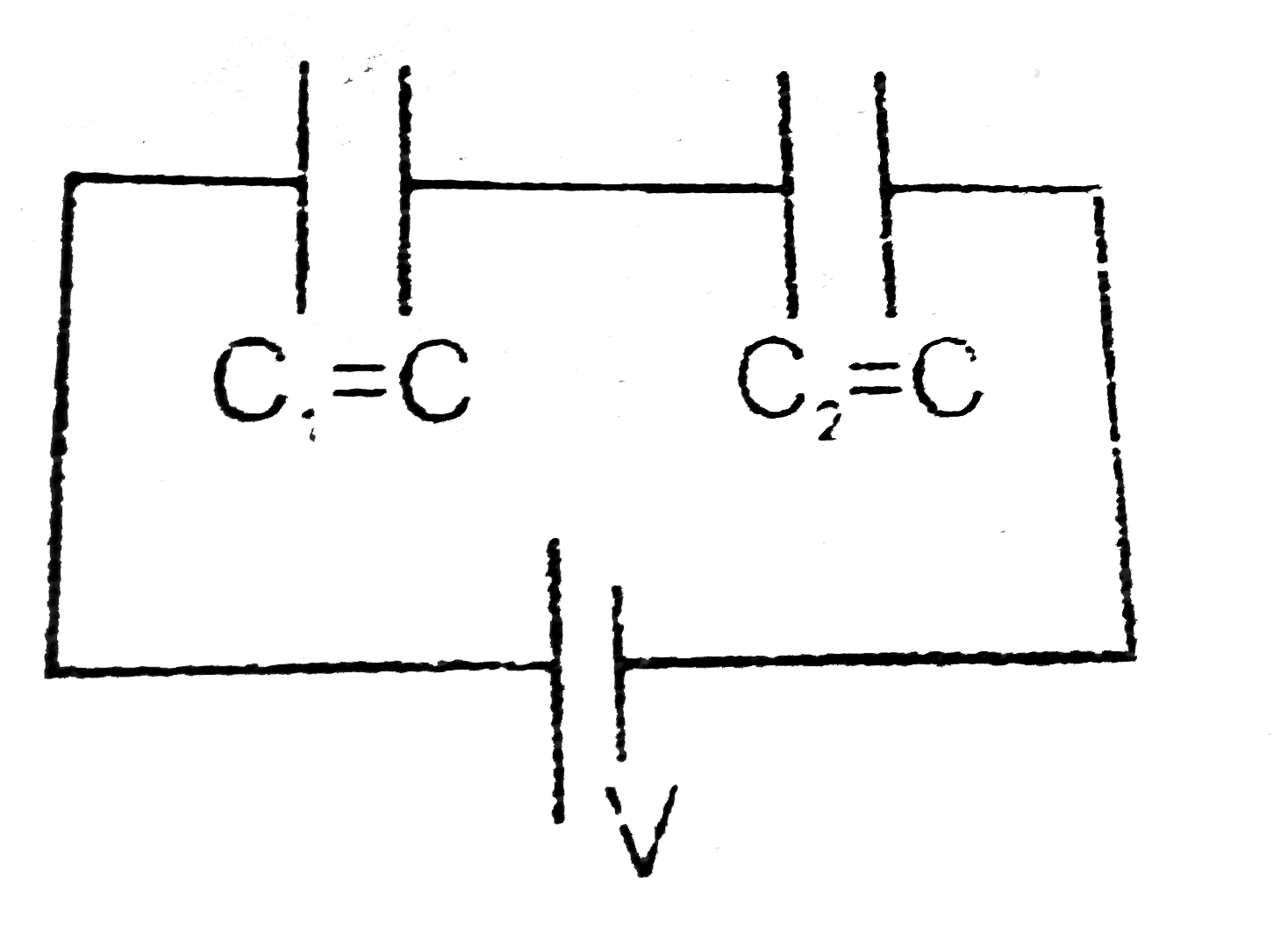
View SolutionTwo identical parallel plate capacitors are connected in series to a battery of 100V. A dielectric slab of dielectric constant 4.0 is inserted between the plates of second capacitor. The potential difference across the capacitors will now be respectively
View Solution
