A wire of mass 100g is carrying a current of 2A towards increasing x in the form of y=x2(−2m≤x≤+2m). This wire is placed in a magnetic field B=−0.02ˆk tesla. The acceleration of the wire (inms2) is
−1.6ˆj
−3.2ˆj
1.6ˆj
zero
The correct Answer is:C
To solve the problem, we need to find the acceleration of the wire carrying a current in a magnetic field. Here are the steps to arrive at the solution:
Step 1: Identify the Given Values
- Mass of the wire, m=100g=0.1kg
- Current in the wire, I=2A
- Magnetic field, B=−0.02^kT
Step 2: Determine the Effective Length of the Wire
The wire is described by the equation y=x2 from x=−2 to x=2. The effective length of the wire can be calculated as follows:
- The length of the wire can be calculated using the limits of integration:
L=∫2−2√1+(dydx)2dx
The derivative dydx=2x. Therefore,
L=∫2−2√1+(2x)2dx=∫2−2√1+4x2dx
However, for the sake of this problem, we can consider the effective length of the wire to be L=4m (the horizontal span from -2 to 2).
Step 3: Calculate the Force on the Wire
The force F on a current-carrying wire in a magnetic field is given by the formula:
F=IL×B
Where:
- L=4^im (since the wire is along the x-direction)
- B=−0.02^kT
Now, we calculate the cross product:
F=2⋅(4^i)×(−0.02^k)
Using the right-hand rule, we find:
^i×^k=^j⇒F=8⋅(−0.02)^j=−0.16^jN
Step 4: Calculate the Acceleration
Using Newton's second law, F=ma:
a=Fm
Substituting the values we have:
a=−0.16^j0.1=−1.6^jm/s2
Final Answer
The acceleration of the wire is:
a=−1.6^jm/s2
---
- Mass of the wire, m=100g=0.1kg
- Current in the wire, I=2A
- Magnetic field, B=−0.02^kT
The wire is described by the equation y=x2 from x=−2 to x=2. The effective length of the wire can be calculated as follows:
- The length of the wire can be calculated using the limits of integration:
L=∫2−2√1+(dydx)2dx
The force F on a current-carrying wire in a magnetic field is given by the formula:
- L=4^im (since the wire is along the x-direction)
- B=−0.02^kT
Using Newton's second law, F=ma:
The acceleration of the wire is:
Topper's Solved these Questions
- MAGNETICSBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETICSEXERCISE - MCQ_TYPE
1 Video
- MAGNETICSBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETICSEXERCISE - Exercise
160 Videos
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Medical entrance s gallery
59 Videos
- MAGNETISM AND MATTERBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETISM AND MATTEREXERCISE - Medical gallery
1 Video
Similar Questions
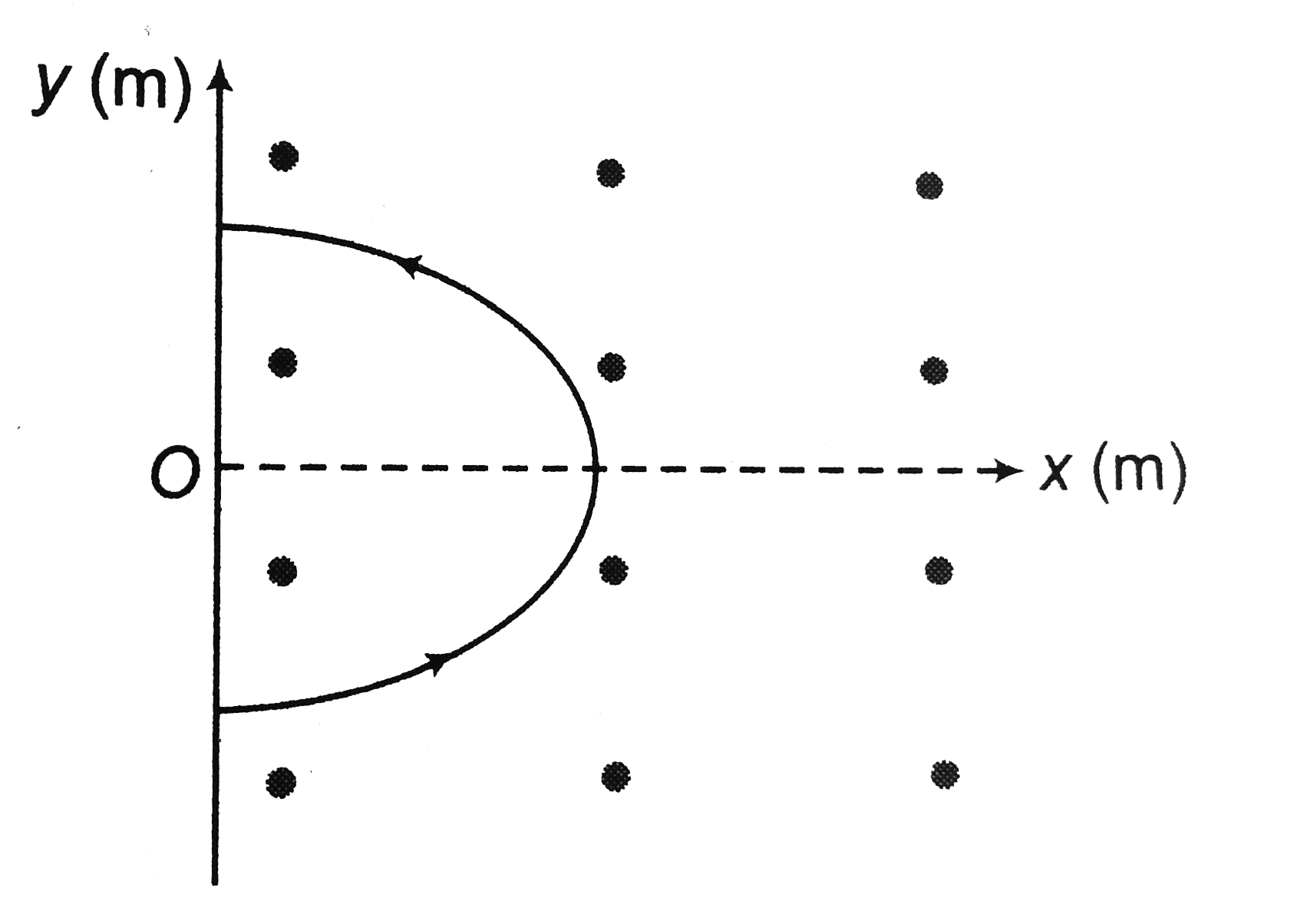
A wire carrying a current of 3A is bent in the form of a parabola y2=4−x as shown in figure, where x and y are in metre. The wire is placed in a uniform magnetic field B=5ˆk tesla. The force acting on the wire is
View SolutionA unifrom conducting wire ABC has a mass of 10g. A current of 2A flows through it. The wire is kept in a unifrom magnetic field B=2T. The accleration of the wire will be

View Solution
Knowledge Check
A current of 10 ampere is flowing in a wire of length 1.5m. A force of 15N acts on it when it is placed in a uniform magnetic field of 2 tesla. The angle between the magnetic field and the direction of the current is
A30∘B45∘C60∘D90∘
A conducting wire bent in the from of a parabola y2=2x carries a current i=2A as shown in figure This wire is placed in a unifrom magnetic field
→B=−4ˆk Tesla The magnetic force on the wire is (newton)
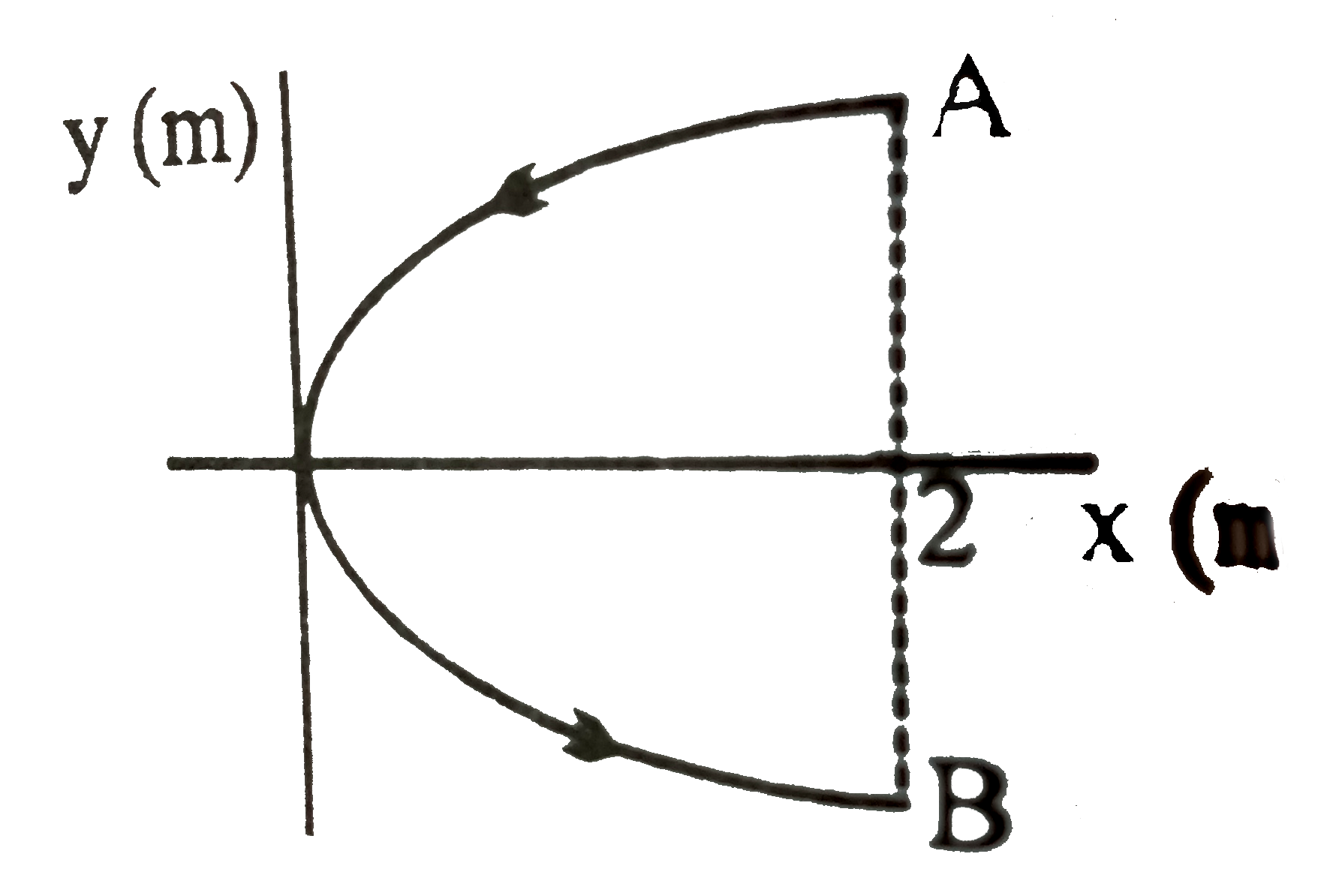 .
.
View SolutionA wire of length 1rn along x-axis carries a current 2A , is placed inside the field of →B=(3.0ˆj+4.0ˆk) tesla, then force exerted on the wire is
View SolutionA wire carrying a current i is placed in a uniform magnetic field in the form of the curve y=asin(πxL)0≤x≤2L. The force acting on the wire is
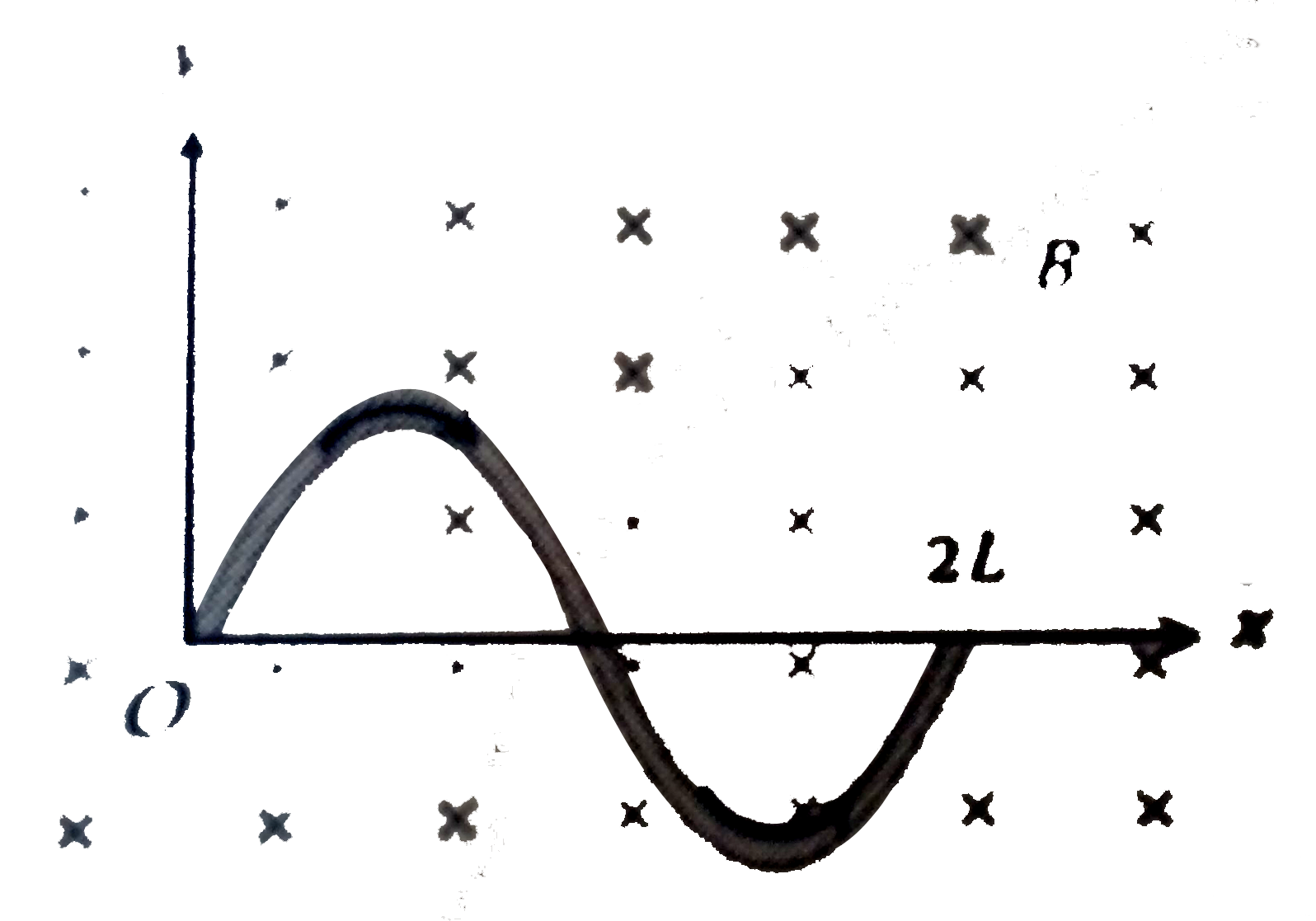
View SolutionA straight wire of mass 400 g and length 2m carries a current of 2A. It is suspended in mid air by a uniform horizontal field B. What is the magnitude of the magnetic field ?
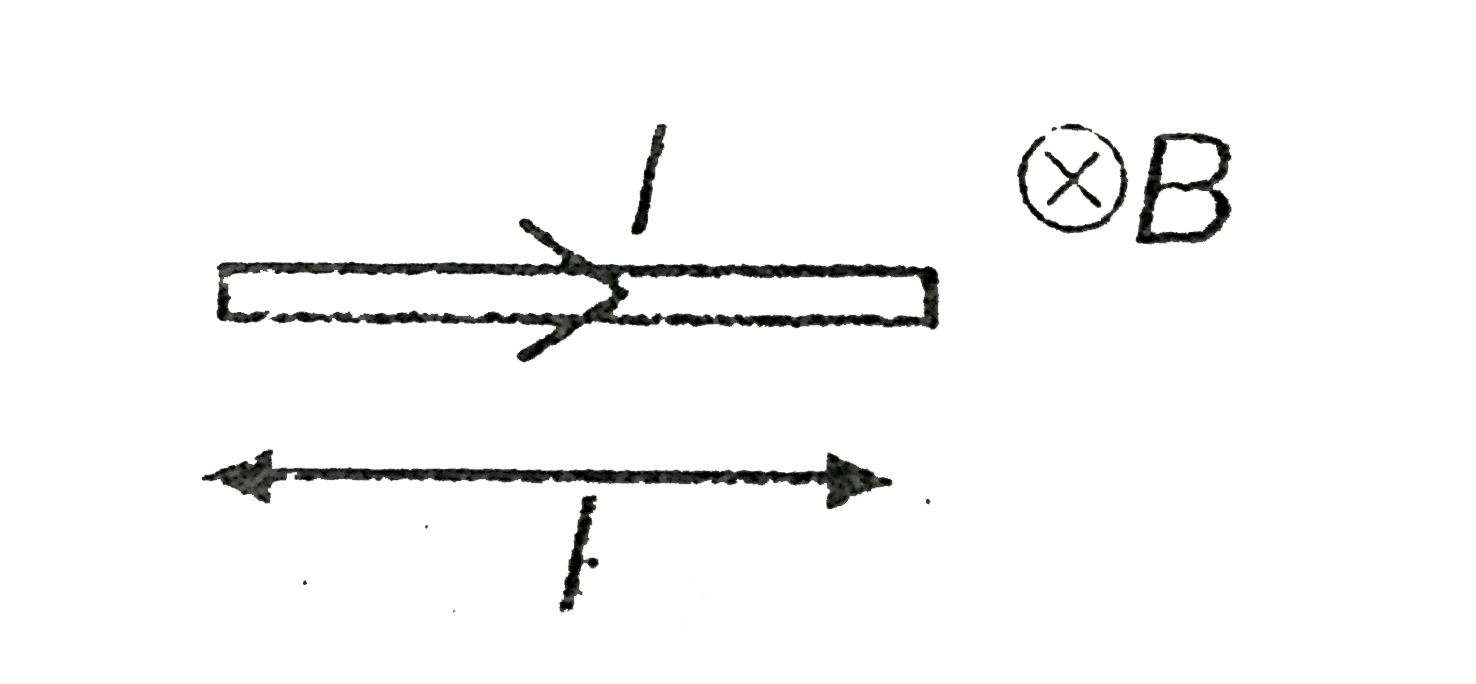
View SolutionA straight wire of mass 400 g and length 2m carries a current of 2A. It is suspended in mid air by a uniform horizontal field B. What is the magnitude of the magnetic field ?

View SolutionA current of 10 ampere is flowing in a wire of length 1.5m. A force of 15N acts on it when it is placed in a uniform magnetic field of 2 tesla. The angle between the magnetic field and the direction of the current is
View SolutionIn the figure,the force on the wire ABC in the given uniform magnetic field will be (B=2tesla)
View Solution