An L−C circuit consists of a 20.0mH inductor and a 0.5μF capacitor. If the maximum instantaneous current is 0.1A, what is the greatest potential difference across the capacitor?
To solve the problem, we need to find the greatest potential difference across the capacitor in an L-C circuit consisting of a 20.0 mH inductor and a 0.5 µF capacitor, given that the maximum instantaneous current is 0.1 A.
1. Identify the Formula for Maximum Voltage:
The maximum voltage across the capacitor (V_max) in an L-C circuit can be derived from the relationship between the maximum current (I_max), inductance (L), and capacitance (C). The formula is:
Vmax=Imax√LC
2. Convert Units:
We need to ensure that the units are consistent. The inductance is given as 20.0 mH (milliHenries) and the capacitance is given as 0.5 µF (microFarads). We convert these to standard SI units:
- L=20.0mH=20.0×10−3H=0.020H
- C=0.5μF=0.5×10−6F=0.0000005F=5×10−7F
3. Substitute Values into the Formula:
Now we can substitute the values into the formula:
Vmax=Imax√LC=0.1A√0.020H5×10−7F
4. Calculate the Square Root:
First, calculate the fraction inside the square root:
0.0205×10−7=40000
Now take the square root:
√40000=200
5. Calculate V_max:
Now substitute back to find V_max:
Vmax=0.1×200=20V
Final Answer:
The greatest potential difference across the capacitor is 20 V.
The maximum voltage across the capacitor (V_max) in an L-C circuit can be derived from the relationship between the maximum current (I_max), inductance (L), and capacitance (C). The formula is:
Vmax=Imax√LC
We need to ensure that the units are consistent. The inductance is given as 20.0 mH (milliHenries) and the capacitance is given as 0.5 µF (microFarads). We convert these to standard SI units:
- L=20.0mH=20.0×10−3H=0.020H
- C=0.5μF=0.5×10−6F=0.0000005F=5×10−7F
Now we can substitute the values into the formula:
Vmax=Imax√LC=0.1A√0.020H5×10−7F
First, calculate the fraction inside the square root:
0.0205×10−7=40000
Now take the square root:
√40000=200
Now substitute back to find V_max:
Vmax=0.1×200=20V
The greatest potential difference across the capacitor is 20 V.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercise 27.72videos
ELECTROMAGNETIC INDUCTION
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Level 1 Assertion And Reason10videos
ELECTROMAGNETIC INDUCTION
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercise 27.53videos
ELECTROMAGNETIC INDUCTION
- BOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Medical entrances gallery97videos
CURRENT ELECTRICITY
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C22videos
ELECTROMAGNETIC WAVES
Similar Questions
Knowledge Check
- Question 1 - Select One
An LC circuit contains a 20 mH inductor and 25μF capacitor with an initial charge of 5 mC. The total energy stored in the circuit initially is
A5 JB0.5JC50 JD500 J
In the given circuit, with steady current, the potential difference across the capacitor is
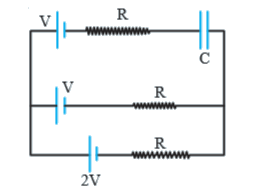 View Solution
View SolutionWhat is the potential difference across 2μF capacitor in the circuit shown?
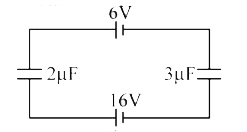 View Solution
View SolutionAn L-C circuit contains a 0.60 H inductor and a 25μF capacitor. What is rate of change of current when the charges on the capacitor is3.0×10−5C?
View SolutionThe ratio of potential differences between 1μFand5μF capacitors is
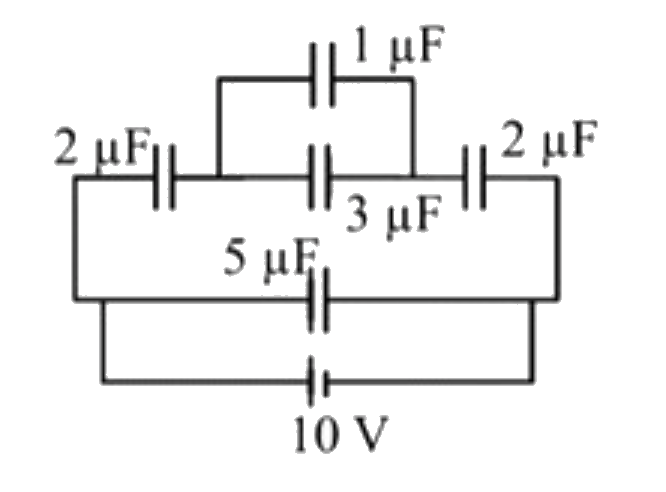 View Solution
View SolutionIn the given circuit, with steady current, the potential drop across the capacitor must be
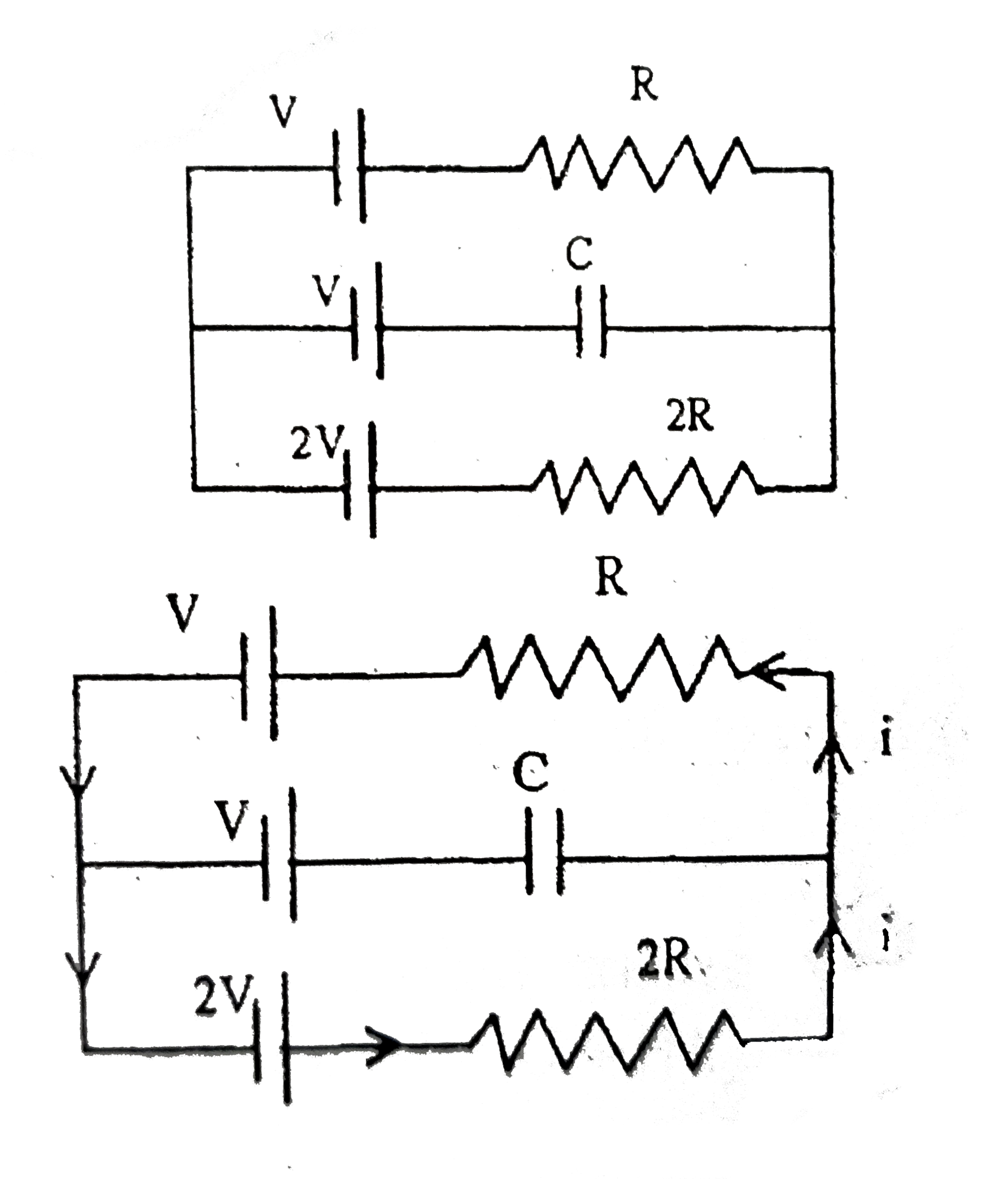 View Solution
View SolutionIn the given circuit, with steady current, the potential drop across the capacitor must be
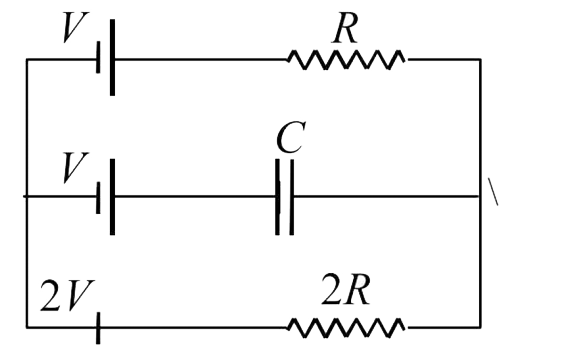 View Solution
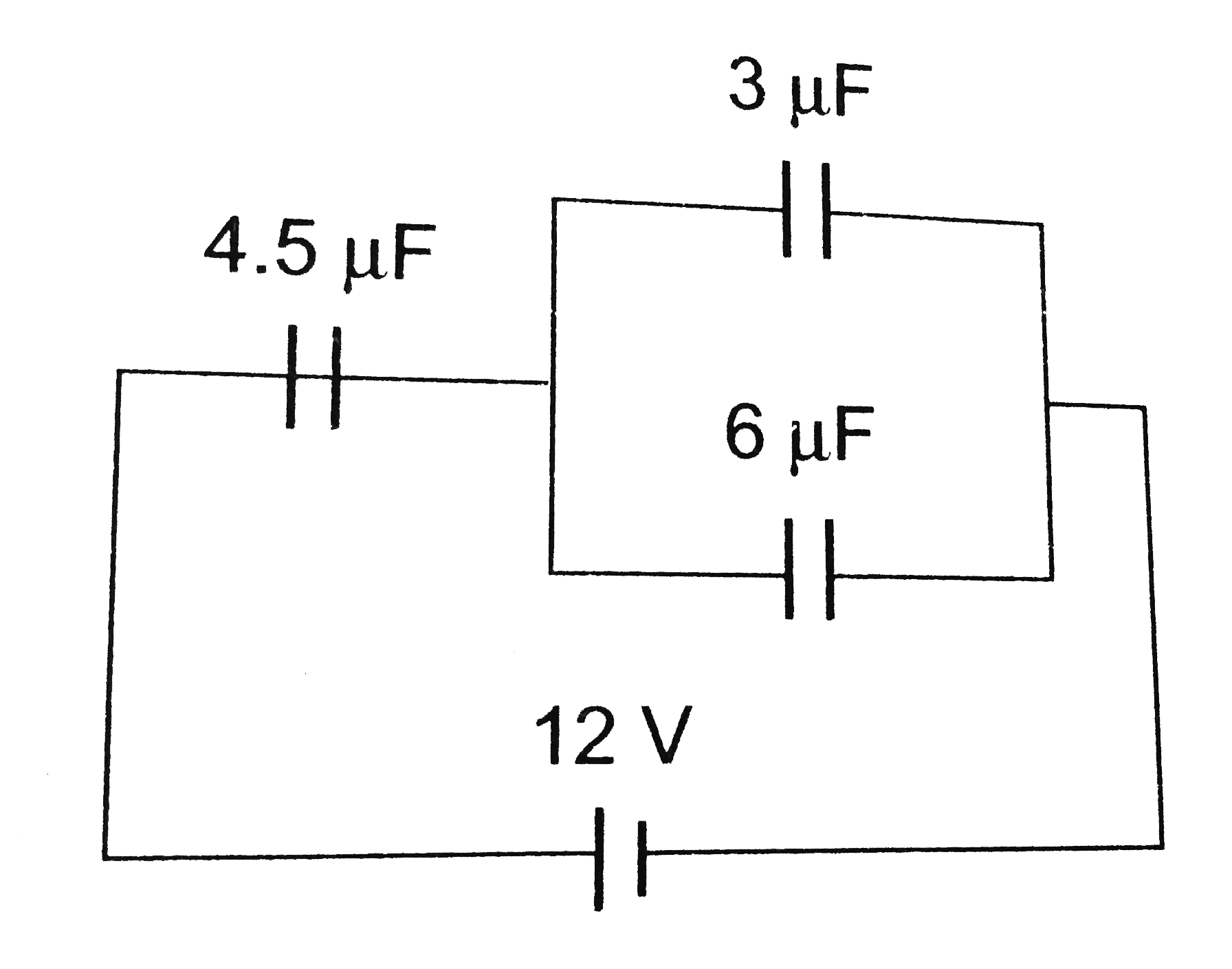
View SolutionIn the given circuit, the potential difference across the 6μF capacitor in steady state is
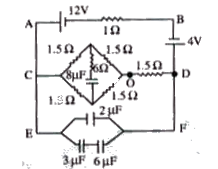 View Solution
View Solution