While comparing the L−C oscillations with the oscillations of spring-block system, with whom the magnetic energy can be compared and why?
To compare the magnetic energy in LC oscillations with another form of energy, we can analyze the oscillations of both LC circuits and spring-block systems step by step.
1. Understanding LC Oscillations:
- In an LC circuit, energy oscillates between the capacitor (electric energy) and the inductor (magnetic energy). The total energy in the system is conserved and is the sum of the energy stored in the capacitor and the energy stored in the inductor.
2. Energy Expressions:
- The energy stored in the capacitor is given by:
UC=12CV2
- The energy stored in the inductor is given by:
UL=12LI2
- Here, C is the capacitance, V is the voltage across the capacitor, L is the inductance, and I is the current through the inductor.
3. Comparing with Spring-Block System:
- In a spring-block system, the potential energy stored in the spring is given by:
Us=12kx2
- The kinetic energy of the block is given by:
K=12mv2
- Here, k is the spring constant, x is the displacement from the equilibrium position, m is the mass of the block, and v is its velocity.
4. Identifying Correspondences:
- In the LC circuit, the magnetic energy UL corresponds to the kinetic energy K in the spring-block system. This is because both represent energy associated with motion:
- The magnetic energy in the inductor is related to the current (which is analogous to velocity in the spring-block system).
- The kinetic energy in the spring-block system is directly related to the motion of the block.
5. Conclusion:
- Therefore, the magnetic energy in an LC circuit can be compared to the kinetic energy in a spring-block system because both represent energy associated with motion, albeit in different forms (magnetic field energy vs. kinetic energy of a moving mass).
- In an LC circuit, energy oscillates between the capacitor (electric energy) and the inductor (magnetic energy). The total energy in the system is conserved and is the sum of the energy stored in the capacitor and the energy stored in the inductor.
- The energy stored in the capacitor is given by:
UC=12CV2
- The energy stored in the inductor is given by:
UL=12LI2
- Here, C is the capacitance, V is the voltage across the capacitor, L is the inductance, and I is the current through the inductor.
- In a spring-block system, the potential energy stored in the spring is given by:
Us=12kx2
- The kinetic energy of the block is given by:
K=12mv2
- Here, k is the spring constant, x is the displacement from the equilibrium position, m is the mass of the block, and v is its velocity.
- In the LC circuit, the magnetic energy UL corresponds to the kinetic energy K in the spring-block system. This is because both represent energy associated with motion:
- The magnetic energy in the inductor is related to the current (which is analogous to velocity in the spring-block system).
- The kinetic energy in the spring-block system is directly related to the motion of the block.
- Therefore, the magnetic energy in an LC circuit can be compared to the kinetic energy in a spring-block system because both represent energy associated with motion, albeit in different forms (magnetic field energy vs. kinetic energy of a moving mass).
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercise 27.72videos
ELECTROMAGNETIC INDUCTION
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Level 1 Assertion And Reason10videos
ELECTROMAGNETIC INDUCTION
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercise 27.53videos
ELECTROMAGNETIC INDUCTION
- BOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Medical entrances gallery97videos
CURRENT ELECTRICITY
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C22videos
ELECTROMAGNETIC WAVES
Similar Questions
Comparing the L-C oscillations with the oscillations of a spring-block system
View SolutionIn L − C oscillations
View SolutionComparing the L-C oscillations with the oscillations of a spring-block system (force constant of spring=k and mass of block=m), the physical quantity mk is similar to
View SolutionComparing L-C oscillation with the oscillation if spring-block-system, match the following table.
Comparing L-C oscillations with the oscillations of spring-block sytem, match the following table Table-1(LC oscillations)Table-2(Spring-block oscillations)(A)L(P)k(B)C(Q)m(C)i(R)v(D)didt(S)x(T)NoneView SolutionWhy do animals consume more energy as compared to plants
View SolutionAssertion : All small oscillation are simple harmonic in nature.
Reason : Oscillation of spring block system are always simple harmonic whether amplitude is small or large.View SolutionA loaded vertical spring executes simple harmonic oscillations with period of 4 s. The difference between the kinetic energy and potential energy of this system oscillates with a period of
View SolutionIn an L-C oscillation maximum charge on capacitor can be Q0 . If at any instant electrical energy is th 34 of the magnetic energy then the charge on capacitor at that instant is
View SolutionA block of mass m is attached to a cart of mass 4m through spring of spring constant k as shown in the figure. Friction is absent everywhere. The time period of oscillations of the system, when spring is compressed and then released, is
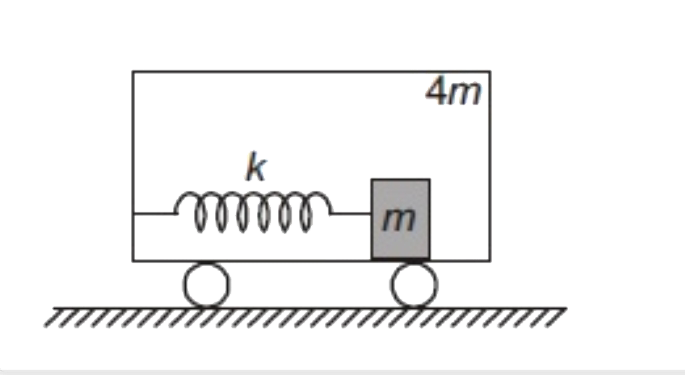 View Solution
View SolutionA block of mass M suspended from a spring oscillates with time period T. If spring is cut in to two equal parts and same mass M is suspended from one part, new period os oscillation is
View Solution

