A radioactive material of half-life T was kept in a nuclear reactor at two different instants. The quantity kept second time was twice of the kept first time. If now their present activities are A1 and A2 respectively, then their age difference equals
(a) T1n21n2A1A2
(b) T1nA1A2
(c) T1n21nA22A1
(d) T1nA22A1
The correct Answer is:C
To solve the problem step by step, we need to analyze the relationship between the activities of two samples of radioactive material kept at different times and their respective ages.
Step 1: Understand the relationship between activity and time
The activity A of a radioactive substance is related to its initial activity A0 and the decay constant λ by the equation:
A=A0e−λt
where t is the time elapsed since the material was kept.
Step 2: Set up the equations for both samples
Let’s denote:
- For the first sample, the initial activity is A01 and the time elapsed is t1.
- For the second sample, the initial activity is A02=2A01 (since the second quantity is twice the first) and the time elapsed is t2.
From the activity equation, we can write:
A1=A01e−λt1
A2=A02e−λt2=2A01e−λt2
Step 3: Relate the activities
Now we can express the ratio of the activities:
A1A2=A01e−λt12A01e−λt2=e−λt12e−λt2
This simplifies to:
A1A2=12eλ(t2−t1)
Step 4: Take the natural logarithm
Taking the natural logarithm of both sides gives:
ln(A1A2)=ln(12)+λ(t2−t1)
Step 5: Solve for the time difference
Rearranging the equation to isolate t2−t1:
λ(t2−t1)=ln(A1A2)−ln(12)
Using properties of logarithms, we can express this as:
t2−t1=1λ(ln(A1A2)+ln(2))
This can be simplified to:
t2−t1=1λln(2A1A2)
Step 6: Substitute decay constant
The decay constant λ can be expressed in terms of the half-life T:
λ=ln(2)T
Substituting this into the equation gives:
t2−t1=Tln(2)ln(2A1A2)
Final Result
Thus, the age difference between the two samples is:
t2−t1=Tln(2)ln(2A1A2)
The activity A of a radioactive substance is related to its initial activity A0 and the decay constant λ by the equation:
A=A0e−λt
where t is the time elapsed since the material was kept.
Let’s denote:
- For the first sample, the initial activity is A01 and the time elapsed is t1.
- For the second sample, the initial activity is A02=2A01 (since the second quantity is twice the first) and the time elapsed is t2.
A1=A01e−λt1
A2=A02e−λt2=2A01e−λt2
Now we can express the ratio of the activities:
A1A2=A01e−λt12A01e−λt2=e−λt12e−λt2
This simplifies to:
A1A2=12eλ(t2−t1)
Taking the natural logarithm of both sides gives:
ln(A1A2)=ln(12)+λ(t2−t1)
Rearranging the equation to isolate t2−t1:
λ(t2−t1)=ln(A1A2)−ln(12)
Using properties of logarithms, we can express this as:
t2−t1=1λ(ln(A1A2)+ln(2))
This can be simplified to:
t2−t1=1λln(2A1A2)
The decay constant λ can be expressed in terms of the half-life T:
λ=ln(2)T
Substituting this into the equation gives:
t2−t1=Tln(2)ln(2A1A2)
Thus, the age difference between the two samples is:
t2−t1=Tln(2)ln(2A1A2)
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - MODERN PHYSICS - 2EXERCISE - Level 2 More Than One Correct6videos
MODERN PHYSICS - 2
- BOOK - DC PANDEY ENGLISHCHAPTER - MODERN PHYSICS - 2EXERCISE - Level 2 Comprehension Based3videos
MODERN PHYSICS - 2
- BOOK - DC PANDEY ENGLISHCHAPTER - MODERN PHYSICS - 2EXERCISE - Level 1 Subjective Questions1videos
MODERN PHYSICS - 2
- BOOK - DC PANDEY ENGLISHCHAPTER - MODERN PHYSICS - 1EXERCISE - Level 2 Subjective23videos
MODERN PHYSICS - 1
- BOOK - DC PANDEY ENGLISHCHAPTER - NUCLEIEXERCISE - C MADICAL ENTRANCES GALLERY46videos
NUCLEI
Similar Questions
Two radioactive samples of different elements (half-lives t1 and t2 respectively) have same number of nuclei at t=0. The time after which their activities are same is
View SolutionThe count rate of 10g of radioactive material was measured at different times and times has been shown in the figure. The half-life of material and the total counts (approximately) in the first half life period, respectively are.
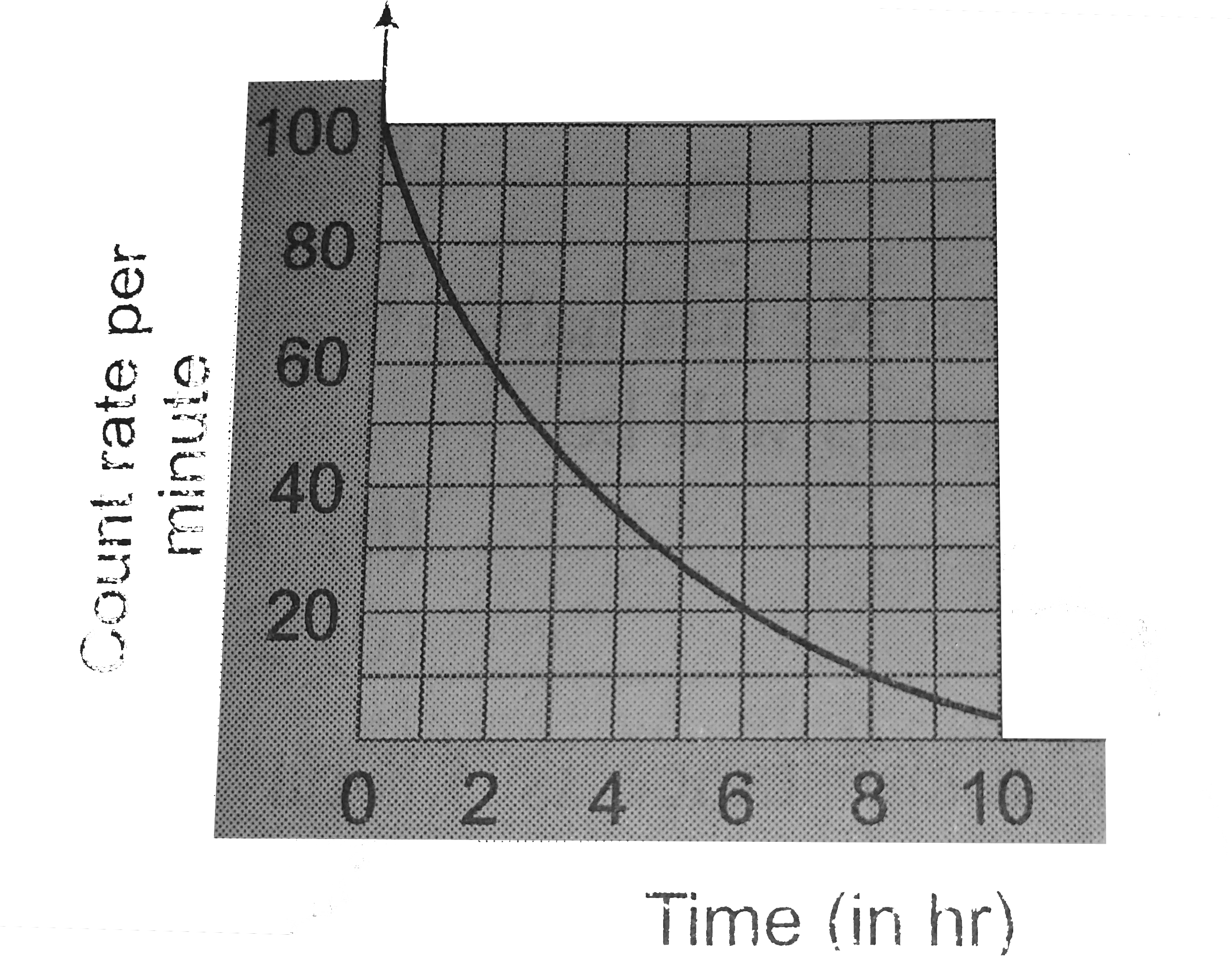.View Solution
Knowledge Check
- Question 1 - Select One
The decay constant of a radioactive isotope is λ. If A1 and A2 are its activites at time t1 and t2 respectively, then the number of nuclei which have decayed the time (t1−t2)
AA1t1−A2t2BA1−A2C(A1−A2)/λDλ(A1−A2)
A and B participate in a race with acceleration a1anda2 , respectively . A reaches t times earlier than B at finish line and their velocities at finish line are v1andv2 , respectively. If difference between their velocities is v , then find the value of v
View SolutionTwo conducting cylinders of equal length but different radii are connected in series between two heat baths kept at temperatures T1=300KandT2=100K, as shown in the figure. The radius of the bigger cylinder is twice that of the smaller one and the thermal conductivities of the materials of the smaller and the larger cylinders are K1andK2 respectively. If the temperature at the junction of the two cylinders in the steady state is 200 K, then K1/K2=__________.
View SolutionTwo identical samples (same material and same amout) PandQ of a radioactive substance having mean life T are observed to have activities AP and AQ respectively at the time of observation. If P is older than Q, then the difference in their age is
View SolutionTwo identical samples (same material and same amout) P and Q of a radioactive substance having mean life T are observed to have activities AP and AQ respectively at the time of observation. If P is older than Q, then the difference in their age is
View SolutionTwo radioactive substances have half-lives T and 2T. Initially, they have equal number of nuclei. After time t=4T, the ratio of their number of nuclei is x and the ratio of their activity is y. Then,
View SolutionFor a radioactive material, its activity A and rate of change of its activity of R are defined as A=−dNdt and R=−dAdt, where N(t) is the number of nuclei at time t. Two radioactive source P (mean life τ) and Q (mean life 2τ) have the same activity at t=0. Their rates of activities at t=2τ are Rp and RQ, respectively. If RPRQ=ne, then the value of n is:
View SolutionThe half-life of a radioactive sample is T. If the activities of the sample at time t1 and t2 (t1<t2) and R1 and R2 respectively, then the number of atoms disintergrated in time t2−t1 is proportional to
View Solution