A cannon ball is fired with a velocity 200m / sec at an angle of 60° with the horizontal. At the highest point of its flight it explodes into 3 equal fragments, one going vertically upwards with a velocity 100 m / sec , the second one falling vertically downwards with a velocity 100 m / sec . The third fragment will be moving with a velocity
100ms−1 in the horizontal direction
300ms−1 in the horizontal direction
300ms−1 in a direction making an angle of 60∘ with the horizontal
200ms−1 in a direction making an angle of 60∘0 with the horizontal
The correct Answer is:B
To solve the problem step by step, we will analyze the motion of the cannonball and apply the principle of conservation of momentum.
Step 1: Determine the initial velocity components of the cannonball.
The cannonball is fired with a velocity of 200m/s at an angle of 60∘ with the horizontal. We can break this velocity into its horizontal and vertical components.
- Horizontal component (ux):
ux=200cos(60∘)=200×12=100m/s
- Vertical component (uy):
uy=200sin(60∘)=200×√32=100√3m/s
Step 2: Analyze the motion at the highest point.
At the highest point of its flight, the vertical component of the velocity becomes 0 (as it momentarily stops rising before falling). Therefore, the velocity of the cannonball at the highest point is purely horizontal:
→V=100^im/s
Step 3: Apply conservation of momentum during the explosion.
When the cannonball explodes into three equal fragments, we need to apply the conservation of momentum. The initial momentum of the cannonball before the explosion is:
→Pinitial=m⋅→V=m⋅(100^i)kg m/s
After the explosion, the momentum of the three fragments can be expressed as follows:
1. The first fragment goes vertically upwards with a velocity of 100m/s:
→P1=m3⋅(100^j)kg m/s
2. The second fragment falls vertically downwards with a velocity of 100m/s:
→P2=m3⋅(−100^j)kg m/s
3. The third fragment has an unknown velocity →V3:
→P3=m3⋅→V3kg m/s
Step 4: Set up the momentum conservation equation.
According to the conservation of momentum, the total initial momentum must equal the total final momentum:
m⋅(100^i)=m3⋅(100^j)+m3⋅(−100^j)+m3⋅→V3
Step 5: Simplify the equation.
The vertical components from the first two fragments cancel each other out:
m⋅(100^i)=m3⋅→V3
Step 6: Solve for →V3.
We can cancel m from both sides (assuming m≠0):
100^i=13→V3
Multiplying both sides by 3:
→V3=300^im/s
Conclusion
The third fragment moves with a velocity of 300m/s in the horizontal direction.
ux=200cos(60∘)=200×12=100m/s
uy=200sin(60∘)=200×√32=100√3m/s
→P1=m3⋅(100^j)kg m/s
→P2=m3⋅(−100^j)kg m/s
→P3=m3⋅→V3kg m/s
Topper's Solved these Questions
- CENTRE OF MASSBook:DC PANDEY ENGLISHChapter:CENTRE OF MASSExercise:Assertion and reason
Explore 21 Videos
- CENTRE OF MASSBook:DC PANDEY ENGLISHChapter:CENTRE OF MASSExercise:Match the coloumns
Explore 9 Videos
- CENTRE OF MASSBook:DC PANDEY ENGLISHChapter:CENTRE OF MASSExercise:Check points
Explore 50 Videos
- CALORIMETRY AND HEAT TRANSFERBook:DC PANDEY ENGLISHChapter:CALORIMETRY AND HEAT TRANSFERExercise:Medical entrance s gallery
Explore 38 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBook:DC PANDEY ENGLISHChapter:CENTRE OF MASS, IMPULSE AND MOMENTUMExercise:Comprehension type questions
Explore 15 Videos
Similar Questions
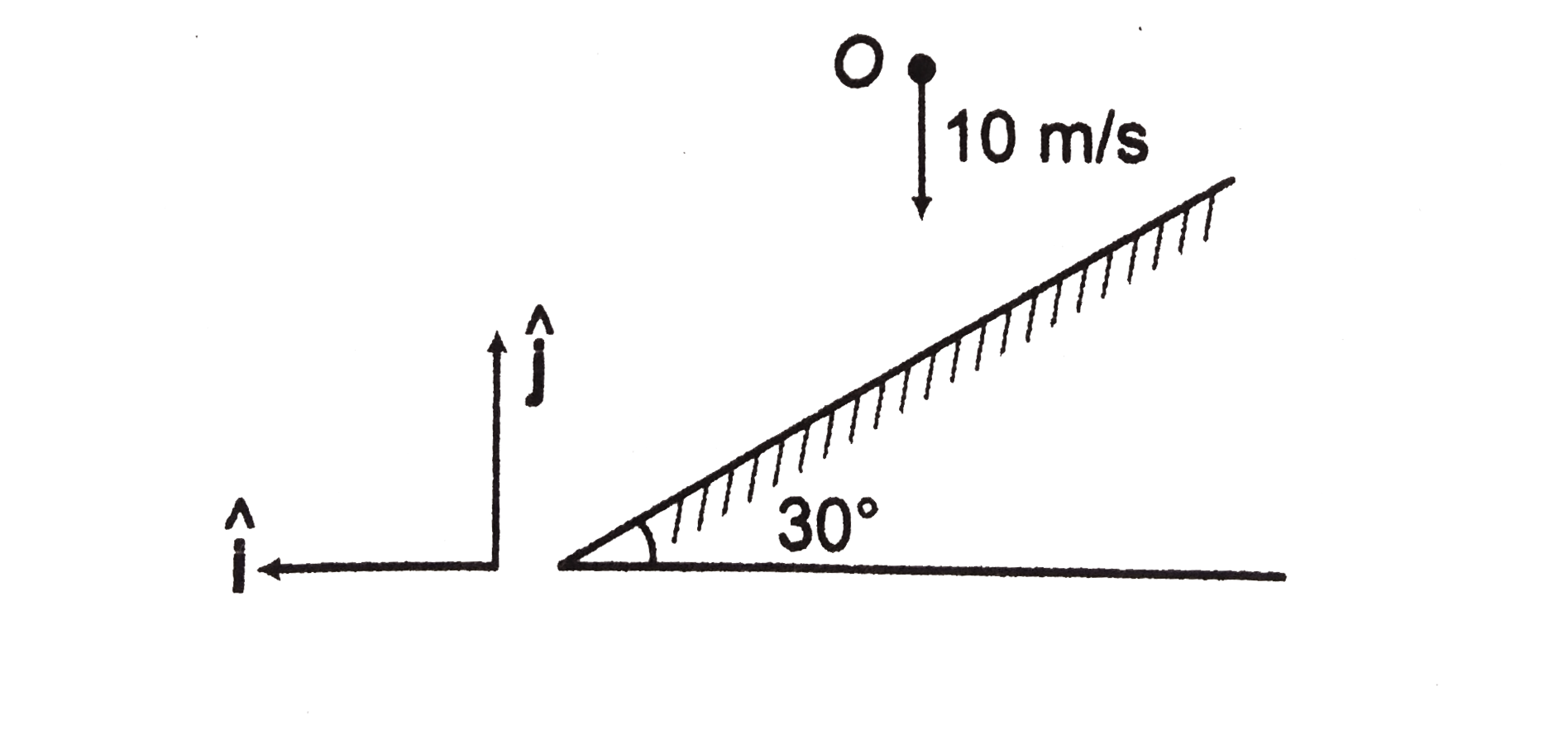
An object is falling vertically downwards with velocity 10m/s.In terms ofˆiandˆj find the image velocity. M
View SolutionA body is projected with an initial Velocity 20 m/s at 60° to the horizontal. Its velocity after 1 sec is
View SolutionA ball is projected vertically up wards with a velocity of 100 m/s. Find the speed of the ball at half the maximum height. (g=10m/s2)
View SolutionA ball thrown vertically upwards with an initial velocity of 1.4m/s. The total displcement of the ball is
View SolutionA projectile is fired with velocity v0 at angle 60∘ with horizontal. At top os its trajectory it explodes into three fragments of equal masses. First fragment retraces the path, second moves vertically upwards with speed 3v02. Speed of the third fragment is
View SolutionA projectile is fired with velocity.v0 at an angle 60∘ with horizontal . At top of its trajectory it explodes into two fragments of equal mass. If one fragment retraces the path then the speed of the other fragment is
View SolutionA body is projected with an initial velocity of 58.8 m/s at angle 60° with the vertical. The vertical component of velocity after 2 sec is
View SolutionA shell is fired from a cannon with a velocity V at an angle θ with the horizontal direction. A the highest point i its path, it explodes into two pieces of equal masses. One of the pieces retraces its path to the cannon. The speed of the other piece immediately after the explosion is
View SolutionA shell is fired from a cannon with a speed of 100m/s at an angle 30∘with the vertical (y-direction). At the highest point of its trajectory, the shell explodes into two fragments of masses in the ratio 1:2. The lighter fragment moves vertically upwards with an initial speed of 200m/s. What is the speed of the heavier fragment at the time of explosion?
View SolutionA projectile is fired with velocity u at an angle θ with horizontal. At the highest point of its trajectory, it splits up into three segments of masses m, m and 2m. First part falls vertically downward with zero initial velocity and second part returns via same path to the point of projection. The velocity of third part of mass 2 m just after explosion will be
View Solution