A particle mass m begins to slide down a fixed smooth sphere from the top of its vertical diameter. Calculate its tangential acceleration, radial acceleration and total acceleration when it breaks off.
2g3
√5g3
g
g3
The correct Answer is:B
To solve the problem of a particle of mass m sliding down a fixed smooth sphere from the top of its vertical diameter, we need to calculate its tangential acceleration, radial acceleration, and total acceleration when it breaks off. Here’s a step-by-step solution:
Step 1: Determine the position of the particle
- The particle starts at the top of the sphere and slides down to a point P at an angle θ from the vertical.
Step 2: Analyze forces acting on the particle
- At point P, the forces acting on the particle are:
- The gravitational force mg acting downward.
- The normal force N exerted by the sphere acting perpendicular to the surface.
Step 3: Resolve gravitational force into components
- The gravitational force can be resolved into two components:
- Radial component: mgcosθ (towards the center of the sphere)
- Tangential component: mgsinθ (along the direction of motion)
Step 4: Apply Newton's second law in the radial direction
- The net radial force is given by:
N−mgcosθ=−m⋅ar
where ar is the radial acceleration.
- At the point of breaking off, the normal force N=0:
−mgcosθ=−m⋅ar⟹ar=gcosθ
Step 5: Apply conservation of energy
- The total mechanical energy is conserved. Initially, the particle has potential energy and no kinetic energy:
E1=mgh=mgR(at the top)
- At point P:
E2=Potential Energy+Kinetic Energy=mg(R−Rcosθ)+12mv2
Setting E1=E2:
mgR=mgR(1−cosθ)+12mv2
Simplifying gives:
mgRcosθ=12mv2⟹v2=2gRcosθ
Step 6: Radial acceleration
- The radial acceleration can be expressed as:
ar=v2R=2gRcosθR=2gcosθ
Step 7: Tangential acceleration
- The tangential acceleration at is given by:
at=gsinθ
Step 8: Calculate values at the breaking point
- At the breaking point, we have found that cosθ=23:
- Calculate ar:
ar=2gcosθ=2g⋅23=4g3
- Calculate at:
at=gsinθ=g√1−cos2θ=g√1−(23)2=g√59=g√53
Step 9: Total acceleration
- The total acceleration a is given by:
a=√a2r+a2t
Substituting the values:
a=
⎷(4g3)2+(g√53)2=√16g29+5g29=√21g29=g√213
Final Results
- Radial Acceleration ar: 4g3
- Tangential Acceleration at: g√53
- Total Acceleration a: g√213
- The particle starts at the top of the sphere and slides down to a point P at an angle θ from the vertical.
- At point P, the forces acting on the particle are:
- The gravitational force mg acting downward.
- The normal force N exerted by the sphere acting perpendicular to the surface.
- The gravitational force can be resolved into two components:
- Radial component: mgcosθ (towards the center of the sphere)
- Tangential component: mgsinθ (along the direction of motion)
- The net radial force is given by:
N−mgcosθ=−m⋅ar
where ar is the radial acceleration.
- At the point of breaking off, the normal force N=0:
−mgcosθ=−m⋅ar⟹ar=gcosθ
- The total mechanical energy is conserved. Initially, the particle has potential energy and no kinetic energy:
E1=mgh=mgR(at the top)
- At point P:
E2=Potential Energy+Kinetic Energy=mg(R−Rcosθ)+12mv2
Setting E1=E2:
mgR=mgR(1−cosθ)+12mv2
Simplifying gives:
mgRcosθ=12mv2⟹v2=2gRcosθ
- The radial acceleration can be expressed as:
ar=v2R=2gRcosθR=2gcosθ
- The tangential acceleration at is given by:
at=gsinθ
- At the breaking point, we have found that cosθ=23:
- Calculate ar:
ar=2gcosθ=2g⋅23=4g3
- Calculate at:
at=gsinθ=g√1−cos2θ=g√1−(23)2=g√59=g√53
- The total acceleration a is given by:
a=√a2r+a2t
Substituting the values:
a=
⎷(4g3)2+(g√53)2=√16g29+5g29=√21g29=g√213
- Radial Acceleration ar: 4g3
- Tangential Acceleration at: g√53
- Total Acceleration a: g√213
Topper's Solved these Questions
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - JEE Advanced
24 Videos
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - More than One Option is Correct
13 Videos
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Subjective Questions
2 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Subjective
21 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Only One Option is Correct
27 Videos
Similar Questions
A particle of mass m starts to slide down from the top of the fixed smooth sphere. What is the tangential acceleration when it break off the sphere ?
View SolutionA particle of mass m is thrown horizontally from the top of a tower and anoher particle of mass 2m is thrown vertically upward. The acceleration of centre of mass is
View SolutionA particle of mass m is thrown horizontally from the top of a tower and anoher particle of mass 2m is thrown vertically upward. The acceleration of centre of mass is
View SolutionA point starts from rest and moves along a circular path with a constant tangential acceleration. After one rotation, the ratio of its radial acceleration to its tangential acceleration will be equal to
View SolutionA particle moves in a circle with constant tangential acceleration starting from rest. At t = 1/2 s radial acceleration has a value 25% of tangential acceleration. The value of tangential acceleration will be correctly represented by
View SolutionA particle is moving on a circle of radius A .At an instant its speed and tangential acceleration are B and C respectively.Total acceleration of the particle at that instant is
View SolutionA particle is moving along a circle and its linear velocity is 0.5ms when its angular velocity is 2.5rads−1. Its tangential acceleration is 0.2ms−2 then its angular acceleration is
View SolutionA particle is given an intial speed u inside a smooth spherical shell of radius R so that it is just able to complete the circle. Acceleration of the particle, when its velocity is vertical, is

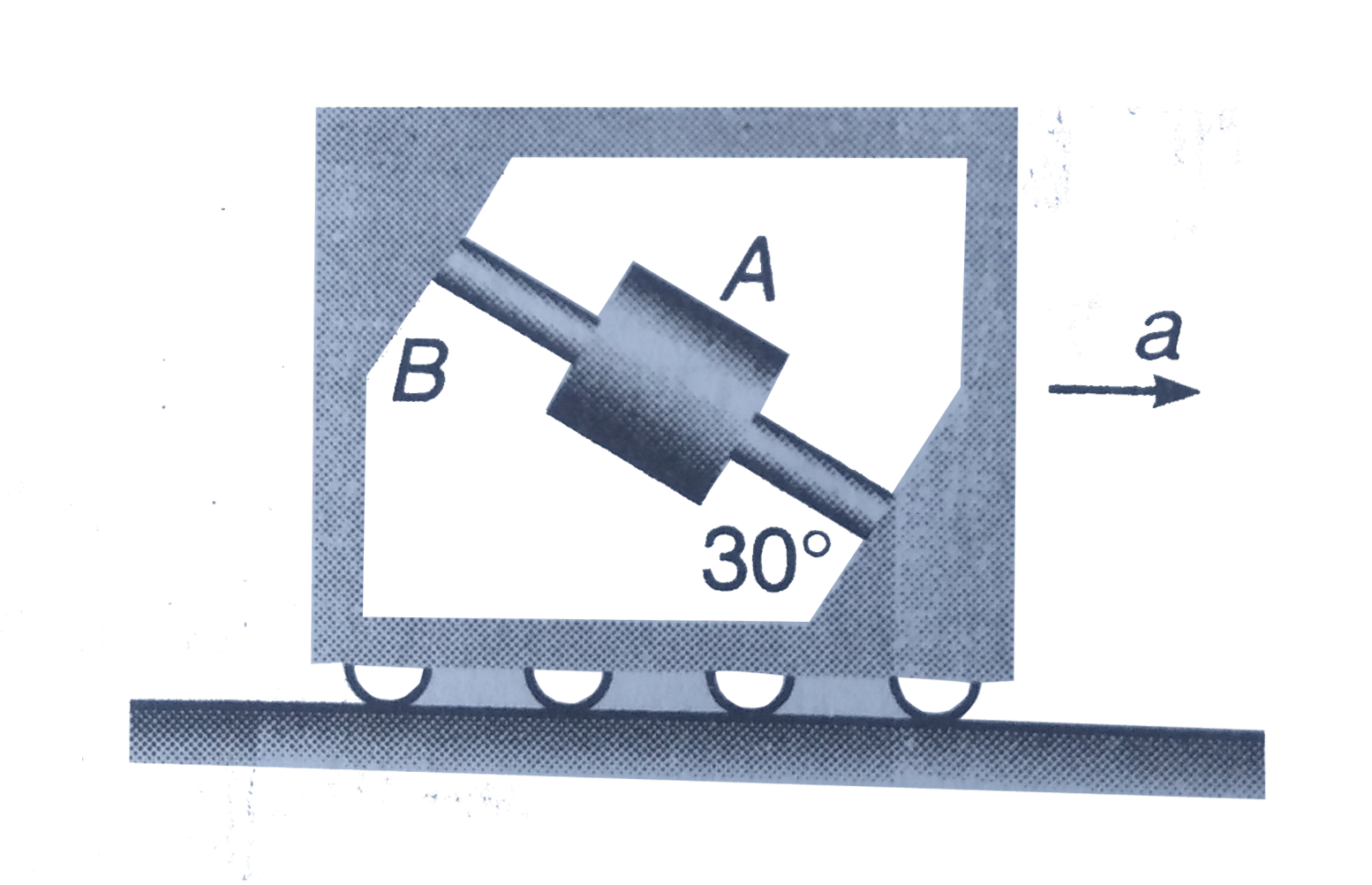
View SolutionThe collar A is free to slide along the smooth shaft B mounted in the frame. The plane of the frame is the vertical . Determine the horizontal acceleration a of the frame necessary to maintain the collar in a fixed position on the shaft. (g=9.8m/s2)
View SolutionFigur shows the total acceleration and velocity of a particle moving clockwise in a circle of radius 2.5m at a given instant of time. At this instant, find:
(a) the radius acceleration,
(b) the speed of the acceleration,
(c) its tangential acceleration.
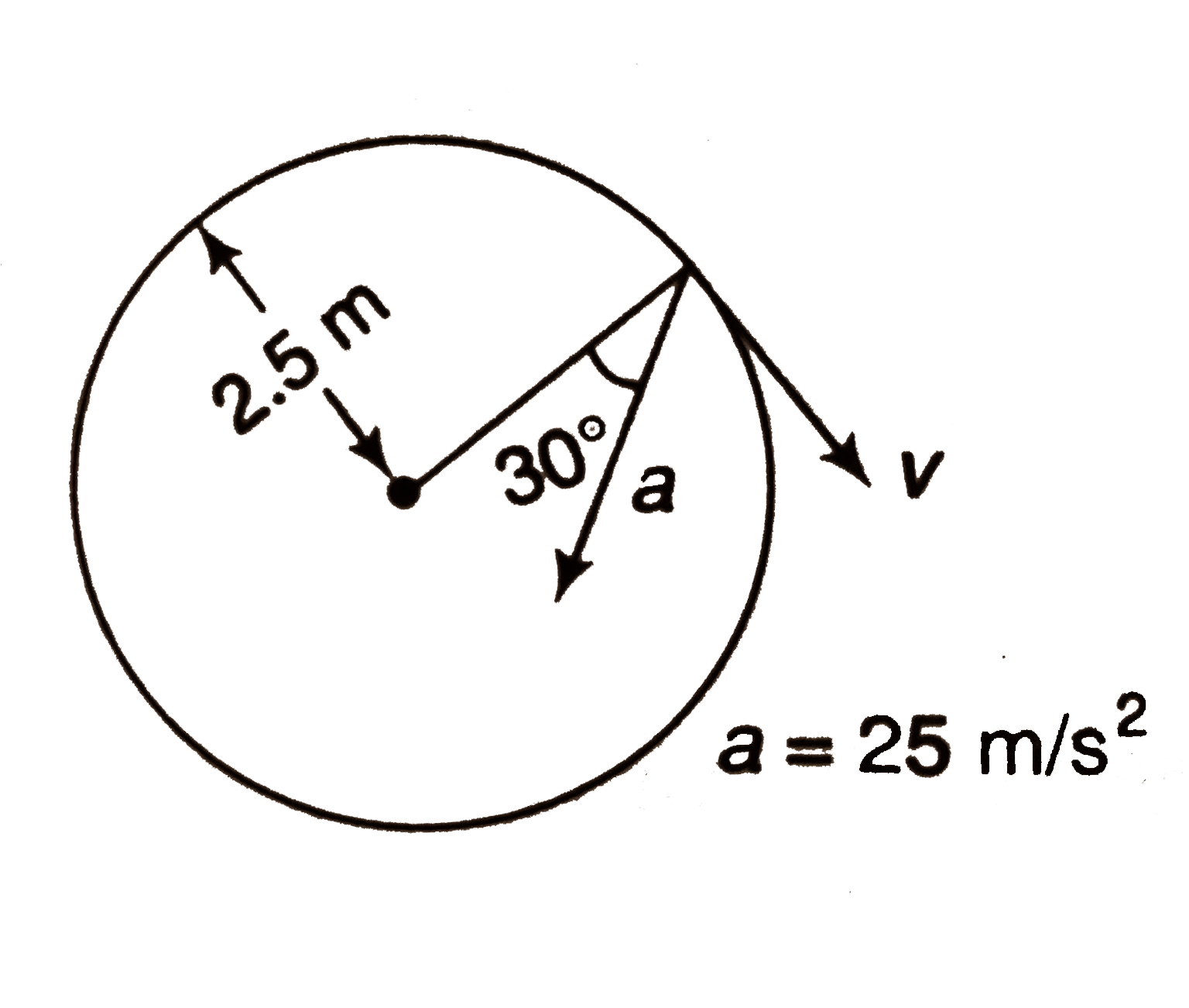
View Solution
