A uniform rod of mass 2kg and length 1m lies on a smooth horizontal plane. A particle of mass 1 kg moving at a speed of 2ms−1 perpendicular to the length of the rod strikes it at a distance 14 m from the centre and stops . Find the angular velocity of the rod about its centre just after the collision (in rad/s)
The correct Answer is:3
To solve the problem step by step, we will follow these steps:
Step 1: Understand the given data
- Mass of the rod, mrod=2kg
- Length of the rod, l=1m
- Mass of the particle, mparticle=1kg
- Speed of the particle, v=2m/s
- Distance from the center of the rod where the particle strikes, d=14m
Step 2: Calculate the initial momentum of the system
The initial momentum of the particle before the collision is given by:
pinitial=mparticle⋅v=1kg⋅2m/s=2kg m/s
Step 3: Apply the conservation of linear momentum
After the collision, the particle comes to a stop, and the rod starts moving. Let V be the velocity of the center of mass of the rod after the collision. According to the conservation of linear momentum:
pinitial=pfinal
2kg m/s=mrod⋅V
Substituting the mass of the rod:
2=2⋅V⟹V=1m/s
Step 4: Calculate the moment of inertia of the rod
The moment of inertia I of a uniform rod about its center is given by:
I=112mrodl2=112⋅2⋅(1)2=16kg m2
Step 5: Calculate the angular momentum before the collision
The angular momentum L of the particle about the center of the rod just before the collision is given by:
Linitial=mparticle⋅v⋅d=1⋅2⋅14=12kg m2/s
Step 6: Calculate the angular momentum after the collision
After the collision, the angular momentum is given by:
Lfinal=I⋅ω
where ω is the angular velocity we want to find. Setting the initial angular momentum equal to the final angular momentum:
12=16⋅ω
Step 7: Solve for angular velocity ω
Rearranging the equation gives:
ω=1/21/6=1/2⋅61=3rad/s
Conclusion
The angular velocity of the rod about its center just after the collision is ω=3rad/s.
---
- Mass of the rod, mrod=2kg
- Length of the rod, l=1m
- Mass of the particle, mparticle=1kg
- Speed of the particle, v=2m/s
- Distance from the center of the rod where the particle strikes, d=14m
The initial momentum of the particle before the collision is given by:
pinitial=mparticle⋅v=1kg⋅2m/s=2kg m/s
After the collision, the particle comes to a stop, and the rod starts moving. Let V be the velocity of the center of mass of the rod after the collision. According to the conservation of linear momentum:
pinitial=pfinal
2kg m/s=mrod⋅V
Substituting the mass of the rod:
2=2⋅V⟹V=1m/s
The moment of inertia I of a uniform rod about its center is given by:
I=112mrodl2=112⋅2⋅(1)2=16kg m2
The angular momentum L of the particle about the center of the rod just before the collision is given by:
Linitial=mparticle⋅v⋅d=1⋅2⋅14=12kg m2/s
After the collision, the angular momentum is given by:
Lfinal=I⋅ω
where ω is the angular velocity we want to find. Setting the initial angular momentum equal to the final angular momentum:
12=16⋅ω
Rearranging the equation gives:
ω=1/21/6=1/2⋅61=3rad/s
The angular velocity of the rod about its center just after the collision is ω=3rad/s.
Topper's Solved these Questions
- ROTATIONAL MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - Matrix Matching Type Questions
16 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Subjective Questions
2 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct
3 Videos
Similar Questions
A uniform rod of mass M and length a lies on a smooth horizontal plane. A particle of mass m moving at a speed v perpendicular to the length of the rod strikes it at a distance a4 from the centre and stops after the collision. Find a. the velocity of the cente of the rod and b. the angular velocity of the rod abut its centre just after the collision.
View SolutionA uniform rod of length L lies on a smooth horizontal table. The rod has a mass M. A particle of mass m moving with speed v strikes the rod perpendicularly at one of the ends of the rod sticks to it after collision.
Find the velocity of the particle with respect to C before the collision
View SolutionA uniform rod of mass m and length L is at rest on a smooth horizontal surface. A ball of mass m, moving with velocity v0, hits the rod perpendicularly at its one end and sticks to it. The angular velocity of rod after collision is

View SolutionA uniform rod AB of mass m and length l is at rest on a smooth horizontal surface. An impulse J is applied to the end B, perpendicular to the rod in the horizontal direction. Speed of particlem P at a distance l6 from the centre towards A of the rod after time t=πml12J is.
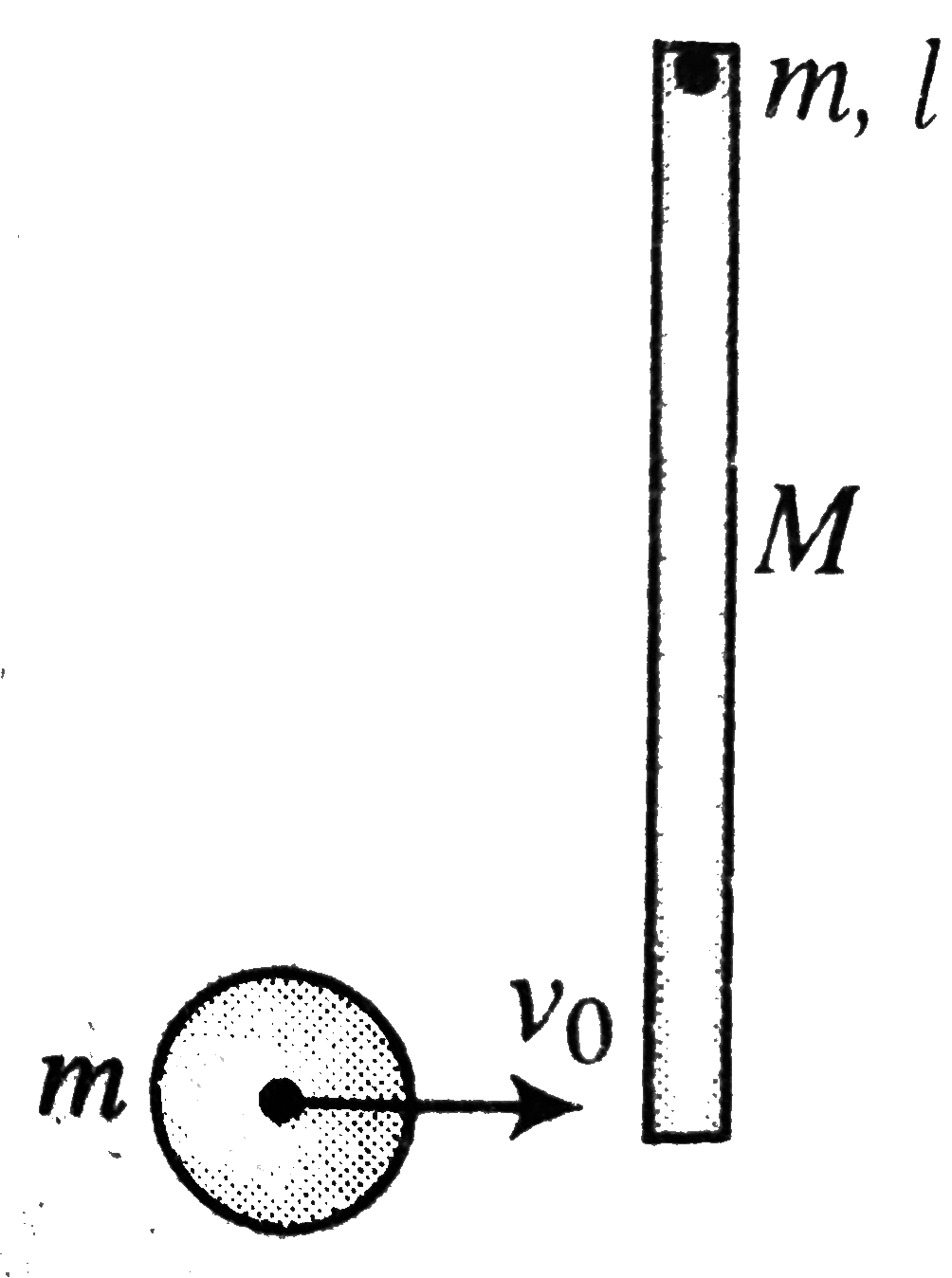
View SolutionA uniform rod of mass M and length l is placed on a smooth- horizontal surface with its one end pivoted to the surface. A small ball of mass m moving along the surface with a velocity v0, perpendicular to the rod, collides elastically with the free end of the rod. Find:
a. the angular velocity of the rod after collision.
b. the impulse applied by the pivot on the rod during collision. (Take M/m=2)
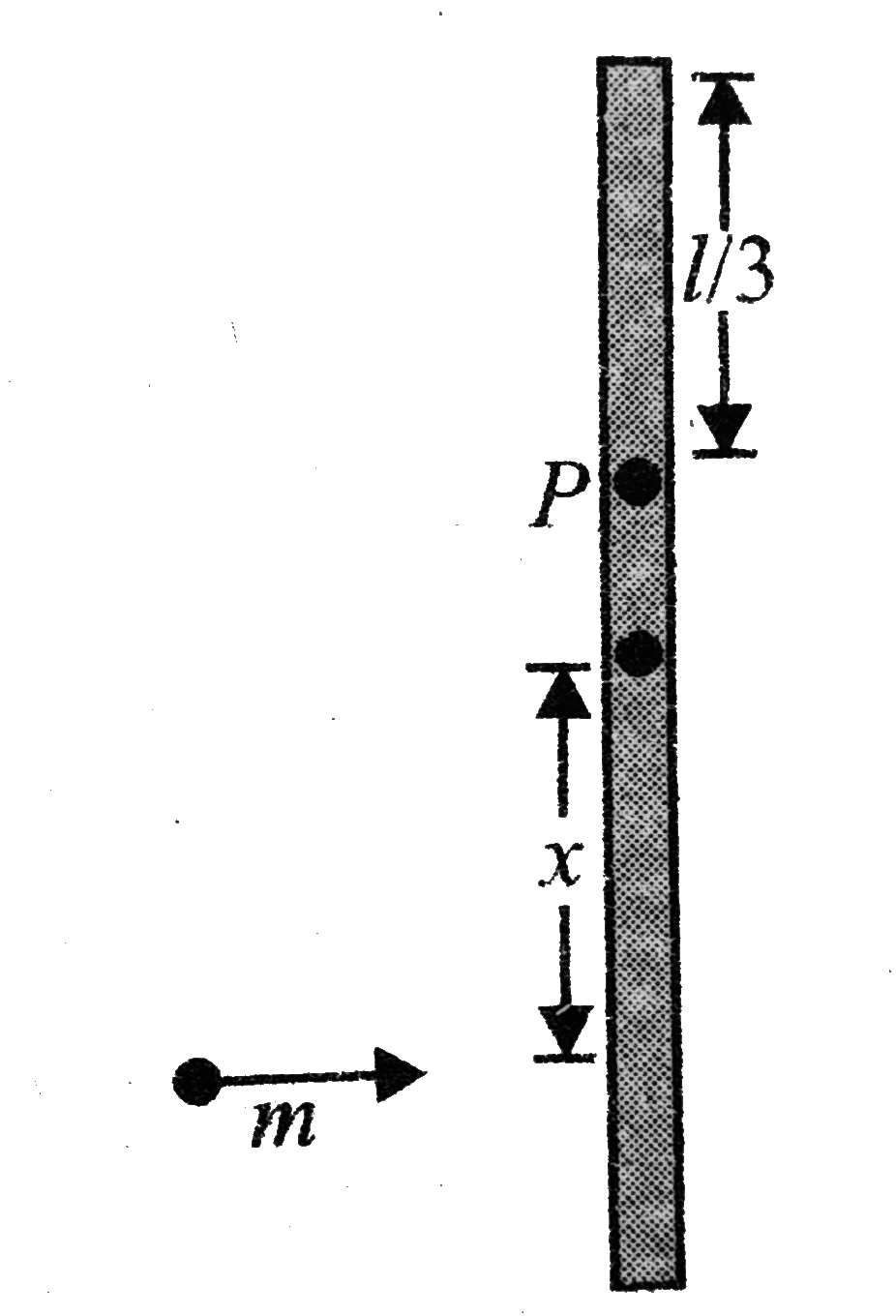
View SolutionA thin uniform rod of mass m and length l is kept on a smooth horizontal surface such that it can move freely. At what distance from centre of rod should a particle of mass m strike on the rod such that the point P at a distance l3 from the end of the rod is instantaneously at rest just after the elastic collision?
View SolutionA uniform rod of mass m=2kg and length l is kept on a smooth horizontal plane. If the ends A and B of the rod move with speeds v and 2v respectively perpendicular to the rod, find the:
a. angulr velocity of CM of the rod.
b. Kinetic energy of the rod (in Joule).

View SolutionA Iong slender rod of mass 2kg and length m is placed on a smooth horizontal table. Two particles of masses 2kg and 1kg strike the rod simultaneously and stick to the rod after collision as shown in fig.
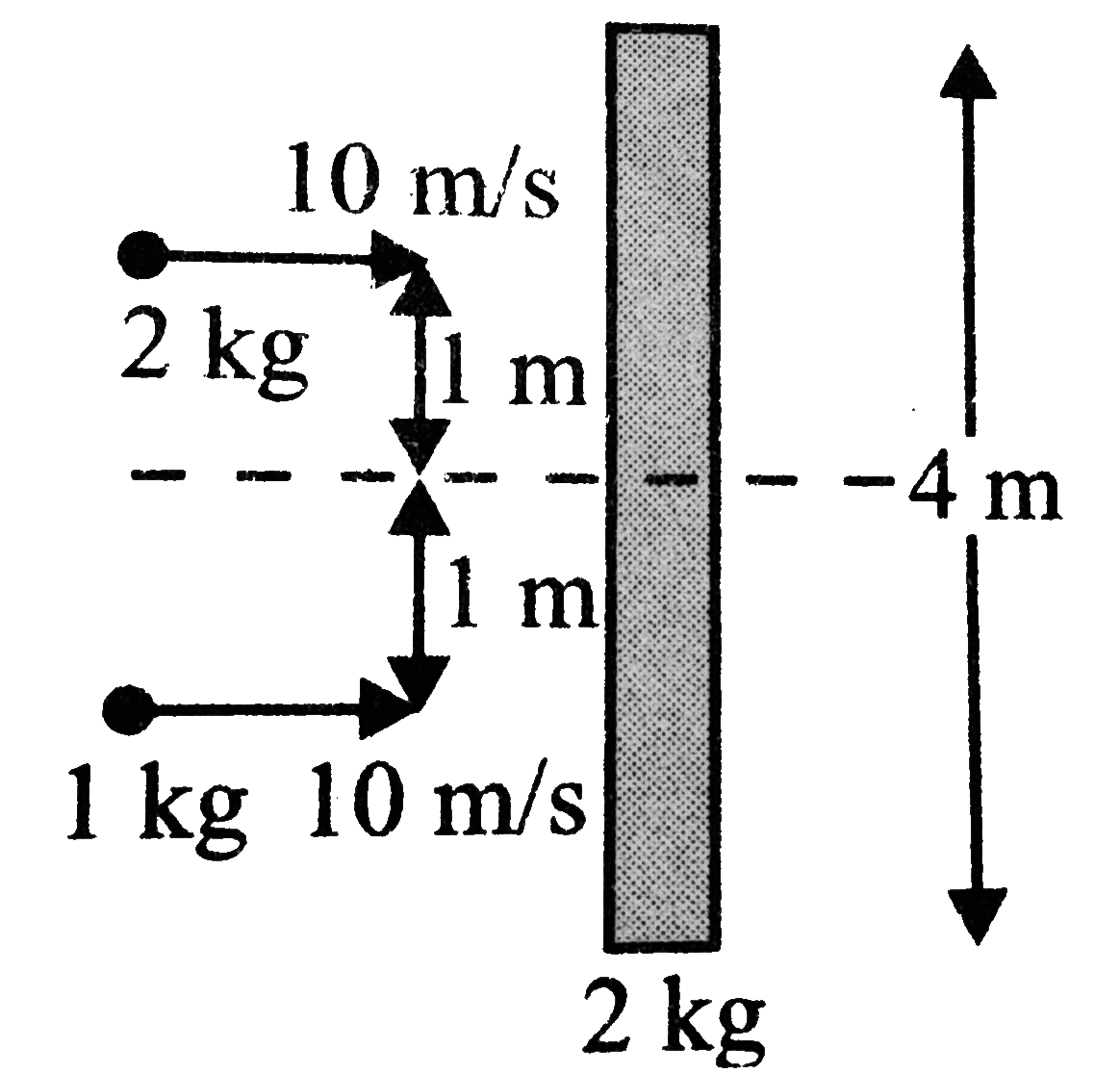
Velocity of the centre of mass of the rod after collision is
View SolutionA uniform rod of mass m, length l rests on a smooth horizontal surface. Rod is given a sharp horizontal impulse p perpendicular to the rod at a distance l/4 from the centre. The angular velocity of the rod will be
View SolutionA uniform rod of mass m and length L lies radialy on a disc rotating with angular speed ω in a horizontal plane about vertical axis passing thorugh centre of disc. The rod does not slip on the disc and the centre of the rod is at a distance 2L from the centre of the disc. them the kinetic energy of the rod is

View Solution