A 4 kg blocks A is placed on the top of 8 kg block B which rests on a smooth table .A just slips on B when a force of 12 N is applied on B to make Both A and B move together is (4x) N Find the value of x.
The correct Answer is:6
To solve the problem, we need to determine the value of x in the expression 4x N, which represents the force required to make both blocks A and B move together.
1. Identify the masses of the blocks:
- Mass of block A, mA=4kg
- Mass of block B, mB=8kg
2. Understand the condition for slipping:
- Block A slips on block B when a force of 12N is applied to block B. This force represents the maximum static friction force that can act between the two blocks.
3. Calculate the acceleration of block A:
- The force causing block A to slip is the maximum static friction force, which is 12N.
- Using Newton's second law, F=m⋅a, we can find the acceleration aA of block A:
aA=FmA=12N4kg=3m/s2
4. Determine the total force required to move both blocks together:
- When both blocks A and B move together, they will have the same acceleration. The total mass of the system (A + B) is:
mtotal=mA+mB=4kg+8kg=12kg
- The acceleration of both blocks when a force F is applied can be expressed as:
F=mtotal⋅a
- Substituting the values:
F=12kg⋅3m/s2=36N
5. Relate the total force to 4x:
- According to the problem, the total force F is also given as 4x:
4x=36N
- To find x, we solve for x:
x=36N4=9
Final Answer:
The value of x is 9.
- Mass of block A, mA=4kg
- Mass of block B, mB=8kg
- Block A slips on block B when a force of 12N is applied to block B. This force represents the maximum static friction force that can act between the two blocks.
- The force causing block A to slip is the maximum static friction force, which is 12N.
- Using Newton's second law, F=m⋅a, we can find the acceleration aA of block A:
aA=FmA=12N4kg=3m/s2
- When both blocks A and B move together, they will have the same acceleration. The total mass of the system (A + B) is:
mtotal=mA+mB=4kg+8kg=12kg
- The acceleration of both blocks when a force F is applied can be expressed as:
F=mtotal⋅a
- Substituting the values:
F=12kg⋅3m/s2=36N
- According to the problem, the total force F is also given as 4x:
4x=36N
- To find x, we solve for x:
x=36N4=9
The value of x is 9.
Similar Questions
A 4 kg block A is placed on the top of 8 kg block B which rests on a smooth table.
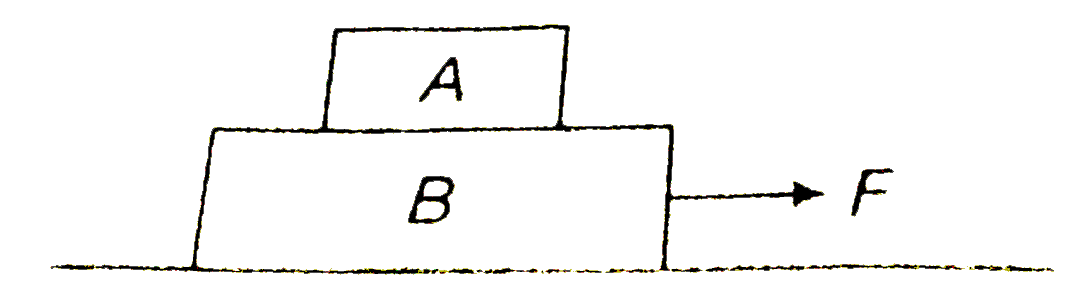
A just slips on B when a force of 12 N is applied on A. Then the maximum horizontal force F applied on B to make both A and B move together, is
View Solution12N of force required to be applied on A to slip on B. Find the maximum horizontal force F to be applied on B so that A and B moves together.

View Solution
Knowledge Check
A block A of mass 4 kg is placed on another block B of mass 5 kg and the block rests on a smooth horizontal table. For sliding the block A on B, a horizontal force of 12 N is required to be applied on it. The maximum horizontal force that can be applied on B so that both A and B move together and the acceleration produced by this force is
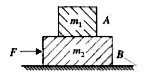 A25 N, 5 m/s2B27 N, 3 m/s2C30 N, 8 m/s2Dcannot be calculated from the given data.
A25 N, 5 m/s2B27 N, 3 m/s2C30 N, 8 m/s2Dcannot be calculated from the given data.
A 5 kg block is placed on the top of a 15 kg block as shown in figure . A horizontal force of 60 N is applied to the 15 kg block and the 5kg block is tied to the wall . The coefficient of friction between moving surfaces is 0.2

(a) Draw the free body diagram for each block w.r.t ground and identify the action reaction forces between two blocks .
(b) Determine the tension in the string and magnitude of the acceleration of the 15 kg block .
View SolutionA block A of mass 4 kg is placed on another block B of mass 5 kg , and the block B rests on a smooth horizontal table . For sliding block A on B , a horizontal force of 12 N is required to be applied on A How much maximum force can be applied on B so that both A and B move togrther ? Also find out acceleration produced by this force .
View SolutionA block A of mass 4 kg is placed on another block B of mass 5 kg , and the block B rests on a smooth horizontal table . For sliding block A on B , a horizontal force of 12 N is required to be applied on A How much maximum force can be applied on B so that both A and B move togrther ? Also find out acceleration produced by this force .
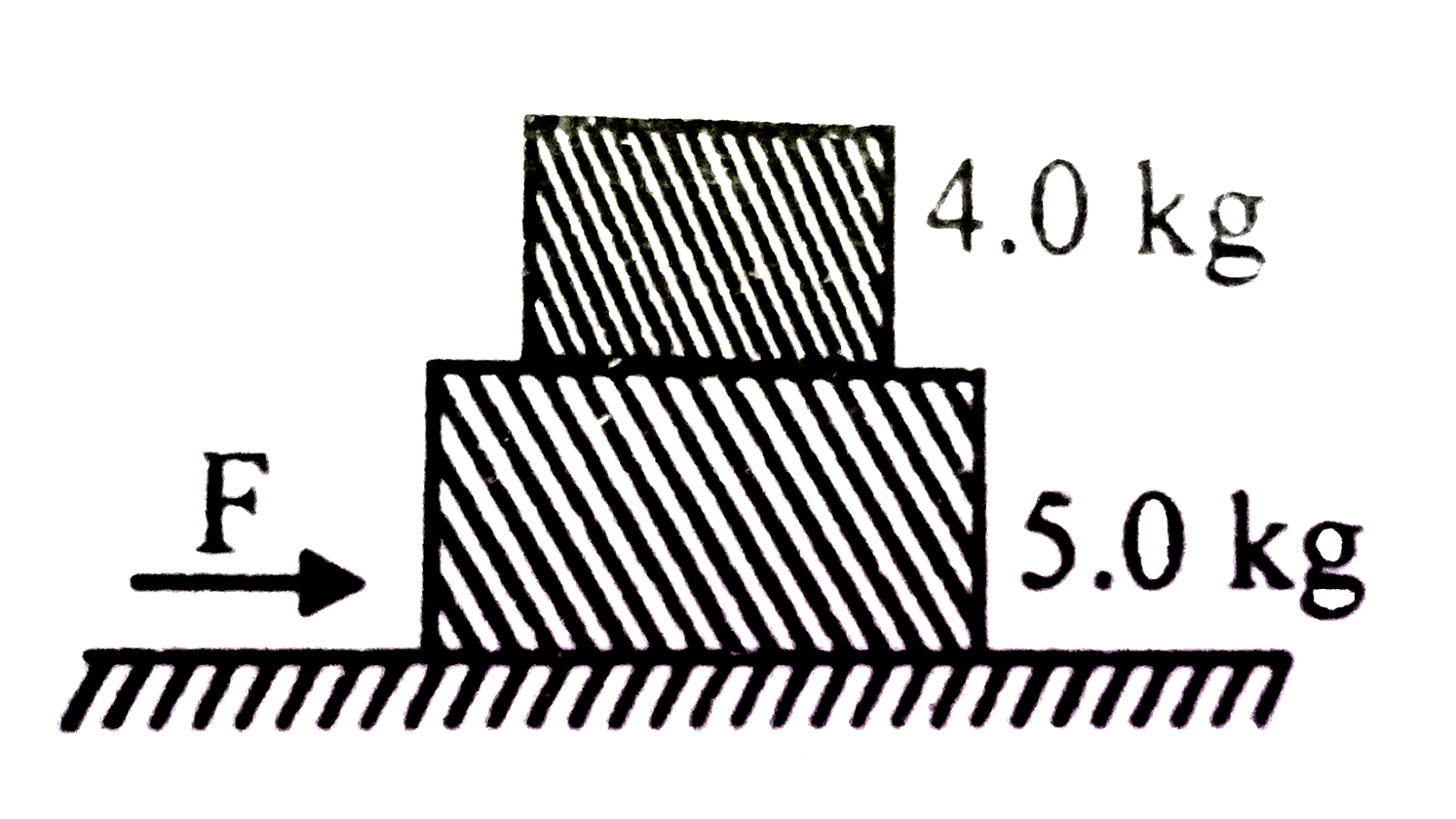
View SolutionA 4.0 kg block is put on top of a 5.0kg block. In order to cause the top block to slip on the bottom one, a horizontal force of 12N must be applied to the top block. Assuming a friction less table the maximum horizontal force F which can be applied to the lower block so that the blocks, will move together should be
View SolutionTwo blocks A and B are placed one over the other on a smooth horizontal surface. The maximum horizontal force that can be applied on the lower block A, so that A and B move without separation is 49N. The coefficient of friction between A and B is (take g = 9.8ms−2 )
View SolutionTwo blocks of mass 2 kg and 4 kg are kept in contact with each other on a smooth horizontal surface. A horizontal force of 12 N is applied on the first block due to which they move with certain constant acceleration. Calculate the force between the blocks.
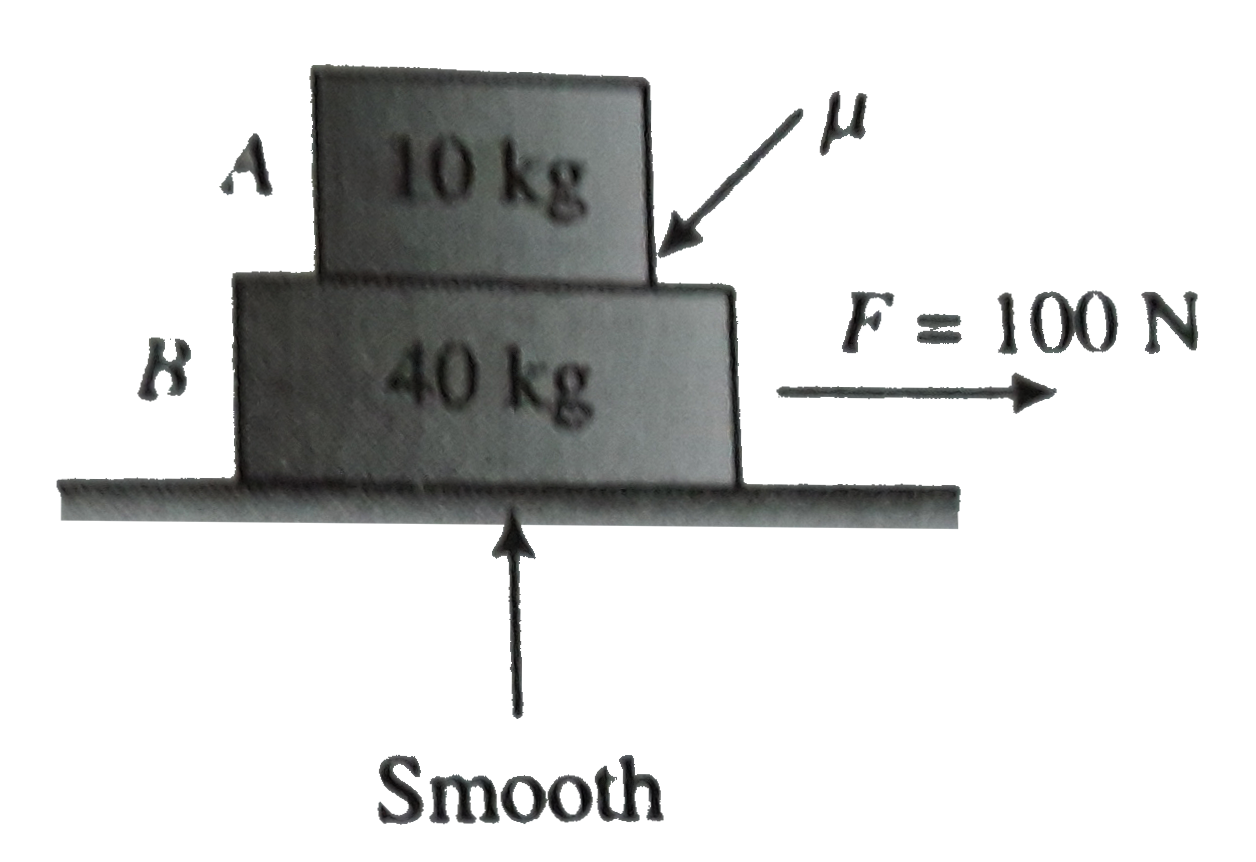
View SolutionA 10kg block is placed on top of a 40kg block as shown in figure .A horizontal force F acting on B cause an acceleration of 2ms−2 to B .For this situation marks our the correct statement(s)
View Solution
