A skater of mass m standing on ice throws a stone of mass M with a velocity of v in a horizontal direction. The distance over which the skater will move back (the coefficient of friction between the skater and the ice is )
To solve the problem, we will follow these steps:
Step 1: Understand the scenario
A skater of mass m throws a stone of mass M with a velocity v in a horizontal direction. When the skater throws the stone, he will experience a backward motion due to the conservation of momentum.
Step 2: Apply conservation of momentum
Before throwing the stone, the total momentum of the system (skater + stone) is zero. After the stone is thrown, the momentum of the stone is Mv, and the momentum of the skater is −mu (where u is the velocity of the skater in the backward direction).
Using the conservation of momentum:
0=Mv−mu
Rearranging gives:
mu=Mv⇒u=Mvm
Step 3: Calculate the frictional force
The skater experiences a frictional force when he moves backward. The frictional force Ff can be expressed as:
Ff=μN
where N is the normal force. Since the skater is on ice and there are no vertical accelerations, N=mg. Therefore:
Ff=μmg
Step 4: Determine the acceleration of the skater
Using Newton's second law, the net force acting on the skater is equal to the mass of the skater multiplied by its acceleration a:
ma=−Ff⇒ma=−μmg
Thus, the acceleration a of the skater is:
a=−μg
Step 5: Use the equations of motion to find the distance
We will use the equation of motion:
v2=u2+2as
Here, the final velocity v=0 (the skater comes to rest), the initial velocity u=Mvm, and the acceleration a=−μg. Substituting these values into the equation gives:
0=(Mvm)2+2(−μg)s
Rearranging gives:
(Mvm)2=2μgs
Solving for s:
s=(Mv)22μgm2
Final Result
Thus, the distance over which the skater will move back is:
s=M2v22μgm2
A skater of mass m throws a stone of mass M with a velocity v in a horizontal direction. When the skater throws the stone, he will experience a backward motion due to the conservation of momentum.
Before throwing the stone, the total momentum of the system (skater + stone) is zero. After the stone is thrown, the momentum of the stone is Mv, and the momentum of the skater is −mu (where u is the velocity of the skater in the backward direction).
0=Mv−mu
Rearranging gives:
mu=Mv⇒u=Mvm
The skater experiences a frictional force when he moves backward. The frictional force Ff can be expressed as:
Ff=μN
where N is the normal force. Since the skater is on ice and there are no vertical accelerations, N=mg. Therefore:
Ff=μmg
Using Newton's second law, the net force acting on the skater is equal to the mass of the skater multiplied by its acceleration a:
ma=−Ff⇒ma=−μmg
Thus, the acceleration a of the skater is:
a=−μg
We will use the equation of motion:
v2=u2+2as
Here, the final velocity v=0 (the skater comes to rest), the initial velocity u=Mvm, and the acceleration a=−μg. Substituting these values into the equation gives:
0=(Mvm)2+2(−μg)s
Rearranging gives:
(Mvm)2=2μgs
Solving for s:
s=(Mv)22μgm2
Thus, the distance over which the skater will move back is:
s=M2v22μgm2
Topper's Solved these Questions
- CENTRE OF MASS, IMPULSE AND MOMENTUMBook:DC PANDEY ENGLISHChapter:CENTRE OF MASS, IMPULSE AND MOMENTUMExercise:More a than one option is Correct
Explore 1 Video
- CENTRE OF MASS, IMPULSE AND MOMENTUMBook:DC PANDEY ENGLISHChapter:CENTRE OF MASS, IMPULSE AND MOMENTUMExercise:Comprehension type questions
Explore 15 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBook:DC PANDEY ENGLISHChapter:CENTRE OF MASS, IMPULSE AND MOMENTUMExercise:Comprehension type questions
Explore 15 Videos
- CENTRE OF MASSBook:DC PANDEY ENGLISHChapter:CENTRE OF MASSExercise:Medical entrances gallery
Explore 27 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBook:DC PANDEY ENGLISHChapter:CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONExercise:Level 2 Subjective
Explore 21 Videos
Similar Questions
A block of mass m slides over a smooth wedge of mass M which is placed over a rough horizontal surface . The centre of mass of the system will move towards left

Here μ = coefficient of friction between the wedge and the ground .
View SolutionAn ice skater moving at 10m/s comes to a halt in 100m on a ice surface. Calculate the coefficient of friction between the ice and the skater ?
View Solution
Knowledge Check
A vehicle of mass M is moving on a rough horizontal road with a momentum P If the coefficient of friction between the tyres and the road is μ is then the stopping distance is .
Ap2μmgBp22μmgCp2μm2gDp22μm2g
What is the minimum stopping distance for a vehicle of mass m moving with speed v along a level road. If the coefficient of friction between the tyres and the road is μ.
View SolutionA block slides on ice with a velocity of 5m/s comes to rest after moving through a distance of 13.5m . Find the coefficient of friction ?
View SolutionA vehicle of mass m is moving on a rough horizontal road with kinetic energy 'E'. If the co-efficient of friction between the tyres and the road be μ. Then the stopping distance is,
View SolutionFind the minimum value of horizontal force (F) required on the block of mass m to keep it at rest on the wall. Given the coefficient of friction between the surfaces is μ.
View SolutionA man of mass 60 kg sitting on ice pushes a block of mass of 12kg on ice horizontally with a speed of 5 ms−1. The coefficient of friction between the man and ice and between block and ice is 0.2. If g =10 ms−2, the distances between man and the block, when they come to rest is
View SolutionA stone of 1kg is thrown with a velocity of 20ms−1 across the frozen surface of lake and comes to rest after travelling a distance of 50m. What is the force of friction between the stone and the ice?
View SolutionA plank A of mass M rests on a smooth horizontal surface over which it can move without friction A cabe B of mass m lies on the plank at one edge .The coefficient of friction between the plank and the cube is μ The size of cube is very small in comparison to the plank.
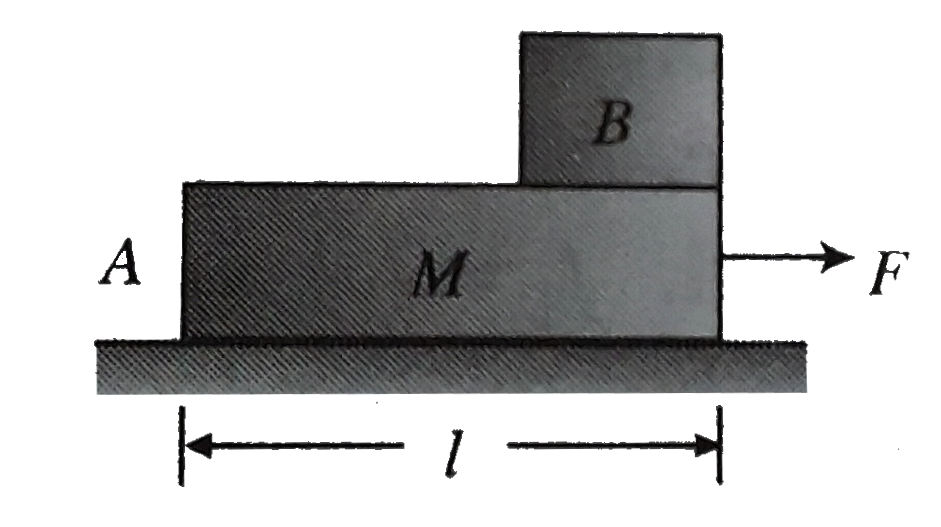
At what force F applied to the plank in the horizontal direction will be cube begin to slide towards the other end of the plank?
View Solution