A glass tube 80cm long and open ends is half immersed in mercury. Then the top of the tube is closed and it is taken out of the mercury. A column of murcury 20cm long then remains in the tube. The atmospheric (in cm of Hg) is
(assume temperature to be constant )
75
30
60
90
The correct Answer is:C
To solve the problem step by step, let's analyze the situation described:
1. Understanding the Setup:
- We have a glass tube that is 80 cm long, and it is half immersed in mercury. This means that 40 cm of the tube is submerged in mercury, while the other 40 cm is above the mercury level.
- When the tube is closed at the top and removed from the mercury, we are told that a column of mercury 20 cm long remains in the tube.
2. Initial Conditions:
- When the tube is half immersed in mercury, the pressure at the bottom of the tube (where it is submerged) is equal to the atmospheric pressure plus the pressure due to the mercury column above it.
- The height of the mercury column in the tube when it is immersed is 40 cm (the submerged part), and the pressure exerted by this column can be calculated using the formula:
P=ρgh
- Here, ρ is the density of mercury, g is the acceleration due to gravity, and h is the height of the mercury column (40 cm).
3. Taking the Tube Out of Mercury:
- After closing the top of the tube and removing it from the mercury, the pressure inside the tube will remain constant because the air inside the tube does not escape.
- The column of mercury that remains in the tube is now 20 cm long.
4. Calculating the Atmospheric Pressure:
- The atmospheric pressure can be represented as:
P0=Pinside+Pmercury
- The pressure inside the tube (when closed) is equal to the pressure exerted by the remaining mercury column (20 cm) plus the pressure due to the air column above it.
- The total height of the mercury column when the tube is half immersed is 40 cm, and when taken out, we have 20 cm remaining. Therefore, the atmospheric pressure can be calculated as:
P0=ρg(hinitial+hremaining)=ρg(40cm+20cm)=ρg(60cm)
5. Final Answer:
- The atmospheric pressure in cm of mercury is 60 cm.
Thus, the atmospheric pressure is 60 cm of Hg.
- We have a glass tube that is 80 cm long, and it is half immersed in mercury. This means that 40 cm of the tube is submerged in mercury, while the other 40 cm is above the mercury level.
- When the tube is closed at the top and removed from the mercury, we are told that a column of mercury 20 cm long remains in the tube.
- When the tube is half immersed in mercury, the pressure at the bottom of the tube (where it is submerged) is equal to the atmospheric pressure plus the pressure due to the mercury column above it.
- The height of the mercury column in the tube when it is immersed is 40 cm (the submerged part), and the pressure exerted by this column can be calculated using the formula:
P=ρgh
- Here, ρ is the density of mercury, g is the acceleration due to gravity, and h is the height of the mercury column (40 cm).
- After closing the top of the tube and removing it from the mercury, the pressure inside the tube will remain constant because the air inside the tube does not escape.
- The column of mercury that remains in the tube is now 20 cm long.
- The atmospheric pressure can be represented as:
P0=Pinside+Pmercury
- The pressure inside the tube (when closed) is equal to the pressure exerted by the remaining mercury column (20 cm) plus the pressure due to the air column above it.
- The total height of the mercury column when the tube is half immersed is 40 cm, and when taken out, we have 20 cm remaining. Therefore, the atmospheric pressure can be calculated as:
P0=ρg(hinitial+hremaining)=ρg(40cm+20cm)=ρg(60cm)
- The atmospheric pressure in cm of mercury is 60 cm.
Topper's Solved these Questions
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - More than one option is correct
21 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Comprehension
32 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Integer
8 Videos
- PROJECTILE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - PROJECTILE MOTIONEXERCISE - Level - 2 Subjective
10 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Integer type q.
14 Videos
Similar Questions
In given figure, A uniform cylindrical tube closed at one end, contains a pallet of mercury 5cm long. When the tube is kept verticaly with the closed end downward, the length of the air column trapped is 30cm. Now the tube is inverted so that the closed end goes up find the new length of the air column trapped. atmospheric pressure =75cm of mercury. (Assume temperature remains constant in this process)

View SolutionThe thickness of a metallic tube is 1cm and the inner diameter of the tube is 12cm. Find the mass of 1m long tube, if the density of the metal be 7.8 gm/cm3
View SolutionThe thickness of a metallic tube is 1cm and the inner diameter of the tube is 12cm. Find the mass of 1m long tube, if the density of the metal be 7.8 gm/cm3
View SolutionA uniform tube closed at aone end, contains a pellet of mercury 10cm long. When the tube is lept vertically with the closed-end upward, the length of the air column trapped is 20cm. Find the length of the air column trapped when the tube is inverted so that the closed-end goes down. Atmospheric pressure=75cm of mercury.
View SolutionThe lower end of a capillary tube is immersed in mercury. The level of mercury in the tube is found to be 2 cm below the outer level. If the same tube is immersed in water, up to what height will the water rise in the capillary ?
View SolutionIn a vertical U - tube a column of mercury oscillates simple harmonically. If the tube contains 1kg of mercury and 1cm of mercury column weighs 20g, then the period of oscillation is
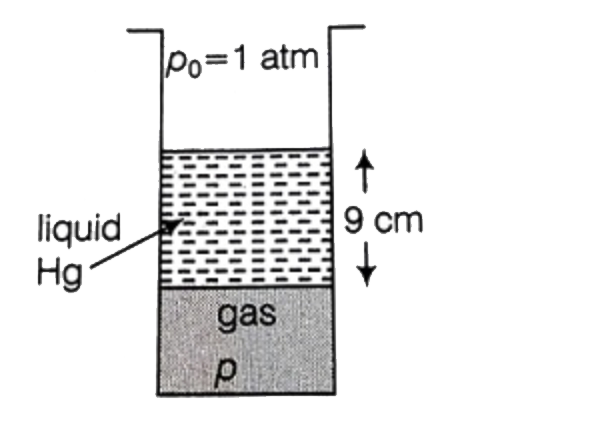
View SolutionIn the given figure, atmospheric pressure p0=1 atm and mercury column length is 9 cm. Pressure p of the gas enclosed in the tube is
View SolutionA hollow square-shaped tube open at both ends is made of iron. The internal square is of 5 cm side and the length of the tube is 8 cm. There are 192 cm3 of iron in this tube. Find its thickness
View SolutionA hollow square-shaped tube open at both ends is made of iron. The internal square is of 5cm side and the length of the tube is 8cm. There are 192cm3 of iron in this tube. Find its thickness.
View SolutionAn open glass tube is immersed in mercury in such a way that a length of 8 cm extends above the mercury level. The open end of the tube is then closed and sealed and the tube is raised vertically up by additional 46 cm. What will be length of the air column above mercury in the tube now? (atmospheric pressure =76 cm of Hg)
View Solution
