A ring of radius R is made of a thin wire of material of density ρ, having cross-section area a and Young's modulus y. The ring rotates about an axis perpendicular to its plane and through its centre. Angular frequency of rotation is ω.
The ratio of kinetic energy to potential energy is
yρR2ω2
2yρR2ω2
y2ρR2ω2
y4ρR2ω2
The correct Answer is:A
To find the ratio of kinetic energy (KE) to potential energy (PE) for a rotating ring, we can follow these steps:
Step 1: Calculate the Kinetic Energy (KE)
The kinetic energy of the ring can be calculated using the formula:
KE=12Iω2
where I is the moment of inertia of the ring and ω is the angular frequency.
For a thin ring of radius R, the moment of inertia I is given by:
I=mR2
where m is the mass of the ring. The mass can be calculated using the density ρ, cross-sectional area a, and the circumference of the ring:
m=ρ⋅a⋅L=ρ⋅a⋅(2πR)
Thus, substituting for m:
I=(ρ⋅a⋅2πR)R2=2πρaR3
Now substituting I back into the kinetic energy formula:
KE=12(2πρaR3)ω2=πρaR3ω2
Step 2: Calculate the Potential Energy (PE)
The potential energy stored in the material of the ring due to its elongation can be calculated using the formula:
PE=12T⋅ΔLL
where T is the tension, ΔL is the change in length, and L is the original length.
The tension T can be related to the Young's modulus Y as:
T=Y⋅AL⋅ΔL
The elongation ΔL can be expressed as:
ΔL=T⋅LY⋅A
Substituting for T:
ΔL=(Y⋅A⋅ΔL)⋅LY⋅A=T⋅LY⋅A
Now, substituting this back into the potential energy formula:
PE=12Y⋅A⋅T⋅LY⋅A=12⋅T2⋅LY⋅A
Using the earlier expression for T:
PE=12⋅(Y⋅A⋅ΔL)2⋅LY⋅A
After simplification, we find:
PE=12⋅Y⋅A⋅(2πR)2⋅ρ2⋅ω2Y
This leads to:
PE=12⋅2π2R2ρ2ω2AY
Step 3: Calculate the Ratio of KE to PE
Now, we can find the ratio of kinetic energy to potential energy:
KEPE=πρaR3ω212⋅2π2R2ρ2ω2AY
This simplifies to:
KEPE=2πρaR3ω2Y2π2R2ρ2ω2A
Canceling out common terms:
KEPE=YρR2
Final Answer
Thus, the ratio of kinetic energy to potential energy is:
KEPE=YρR2
The kinetic energy of the ring can be calculated using the formula:
KE=12Iω2
where I is the moment of inertia of the ring and ω is the angular frequency.
I=mR2
where m is the mass of the ring. The mass can be calculated using the density ρ, cross-sectional area a, and the circumference of the ring:
m=ρ⋅a⋅L=ρ⋅a⋅(2πR)
Thus, substituting for m:
I=(ρ⋅a⋅2πR)R2=2πρaR3
Now substituting I back into the kinetic energy formula:
KE=12(2πρaR3)ω2=πρaR3ω2
The potential energy stored in the material of the ring due to its elongation can be calculated using the formula:
PE=12T⋅ΔLL
where T is the tension, ΔL is the change in length, and L is the original length.
T=Y⋅AL⋅ΔL
The elongation ΔL can be expressed as:
ΔL=T⋅LY⋅A
Substituting for T:
ΔL=(Y⋅A⋅ΔL)⋅LY⋅A=T⋅LY⋅A
Now, substituting this back into the potential energy formula:
PE=12Y⋅A⋅T⋅LY⋅A=12⋅T2⋅LY⋅A
Using the earlier expression for T:
PE=12⋅(Y⋅A⋅ΔL)2⋅LY⋅A
After simplification, we find:
PE=12⋅Y⋅A⋅(2πR)2⋅ρ2⋅ω2Y
This leads to:
PE=12⋅2π2R2ρ2ω2AY
Now, we can find the ratio of kinetic energy to potential energy:
KEPE=πρaR3ω212⋅2π2R2ρ2ω2AY
This simplifies to:
KEPE=2πρaR3ω2Y2π2R2ρ2ω2A
Canceling out common terms:
KEPE=YρR2
Thus, the ratio of kinetic energy to potential energy is:
KEPE=YρR2
Topper's Solved these Questions
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Matrix Matching
12 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Integer
8 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - More than one option is correct
21 Videos
- PROJECTILE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - PROJECTILE MOTIONEXERCISE - Level - 2 Subjective
10 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Integer type q.
14 Videos
Similar Questions
A ring of radius R is made of a thin wire of material of density ρ, having cross-section area a and Young's modulus y. The ring rotates about an axis perpendicular to its plane and through its centre. Angular frequency of rotation is ω.
The tension in the ring will be
View SolutionA ring of radius R is made of a thin wire of material of density ρ having cross section area a. The ring rotates with angular velocity ω about an axis passing through its centre and perpendicular to the plane. If we consider a small element of the ring,it rotates in a circle. The required centripetal force is provided by the component of tensions on the element towards the centre. A small element of length dl of angular width dθ is shown in the figure.
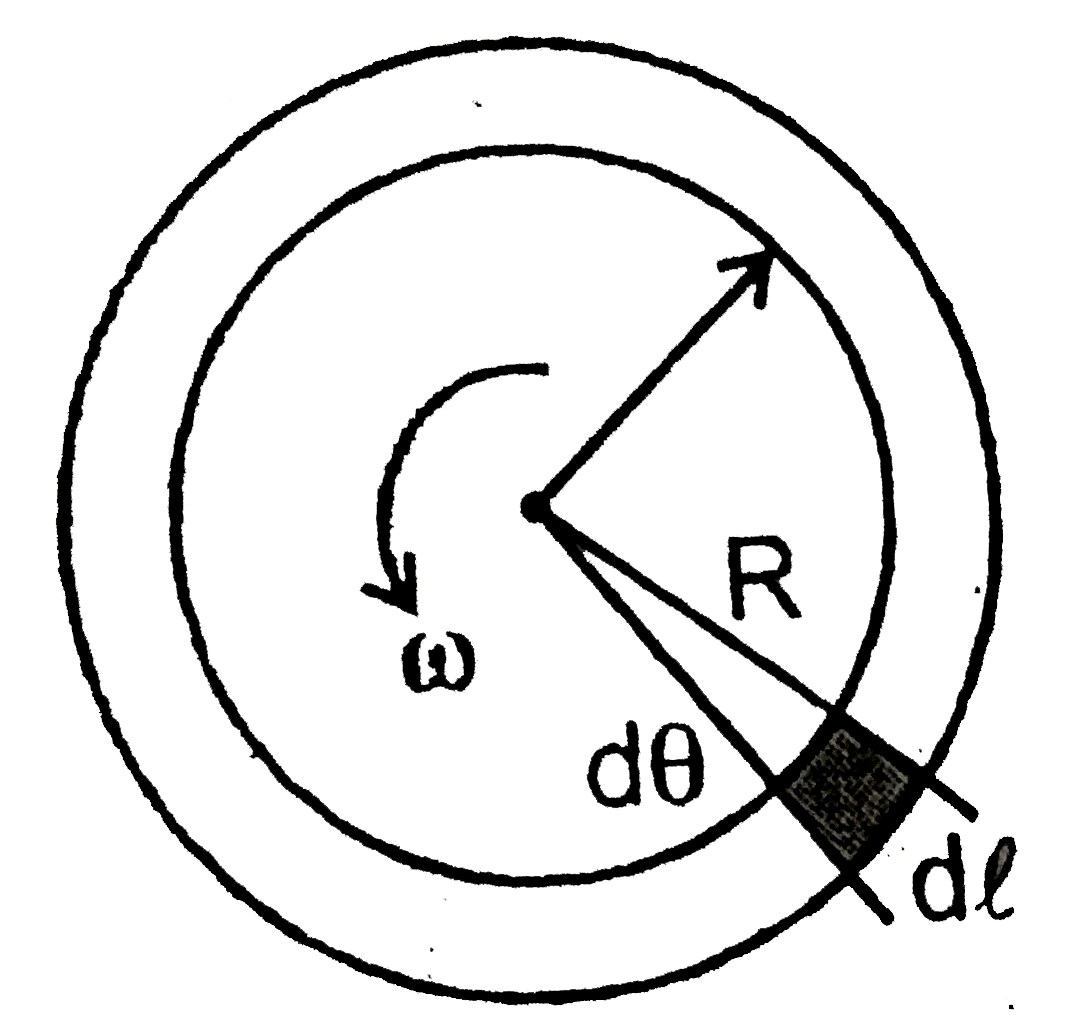
If T is the tension in the ring, then
View SolutionA ring of radius R is made of a thin wire of material of density ρ having cross section area a. The ring rotates with angular velocity ω about an axis passing through its centre and perpendicular to the plane. If we consider a small element of the ring,it rotates in a circle. The required centripetal force is provided by the component of tensions on the element towards the centre. A small element of length dl of angular width dθ is shown in the figure.

If for a given mass of the ring and angular velocity, the radius R of the ring is increased to 2R, the new tension will be
View SolutionA thin ring of radius R is made of a material of density ρ and Young's modulus Y. If the ring is rotated about its centre in its own plane with angular velocity ω , find the small increase in its radius.
View SolutionCircular disc of mass 2 kg and radius 1 m is rotating about an axis perpendicular to its plane and passing through its centre of mass with a rotational kinetic energy of 8 J. The angular momentum is (Js) is
View SolutionA flywheel of mass 0.2 kg and radius 10 cm is rotating with 5πrevs about an axis perpendicular to its plane passing through its centre. Calculate angular momentum and kinetic energy of flywheel.
View SolutionA flywheel having a radius of gyration of 2m and mass 10 kg rotates at an angular speed of 5rads−1 about an axis perpendicular to it through its centre. The kinetic energy of rotation is
View SolutionA block of mass M is suspended from a wire of length L, area of cross-section A and Young's modulus Y. The elastic potential energy stored in the wire is
View SolutionA ring of mass m and radius r rotates about an axis passing through its centre and perpendicular to its plane with angular velocity ω. Its kinetic energy is
View SolutionA solid sphere of radius r and mass m rotates about an axis passing through its centre with angular velocity ω. Its K.E. Is
View Solution
