A thin ring of radius R is made of a material of density ρ and Young's modulus Y. If the ring is rotated about its centre in its own plane with angular velocity ω , find the small increase in its radius.
The correct Answer is:A, B, C, D
To find the small increase in the radius of a thin ring of radius R, made of a material with density ρ and Young's modulus Y, when it is rotated about its center in its own plane with angular velocity ω, we can follow these steps:
Step 1: Understand the Forces Acting on the Ring
When the ring rotates, each element of the ring experiences a centripetal force due to its circular motion. This force is provided by the tension in the ring.
Step 2: Consider an Element of the Ring
Take a small element of the ring. The tension T acts tangentially to the ring. For a small angle θ, the horizontal components of the tension from both sides of the element will contribute to the net force acting towards the center of the ring.
Step 3: Set Up the Equation for Centripetal Force
The net force acting towards the center due to the tension can be expressed as:
Fnet=2Tsin(θ)
For small angles, sin(θ)≈θ. Thus, we can write:
Fnet≈2Tθ
Step 4: Relate the Net Force to Centripetal Force
The centripetal force required for the mass dm of the element moving in a circle of radius R is given by:
Fcentripetal=dm⋅ω2R
Step 5: Calculate the Mass of the Element
The mass dm of the small element can be expressed in terms of the density ρ and the geometry of the ring:
dm=ρ⋅dV=ρ⋅(dL⋅A)
where dL is the length of the element and A is the cross-sectional area. The length of the element can be approximated as R⋅dθ, so:
dm=ρ⋅(R⋅dθ)⋅A
Step 6: Equate Forces
Setting the net force equal to the centripetal force gives:
2Tθ=ρ(R⋅dθ)A⋅ω2R
Step 7: Solve for Tension T
From the equation above, we can isolate T:
T=ρAω2R2θ2θ=ρAω2R22
Step 8: Relate Tension to Stress and Strain
The tension in the ring produces a stress, which is related to strain through Young's modulus Y:
Stress=TA=Y⋅Strain
The strain is defined as:
Strain=ΔRR
Thus, we can write:
TA=Y⋅ΔRR
Step 9: Substitute for Tension
Substituting our expression for T into the equation gives:
ρAω2R22A=Y⋅ΔRR
This simplifies to:
ρω2R22=Y⋅ΔRR
Step 10: Solve for the Increase in Radius ΔR
Rearranging the equation to solve for ΔR:
ΔR=ρω2R32Y
Final Result
Thus, the small increase in the radius of the ring when it is rotated with angular velocity ω is:
ΔR=ρω2R32Y
When the ring rotates, each element of the ring experiences a centripetal force due to its circular motion. This force is provided by the tension in the ring.
Take a small element of the ring. The tension T acts tangentially to the ring. For a small angle θ, the horizontal components of the tension from both sides of the element will contribute to the net force acting towards the center of the ring.
The net force acting towards the center due to the tension can be expressed as:
Fnet=2Tsin(θ)
For small angles, sin(θ)≈θ. Thus, we can write:
Fnet≈2Tθ
The centripetal force required for the mass dm of the element moving in a circle of radius R is given by:
Fcentripetal=dm⋅ω2R
The mass dm of the small element can be expressed in terms of the density ρ and the geometry of the ring:
dm=ρ⋅dV=ρ⋅(dL⋅A)
where dL is the length of the element and A is the cross-sectional area. The length of the element can be approximated as R⋅dθ, so:
dm=ρ⋅(R⋅dθ)⋅A
Setting the net force equal to the centripetal force gives:
2Tθ=ρ(R⋅dθ)A⋅ω2R
From the equation above, we can isolate T:
T=ρAω2R2θ2θ=ρAω2R22
The tension in the ring produces a stress, which is related to strain through Young's modulus Y:
Stress=TA=Y⋅Strain
The strain is defined as:
Strain=ΔRR
Thus, we can write:
TA=Y⋅ΔRR
Substituting our expression for T into the equation gives:
ρAω2R22A=Y⋅ΔRR
This simplifies to:
ρω2R22=Y⋅ΔRR
Rearranging the equation to solve for ΔR:
ΔR=ρω2R32Y
Thus, the small increase in the radius of the ring when it is rotated with angular velocity ω is:
ΔR=ρω2R32Y
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - ELASTICITYEXERCISE - Exercise 15.14videos
ELASTICITY
- BOOK - DC PANDEY ENGLISHCHAPTER - ELASTICITYEXERCISE - Exercise 15.22videos
ELASTICITY
- BOOK - DC PANDEY ENGLISHCHAPTER - ELASTICITYEXERCISE - Solved Examples6videos
ELASTICITY
- BOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - All Questions469videos
CURRENT ELECTRICITY
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Integer17videos
ELECTROSTATICS
Similar Questions
A ring of radius R is made of a thin wire of material of density ρ, having cross-section area a and Young's modulus y. The ring rotates about an axis perpendicular to its plane and through its centre. Angular frequency of rotation is ω.
The tension in the ring will beView SolutionA ring of radius R is made of a thin wire of material of density ρ, having cross-section area a and Young's modulus y. The ring rotates about an axis perpendicular to its plane and through its centre. Angular frequency of rotation is ω.
The ratio of kinetic energy to potential energy isView SolutionA ring of radius R is made of a thin wire of material of density ρ having cross section area a. The ring rotates with angular velocity ω about an axis passing through its centre and perpendicular to the plane. If we consider a small element of the ring,it rotates in a circle. The required centripetal force is provided by the component of tensions on the element towards the centre. A small element of length dl of angular width dθ is shown in the figure.
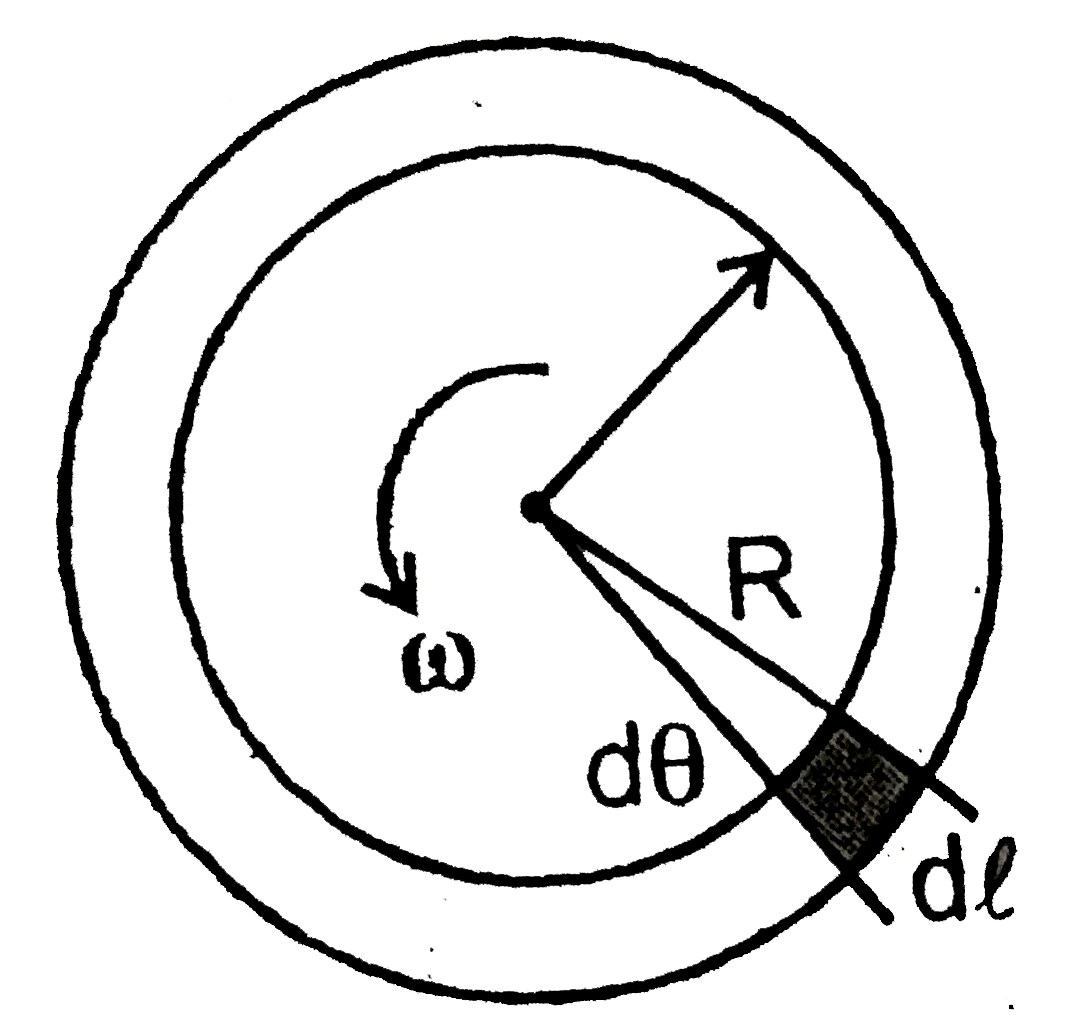
If T is the tension in the ring, thenView SolutionA ring of radius R is made of a thin wire of material of density ρ having cross section area a. The ring rotates with angular velocity ω about an axis passing through its centre and perpendicular to the plane. If we consider a small element of the ring,it rotates in a circle. The required centripetal force is provided by the component of tensions on the element towards the centre. A small element of length dl of angular width dθ is shown in the figure.

If for a given mass of the ring and angular velocity, the radius R of the ring is increased to 2R, the new tension will beView SolutionA ring of mass m and radius R is being rotated about its axis with angular velocity o. If o increases then tension in ring
View SolutionA thin disc of radius R has charge Q distributed uniformly on its surface. The disc is rotated about one of its diametric axis with angular velocity ω. The magnetic moment of the arrangement is
View SolutionA ring of mass m and radius r rotates about an axis passing through its centre and perpendicular to its plane with angular velocity ω. Its kinetic energy is
View Solutionconsider a nonconducting ring of radius r and mass m which has a total charge q distributed uniformly on it the ring is rotated about its axis with an angular speed ω. (a) Find the equivalent electric current in the ring (b) find the magnetic moment μ of the . (c) show thatμ=q2m where l is the angular momentum of the ring about its axis of rotation.
View SolutionA solid sphere of radius r and mass m rotates about an axis passing through its centre with angular velocity ω. Its K.E. Is
View SolutionA ring of radius 'r' and mass per unit length 'm' rotates with an angular velocity 'ω' in free space then tension will be :
View Solution

