A ring of radius R is made of a thin wire of material of density ρ, having cross-section area a and Young's modulus y. The ring rotates about an axis perpendicular to its plane and through its centre. Angular frequency of rotation is ω.
The tension in the ring will be
aρR2ω22
aρR2ω2
2aρR2ω2
aρR2ω24
The correct Answer is:B
To find the tension in the rotating ring, we can follow these steps:
Step 1: Understanding the System
We have a ring of radius R made of a thin wire with density ρ, cross-sectional area a, and Young's modulus y. The ring rotates about an axis perpendicular to its plane with an angular frequency ω.
Step 2: Consider a Small Segment of the Ring
Let's take a small segment of the ring that subtends an angle θ at the center. The length of this small segment is given by:
Length=Rθ
Step 3: Calculate the Mass of the Small Segment
The volume of this small segment can be calculated as:
Volume=Area×Length=a⋅(Rθ)=aRθ
Thus, the mass dm of this small segment is:
dm=ρ⋅Volume=ρ⋅(aRθ)=ρaRθ
Step 4: Forces Acting on the Segment
The tension T in the wire acts on both ends of the small segment. The net force acting towards the center due to the tension can be derived from the components of the tension:
Fnet=2Tcos(θ2)
For small angles, we can approximate cos(θ2)≈1, so:
Fnet≈2T
Step 5: Relate the Net Force to Centripetal Force
The net force acting towards the center must equal the centripetal force required to keep the mass moving in a circle:
Fnet=dm⋅v2R
where v is the linear velocity. Since v=Rω, we can write:
Fnet=dm⋅(Rω)2R=dm⋅Rω2
Step 6: Substitute dm into the Equation
Substituting dm=ρaRθ into the centripetal force equation gives:
2T=(ρaRθ)Rω2
Step 7: Solve for Tension T
Now, we can simplify this equation:
2T=ρaR2ω2θ
Dividing both sides by 2θ:
T=ρaR2ω2θ2θ=ρaR2ω22
Final Answer
Thus, the tension in the ring is:
T=ρaRω2
We have a ring of radius R made of a thin wire with density ρ, cross-sectional area a, and Young's modulus y. The ring rotates about an axis perpendicular to its plane with an angular frequency ω.
Let's take a small segment of the ring that subtends an angle θ at the center. The length of this small segment is given by:
Length=Rθ
The volume of this small segment can be calculated as:
Volume=Area×Length=a⋅(Rθ)=aRθ
Thus, the mass dm of this small segment is:
dm=ρ⋅Volume=ρ⋅(aRθ)=ρaRθ
The tension T in the wire acts on both ends of the small segment. The net force acting towards the center due to the tension can be derived from the components of the tension:
Fnet=2Tcos(θ2)
For small angles, we can approximate cos(θ2)≈1, so:
Fnet≈2T
The net force acting towards the center must equal the centripetal force required to keep the mass moving in a circle:
Fnet=dm⋅v2R
where v is the linear velocity. Since v=Rω, we can write:
Fnet=dm⋅(Rω)2R=dm⋅Rω2
Substituting dm=ρaRθ into the centripetal force equation gives:
2T=(ρaRθ)Rω2
Now, we can simplify this equation:
2T=ρaR2ω2θ
Dividing both sides by 2θ:
T=ρaR2ω2θ2θ=ρaR2ω22
Thus, the tension in the ring is:
T=ρaRω2
Topper's Solved these Questions
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Matrix Matching
12 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Integer
8 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - More than one option is correct
21 Videos
- PROJECTILE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - PROJECTILE MOTIONEXERCISE - Level - 2 Subjective
10 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Integer type q.
14 Videos
Similar Questions
A ring of radius R is made of a thin wire of material of density ρ, having cross-section area a and Young's modulus y. The ring rotates about an axis perpendicular to its plane and through its centre. Angular frequency of rotation is ω.
The ratio of kinetic energy to potential energy is
View SolutionA ring of radius R is made of a thin wire of material of density ρ having cross section area a. The ring rotates with angular velocity ω about an axis passing through its centre and perpendicular to the plane. If we consider a small element of the ring,it rotates in a circle. The required centripetal force is provided by the component of tensions on the element towards the centre. A small element of length dl of angular width dθ is shown in the figure.
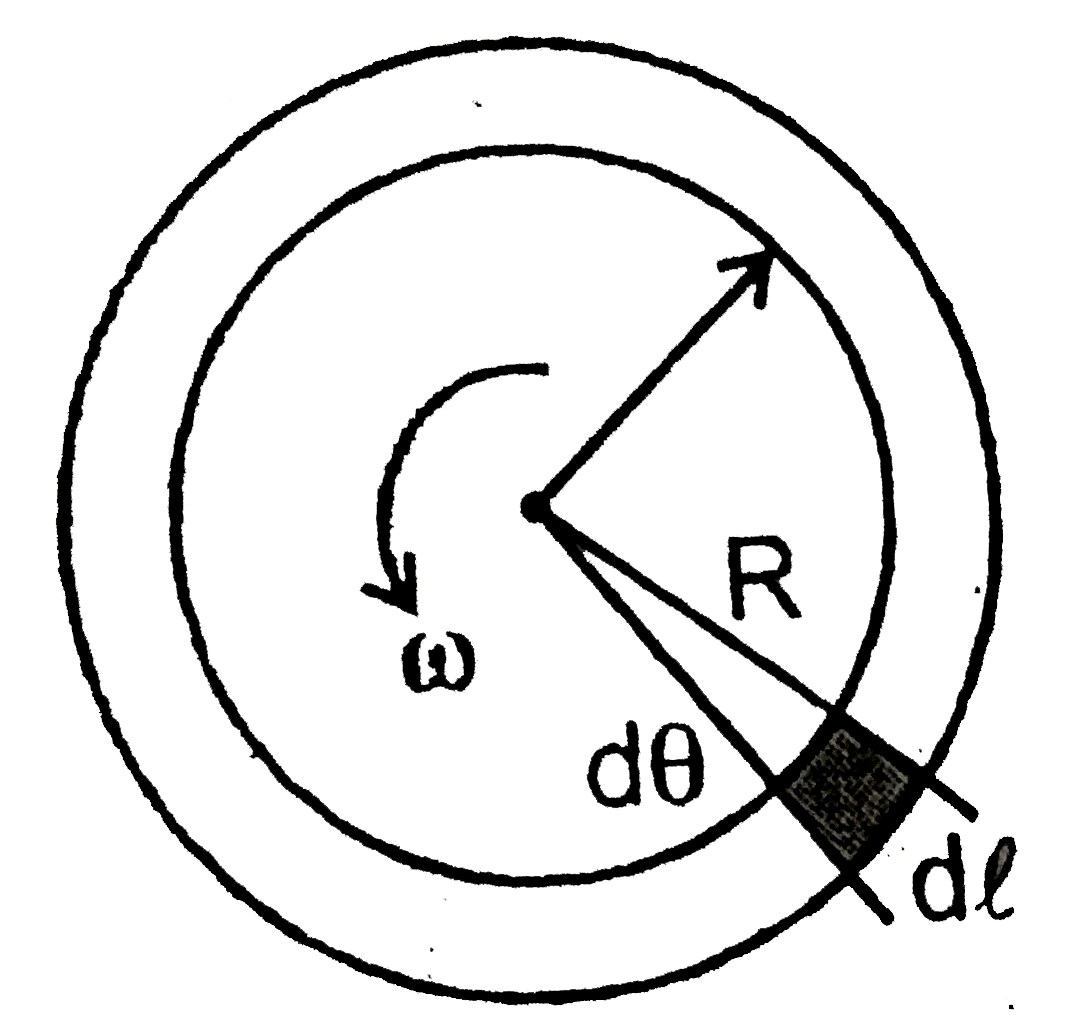
If T is the tension in the ring, then
View Solution
Knowledge Check
A wire of length L and cross-sectional area A is made of a material of Young's modulus Y. IF the wire is stretched by an amount x, the workdone is
AYAx22LBYAx2L2CYAx2LDYAx2L
A ring of radius R is made of a thin wire of material of density ρ having cross section area a. The ring rotates with angular velocity ω about an axis passing through its centre and perpendicular to the plane. If we consider a small element of the ring,it rotates in a circle. The required centripetal force is provided by the component of tensions on the element towards the centre. A small element of length dl of angular width dθ is shown in the figure.

If for a given mass of the ring and angular velocity, the radius R of the ring is increased to 2R, the new tension will be
View SolutionA thin ring of radius R is made of a material of density ρ and Young's modulus Y. If the ring is rotated about its centre in its own plane with angular velocity ω , find the small increase in its radius.
View SolutionThe M.I. of a ring of mass M and radius R about a tangential axis perpendicular to its plane is :
View SolutionA uniform rod of length l, mass m, cross-sectional area A and Young's modulus Y is rotated in horizontal plane about a fixed vertical axis passing through one end, with a constant angular velocity ω. Find the total extension in the rod due to the tension produced in the rod.
View SolutionA solid sphere of radius r and mass m rotates about an axis passing through its centre with angular velocity ω. Its K.E. Is
View SolutionA ring of radius r made of wire of density ρ is rotated about a stationary vertical axis passing through its centre and perpendicular to the plane of the ring as shown in the figure. Determine the angular velocity (in rad/s) of ring at which the ring breaks. The wire breaks at tensile stress σ. Ignore gravity. Take σ/ρ=4 and r=1m.
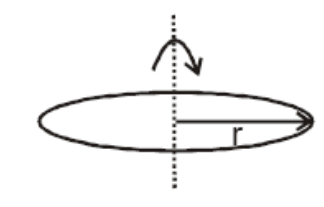
View SolutionA ring of radius r made of wire of density ρ is rotated about a stationary vertical axis passing through its centre and perpendicular to the plane of the ring as shown in the figure. Determine the angular velocity (in rad/s) of ring at which the ring breaks. The wire breaks at tensile stress σ. Ignore gravity. Take σ/ρ=4 and r=1m.

View Solution
