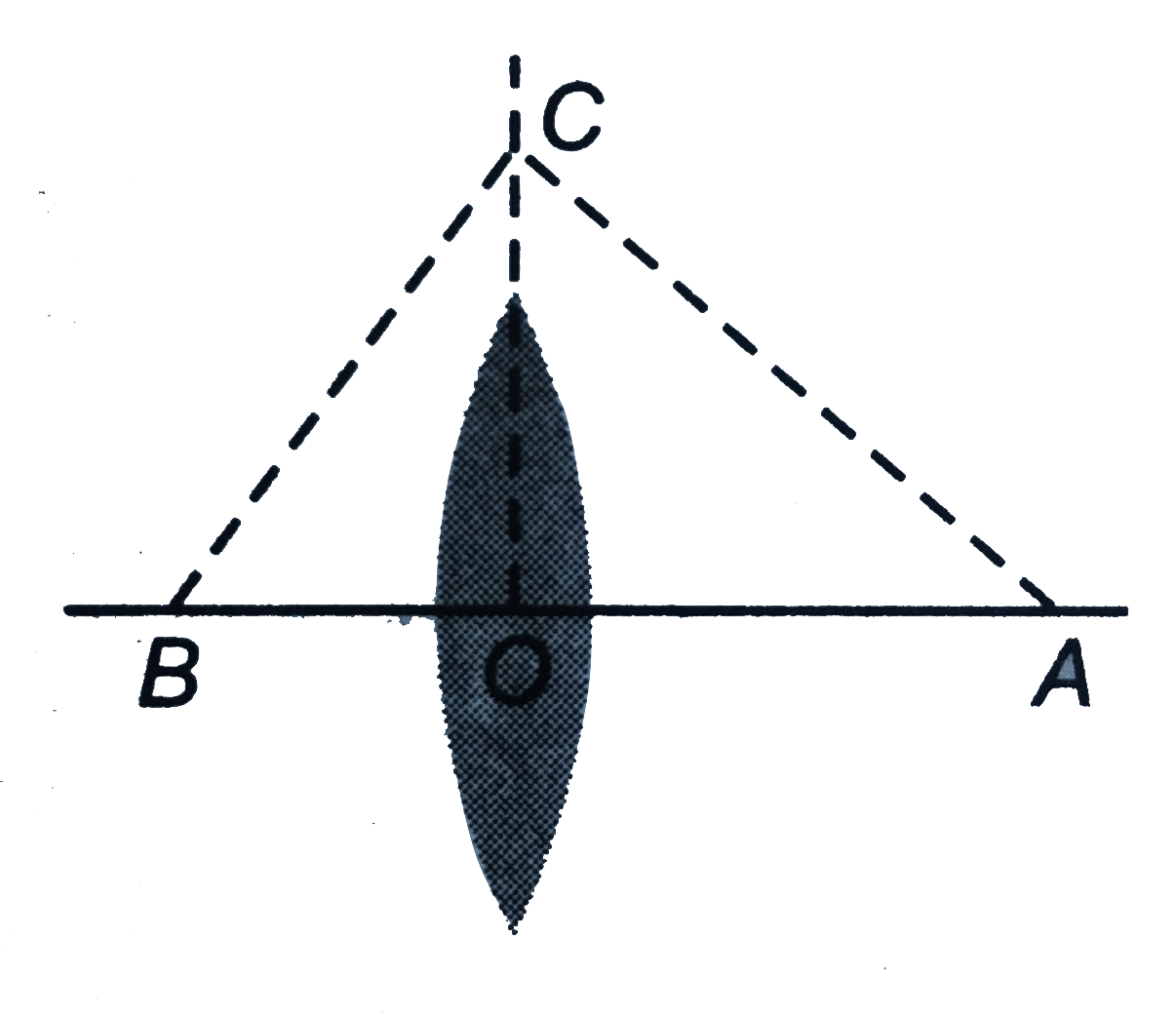
An object is placed at A(OA>f). Here, f is the focal length of the lens. The image is formed at B. A perpendicular is erected at O and C is chosen such that ∠BCA=90∘. Let OA = a, OB = b and OC = c. Then the value of f is
(a+b)3c2
(a+b)c(a+c)
c2a+b
a2a+b+c
The correct Answer is:C
To solve the problem step by step, we will follow the concepts of lens optics and the Pythagorean theorem.
Step 1: Understanding the Setup
We have an object placed at point A, and the distance from the optical center O to A is denoted as OA = a. The image is formed at point B, with OB = b. A perpendicular is erected at O, and point C is chosen such that angle BCA = 90°.
Step 2: Use the Lens Formula
The lens formula is given by:
1v−1u=1f
where:
- v is the image distance (OB = b)
- u is the object distance (OA = -a, as per the sign convention for lenses)
- f is the focal length of the lens.
Since OA is greater than f, we can write:
1b+1a=1f
This simplifies to:
a+bab=1f
Thus, we can express f as:
f=aba+b
Step 3: Apply the Pythagorean Theorem
In triangle ABC, since angle BCA = 90°, we can apply the Pythagorean theorem:
AB2=AC2+BC2
Here, AB is the hypotenuse, which is the distance from A to B, and can be expressed as:
AB=OA+OB=a+b
Let OC=c. Therefore, we have:
(a+b)2=c2+b2
Step 4: Expand and Rearrange
Expanding the left side:
a2+2ab+b2=c2+b2
Now, we can cancel b2 from both sides:
a2+2ab=c2
This can be rearranged to:
c2=a2+2ab
Step 5: Relate c to f
From the previous step, we can express f in terms of c:
f=c2a+b
Step 6: Substitute for c^2
We already found that:
c2=a2+2ab
Substituting this into the expression for f:
f=a2+2aba+b
Step 7: Final Simplification
Now, we can simplify this expression:
f=a2+2aba+b=a(a+2b)a+b
This gives us the final expression for the focal length f.
Conclusion
Thus, the value of f is:
f=c2a+b
We have an object placed at point A, and the distance from the optical center O to A is denoted as OA = a. The image is formed at point B, with OB = b. A perpendicular is erected at O, and point C is chosen such that angle BCA = 90°.
The lens formula is given by:
1v−1u=1f
where:
- v is the image distance (OB = b)
- u is the object distance (OA = -a, as per the sign convention for lenses)
- f is the focal length of the lens.
1b+1a=1f
This simplifies to:
a+bab=1f
Thus, we can express f as:
f=aba+b
In triangle ABC, since angle BCA = 90°, we can apply the Pythagorean theorem:
AB2=AC2+BC2
Here, AB is the hypotenuse, which is the distance from A to B, and can be expressed as:
AB=OA+OB=a+b
Let OC=c. Therefore, we have:
(a+b)2=c2+b2
Expanding the left side:
a2+2ab+b2=c2+b2
Now, we can cancel b2 from both sides:
a2+2ab=c2
This can be rearranged to:
c2=a2+2ab
From the previous step, we can express f in terms of c:
f=c2a+b
We already found that:
c2=a2+2ab
Substituting this into the expression for f:
f=a2+2aba+b
Now, we can simplify this expression:
f=a2+2aba+b=a(a+2b)a+b
This gives us the final expression for the focal length f.
Thus, the value of f is:
f=c2a+b
Topper's Solved these Questions
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - B. More than one option is correct
20 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - C. comprehension type question
14 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Integer type q.
14 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Integer
8 Videos
- ROTATIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - (C) Chapter Exercises
39 Videos
Similar Questions
If an object is placed at A(OA>f), where f is the focal length of the lens, the image is formed at B. A perpendicular is erected at O and C is chosen on it such that the angle (∠BCA) is a right angle. Then, the value of f will be
View SolutionAn object is placed at a distance of f2 from a convex lens of focal length f. The image will be
View SolutionAn object is placed at a distance f in front of a concave lens of focal length f. Locate its image.
View SolutionAn object is placed at f/2 away from first focus of a convex lens where f is the focal length of the lens. Its image is formed at a distance 3f/2 in a slab of refractive index 3/2 , from the face of the slab facing the lens. Find the distance of this face of the slab from the second focus of the lens.
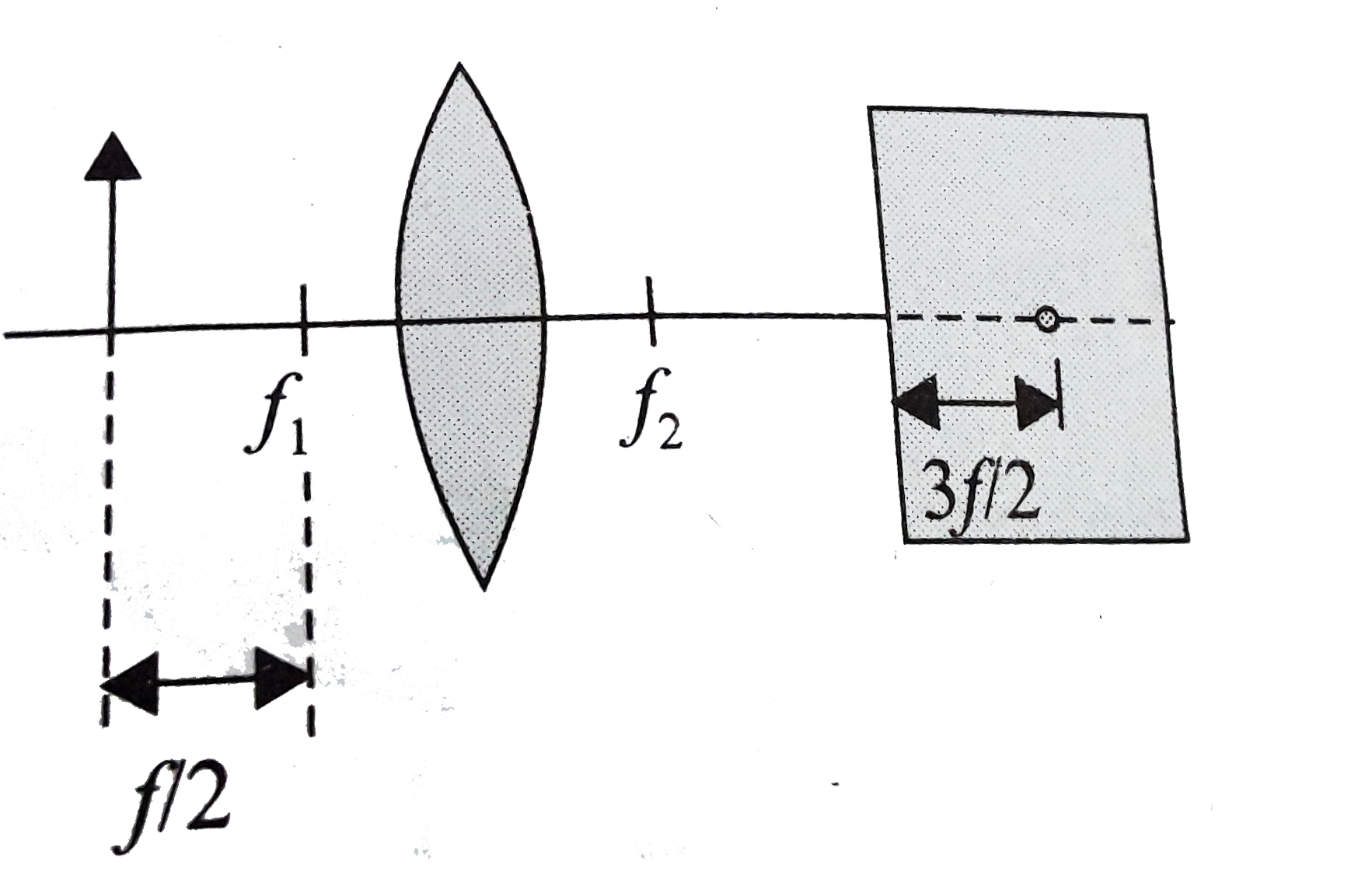
View SolutionAn object is placed at a distance f in the front of a convex mirror. If focal length of the mirror is f, then distance of image from pole of the mirror is
View SolutionA linear object is placed along the axis of a mirror as shown in figure. If 'f' is the focal length of the mirror then the length of image is-

View SolutionAn object is placed in front of a concave mirror of focal length f as shown in figure. Choose the correct shape of the image.

View SolutionAn object of length 2.5 cm is placed at a distance of 1.5 f from a concave mirror where f is the magnitude of the focal length of the mirror. The length of the object is perpendicular to the principal axis. Find the length of the image. Is the image erect to inverted?

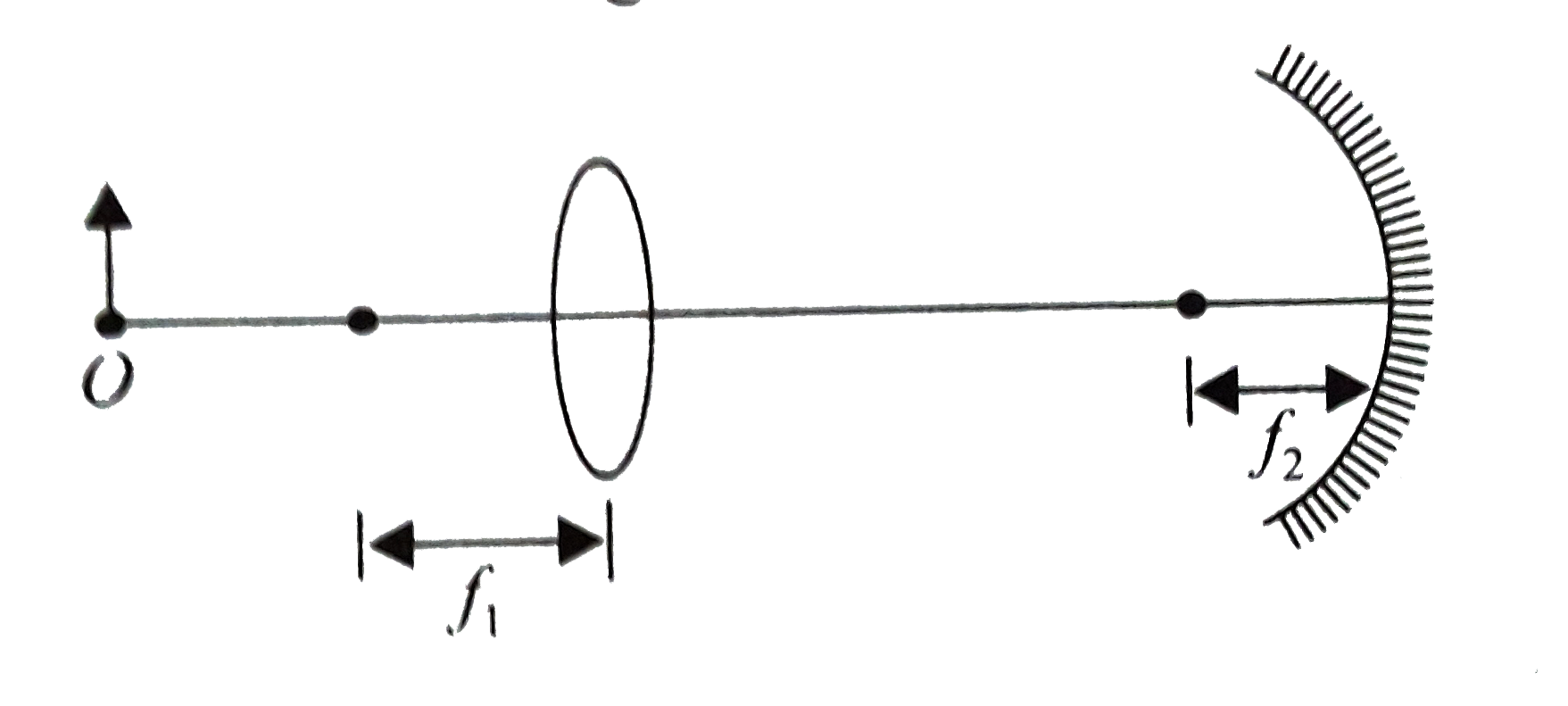
View SolutionAn object is placed in front of a converging lens at a distance equal to twice the focal length f1 of the lens. On the other side of the lens is a concave mirror of focal length f2 separated from the lens is a concave mirror of focal length f2 separated from the lens by a distance 2(f1+f2). Light from the object passes rightward through the lens, reflects from the mirror, passes leftward throught the lens, and forms a final image of the object.
View SolutionA point object is placed on the optic axis of a convex lens of focal length f at a distance of 2f to the left it. The diameter of the lens d. An eye is placed are distance of 3f to the right of the lens and a distance below the optic axis. The maximum value of h to the image is
View Solution
