In case of convex lense, when object is moved from f to 2f, its image is real, inverted and magnified. It moves from infinity to 2f on other side.
Focal len is 10 cm. when the object is moved from 15 cm to 25 cm , the magnitude of liner magnification.
will increases
will decreases
will first increases the decreases
will first decreases then increases
The correct Answer is:B
To solve the problem step by step, we will use the lens formula and the magnification formula. The given focal length of the convex lens is 10 cm, and we need to find the linear magnification when the object is moved from 15 cm to 25 cm.
Step 1: Identify the given values
- Focal length (F) = 10 cm
- Initial object distance (u1) = -15 cm (negative because it is on the same side as the incoming light)
- Final object distance (u2) = -25 cm
Step 2: Use the lens formula to find the image distance (V1) when the object is at u1
The lens formula is given by:
1F=1V−1U
For the first position (u1 = -15 cm):
110=1V1−1−15
Rearranging gives:
1V1=110+115
Finding a common denominator (30):
1V1=330+230=530
Thus,
V1=305=6 cm
Step 3: Calculate the magnification (M1) for the first position
The magnification is given by:
M=VU
For the first position:
M1=V1U1=6−15=−25
Taking the magnitude:
|M1|=25
Step 4: Use the lens formula to find the image distance (V2) when the object is at u2
For the second position (u2 = -25 cm):
110=1V2−1−25
Rearranging gives:
1V2=110+125
Finding a common denominator (50):
1V2=550+250=750
Thus,
V2=507≈7.14 cm
Step 5: Calculate the magnification (M2) for the second position
For the second position:
M2=V2U2=50/7−25=−27
Taking the magnitude:
|M2|=27
Step 6: Compare the magnifications
Now we compare the magnifications:
- Magnification when the object is at 15 cm: |M1| = 2/5 = 0.4
- Magnification when the object is at 25 cm: |M2| = 2/7 ≈ 0.286
Since 0.4>0.286, we can conclude that the magnification decreases as the object moves from 15 cm to 25 cm.
Final Answer
The magnitude of linear magnification will decrease.
---
- Focal length (F) = 10 cm
- Initial object distance (u1) = -15 cm (negative because it is on the same side as the incoming light)
- Final object distance (u2) = -25 cm
The lens formula is given by:
1F=1V−1U
For the first position (u1 = -15 cm):
110=1V1−1−15
Rearranging gives:
1V1=110+115
Finding a common denominator (30):
1V1=330+230=530
Thus,
V1=305=6 cm
The magnification is given by:
M=VU
For the first position:
M1=V1U1=6−15=−25
Taking the magnitude:
|M1|=25
For the second position (u2 = -25 cm):
110=1V2−1−25
Rearranging gives:
1V2=110+125
Finding a common denominator (50):
1V2=550+250=750
Thus,
V2=507≈7.14 cm
For the second position:
M2=V2U2=50/7−25=−27
Taking the magnitude:
|M2|=27
Now we compare the magnifications:
- Magnification when the object is at 15 cm: |M1| = 2/5 = 0.4
- Magnification when the object is at 25 cm: |M2| = 2/7 ≈ 0.286
The magnitude of linear magnification will decrease.
Topper's Solved these Questions
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Matrix matching type Q.
8 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Integer type q.
14 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - B. More than one option is correct
20 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Integer
8 Videos
- ROTATIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - (C) Chapter Exercises
39 Videos
Similar Questions
In case of convex lense, when object is moved from f to 2f, its image is real, inverted and magnified. It moves from infinity to 2f on other side.
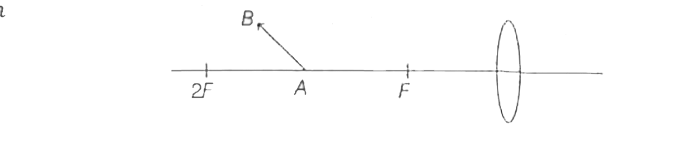
Image of object AB shown in figure will be like
View SolutionA convex lens forms an image of an object placed 20cm away from it at a distance of 20cm on the other side of the lens. If the object is moves 5cm toward the lens, the image will be
View SolutionFind distanace of image from a convex lens of focal length
20cm if object is placed at a distance of 30cm from the lens. Also find its
magnification.
View SolutionA magnifying lens has a focal length of 10 cm. (a) Where should the object be placed if the image is to be 30 cm from the lens ? (b) What will be the magnification ?
View SolutionA dip circle is at right angles to the magnetic meridian. What will be the apparent dip ?
View SolutionA point object is placed at a distance of 10 cm and its real image is formed at a distance of 20 cm from a concave mirror. If the object is moved by 0.1 cm towards the mirror, the image will shift by about
View SolutionIf the distances of an object and its virtual image from the focus of a convex lens of focal length f are 1 cm each, then f is
View SolutionIf the distances of an object and its virtual image from the focus of a convex lens of focal length f are 1 cm each, then f is
View SolutionAn object is placed at 10 cm from a lens and real image is formed with magnification of 0.5. Then the lens is
View SolutionA point object is placed at a distamce of 10 cm anad its real image is formed at a distance of 20 cm from a concave mirror. If the object is moved by 0.1 cm towards the mirror. The image will shift by about
View Solution
