Graph shows magnitude of electric field (E), charge enclosed (qenclosed⟩) within the concentic sphere and net flux (ϕ) through a concentric spherical Gaussian surface as a function of distance (r) from centre of a uniformly positive charged solid sphere of radius R. Choose the correct option (s):




The correct Answer is:B, C
To solve the problem, we need to analyze the relationships between the electric field E, the charge enclosed qenclosed, and the net electric flux Φ through a concentric spherical Gaussian surface surrounding a uniformly positively charged solid sphere of radius R.
1. Understanding Charge Density:
- Let ρ be the volume charge density of the uniformly charged sphere. The total charge Q of the sphere can be expressed as:
Q=ρ⋅43πR3
2. Electric Field Inside the Sphere:
- For a point inside the sphere (where r<R), the charge enclosed qenclosed within a Gaussian surface of radius r is:
qenclosed=ρ⋅43πr3
- According to Gauss's law, the electric field E at a distance r from the center is given by:
E⋅4πr2=qenclosedϵ0
- Substituting for qenclosed:
E⋅4πr2=ρ⋅43πr3ϵ0
- Simplifying gives:
E=ρr3ϵ0
3. Electric Field Outside the Sphere:
- For a point outside the sphere (where r≥R), the charge enclosed is simply the total charge Q:
qenclosed=Q=ρ⋅43πR3
- Again applying Gauss's law:
E⋅4πr2=Qϵ0
- Substituting for Q:
E⋅4πr2=ρ⋅43πR3ϵ0
- This simplifies to:
E=Q4πϵ0r2=ρ⋅43πR34πϵ0r2=ρR33ϵ0r2
4. Net Electric Flux:
- The net electric flux Φ through the Gaussian surface is given by:
Φ=qenclosedϵ0
- For r<R:
Φ=ρ⋅43πr3ϵ0
- For r≥R:
Φ=ρ⋅43πR3ϵ0
5. Graph Analysis:
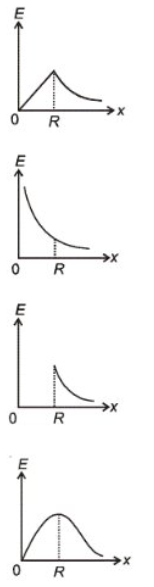
- The electric field E increases linearly with r for r<R and decreases with 1r2 for r≥R.
- The charge enclosed qenclosed increases with r3 for r<R and remains constant for r≥R.
- The net electric flux Φ is constant for r≥R and increases with r3 for r<R.
Conclusion:
Based on the analysis, the correct options for the graphs representing E, qenclosed, and Φ as functions of r are:
- Electric Field E: Increases linearly for r<R and decreases with 1r2 for r≥R.
- Charge Enclosed qenclosed: Increases with r3 for r<R and remains constant for r≥R.
- Net Electric Flux Φ: Increases with r3 for r<R and remains constant for r≥R.
- Let ρ be the volume charge density of the uniformly charged sphere. The total charge Q of the sphere can be expressed as:
Q=ρ⋅43πR3
- For a point inside the sphere (where r<R), the charge enclosed qenclosed within a Gaussian surface of radius r is:
qenclosed=ρ⋅43πr3
- According to Gauss's law, the electric field E at a distance r from the center is given by:
E⋅4πr2=qenclosedϵ0
- Substituting for qenclosed:
E⋅4πr2=ρ⋅43πr3ϵ0
- Simplifying gives:
E=ρr3ϵ0
- For a point outside the sphere (where r≥R), the charge enclosed is simply the total charge Q:
qenclosed=Q=ρ⋅43πR3
- Again applying Gauss's law:
E⋅4πr2=Qϵ0
- Substituting for Q:
E⋅4πr2=ρ⋅43πR3ϵ0
- This simplifies to:
E=Q4πϵ0r2=ρ⋅43πR34πϵ0r2=ρR33ϵ0r2
- The net electric flux Φ through the Gaussian surface is given by:
Φ=qenclosedϵ0
- For r<R:
Φ=ρ⋅43πr3ϵ0
- For r≥R:
Φ=ρ⋅43πR3ϵ0
- The electric field E increases linearly with r for r<R and decreases with 1r2 for r≥R.
- The charge enclosed qenclosed increases with r3 for r<R and remains constant for r≥R.
- The net electric flux Φ is constant for r≥R and increases with r3 for r<R.
Based on the analysis, the correct options for the graphs representing E, qenclosed, and Φ as functions of r are:
- Electric Field E: Increases linearly for r<R and decreases with 1r2 for r≥R.
- Charge Enclosed qenclosed: Increases with r3 for r<R and remains constant for r≥R.
- Net Electric Flux Φ: Increases with r3 for r<R and remains constant for r≥R.
Similar Questions
Flux (ph) as a function of distance (r) from centre of uniformly charged solid sphere of charge Q and radius R, is best represented by
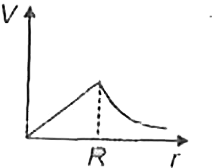
View SolutionWhich graph best represents the variation of electric potential as a function of distance from the centre of a uniformly charged solid sphere of charge of radius R ?
View SolutionPotential at a point x-distance from the centre inside the conducting sphere of radius R and charged with charge Q is
View SolutionThe electrostatic potential of a uniformly charged thin spherical shell of charge Q and radius R at a distance r from the centre
View SolutionDraw a plot showing variation of electric field with distance from the centre of a solid conducting sphere of radius R, having of + Q on its surface.
View SolutionThe electric field due to uniformly charged sphere of radius R as a function of the distance from its centre is represented graphically by
View SolutionA solid sphere of radius R is charged uniformly. The electrostatic potential V is plotted as a function of distance r from the centre of th sphere. Which of the following best represents the resulting curve?
View SolutionAn infinite, uniformly charged sheet with surface charge density σ cuts through a spherical Gaussian surface of radius R at a distance X from its center, as shown in the figure. The electric flux Φ through the Gaussian surface is .

View SolutionA non-conducting solid sphere of radius R, has a uniform charge density. The graph representing variation of magnitude of electric field (E) as a function of distance (x) from the centre of the sphere is
View SolutionIf the potential at the centre of a uniformly charged hollow sphere of radius R is V, then electric field at a distance r from the centre of sphere will be (r>R).

View Solution
