Two stones are thrown up simultaneously from the edge of a cliff with initial speed vand2v. The relative position of the second stone with respect to first varies with time till both the stones strike the ground as.
linearly
first linearly then parabolically
parabolically
first parabolically then linearly
The correct Answer is:B
To solve the problem, we need to analyze the motion of two stones thrown upward from the edge of a cliff with different initial velocities. Let's denote the initial velocity of the first stone as v and that of the second stone as 2v. We will find the relative position of the second stone with respect to the first stone over time until both stones hit the ground.
Step 1: Define the Initial Conditions
- Let the first stone be thrown with an initial velocity v.
- Let the second stone be thrown with an initial velocity 2v.
- Both stones are thrown simultaneously from the same height h.
Step 2: Determine the Relative Position
The relative position of the second stone with respect to the first stone can be expressed as:
s21=s2−s1
where s2 is the position of the second stone and s1 is the position of the first stone.
Step 3: Write the Equations of Motion
For the first stone (with initial velocity v):
s1=h+vt−12gt2
For the second stone (with initial velocity 2v):
s2=h+2vt−12gt2
Step 4: Calculate the Relative Position
Substituting the equations of motion into the relative position equation:
s21=(h+2vt−12gt2)−(h+vt−12gt2)
This simplifies to:
s21=(2vt−vt)=vt
Step 5: Analyze the Motion
- For the upward motion, the relative position s21=vt is linear with respect to time t.
- When both stones reach their maximum height and start falling down, the relative acceleration between the two stones becomes significant.
Step 6: Determine the Downward Motion
During the downward motion, the first stone will have an acceleration of g (as it falls freely after reaching the maximum height), while the second stone will also have an acceleration of g. Thus, the relative position will change as follows:
s21=vt+12gt2
This indicates that the relative position becomes parabolic as both stones fall under gravity.
Conclusion
The relative position of the second stone with respect to the first stone varies first linearly (during the upward motion) and then parabolically (during the downward motion) until both stones strike the ground.
Final Answer
The correct option is: first linearly then parabolically.
- Let the first stone be thrown with an initial velocity v.
- Let the second stone be thrown with an initial velocity 2v.
- Both stones are thrown simultaneously from the same height h.
The relative position of the second stone with respect to the first stone can be expressed as:
s21=s2−s1
where s2 is the position of the second stone and s1 is the position of the first stone.
For the first stone (with initial velocity v):
s1=h+vt−12gt2
For the second stone (with initial velocity 2v):
s2=h+2vt−12gt2
Substituting the equations of motion into the relative position equation:
s21=(h+2vt−12gt2)−(h+vt−12gt2)
This simplifies to:
s21=(2vt−vt)=vt
- For the upward motion, the relative position s21=vt is linear with respect to time t.
- When both stones reach their maximum height and start falling down, the relative acceleration between the two stones becomes significant.
During the downward motion, the first stone will have an acceleration of g (as it falls freely after reaching the maximum height), while the second stone will also have an acceleration of g. Thus, the relative position will change as follows:
s21=vt+12gt2
This indicates that the relative position becomes parabolic as both stones fall under gravity.
The relative position of the second stone with respect to the first stone varies first linearly (during the upward motion) and then parabolically (during the downward motion) until both stones strike the ground.
The correct option is: first linearly then parabolically.
Topper's Solved these Questions
- KINEMATICS 1Book:DC PANDEY ENGLISHChapter:KINEMATICS 1Exercise:MCQ_TYPE
Explore 27 Videos
- KINEMATICS 1Book:DC PANDEY ENGLISHChapter:KINEMATICS 1Exercise:COMPREHENSION_TYPE
Explore 19 Videos
- KINEMATICSBook:DC PANDEY ENGLISHChapter:KINEMATICSExercise:INTEGER_TYPE
Explore 10 Videos
- LAWS OF MOTIONBook:DC PANDEY ENGLISHChapter:LAWS OF MOTIONExercise:Medical entrances gallery
Explore 39 Videos
Similar Questions
Two stones are through up simultaneously from the edge of a cliff 240 m high with initial speed of 10 m/s and 40 m/s respectively. Which of the following graphs best represents the time variation of relative position of the second stone with respect to the first? Assume stones do not rebound after hitting the ground and neglect air resistance, take . g=10m/s2 (The figures are schematic and not drawn to scale)
View SolutionTwo stones are thrown vertically upwards simultaneously from the same point on the ground with initial speed u1=30m/sec and u2=50m/sec. Which of the curve represent correct variation (for the time interval in which both reach the ground) of
(x2−x1) = the relative position of second stone with respect to first with time (t)
(v2−v1) = the relative velocity of second stone with respect to first with time (t).
Assuming that stones do not rebound after hitting.
View Solution
Knowledge Check
Two stones are thrown up simultaneously from the edge of a cliff 200 m high with initial speeds of 15ms−1 and 30ms−1 respectively. The time variation of the relative position of the second stone with respect to the first is shown in the figure. The equation of the linear part is
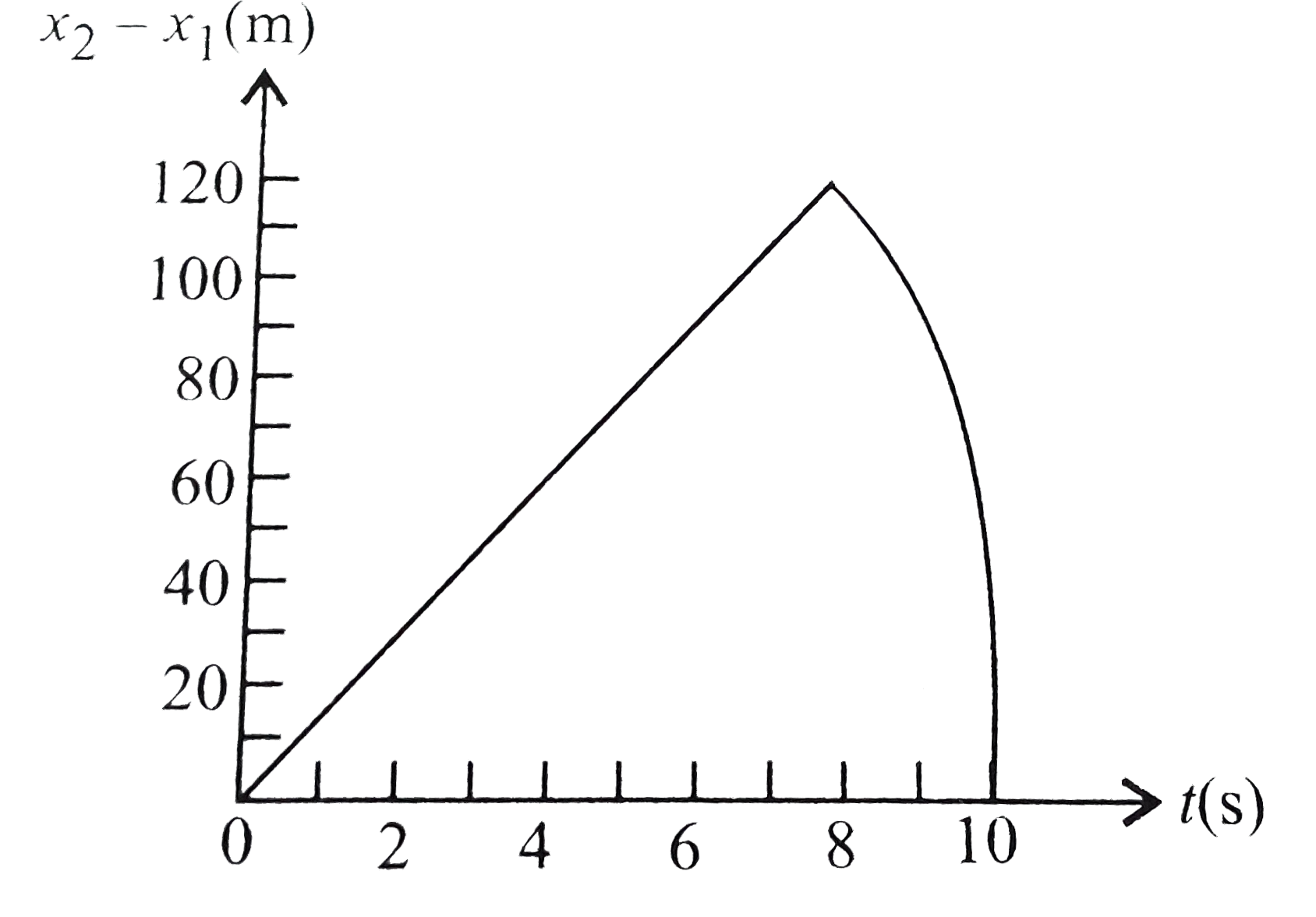 Ax2−x1 = 50tBx2−x1 = 10tCx2−x1 = 15tDx2−x1 = 20t
Ax2−x1 = 50tBx2−x1 = 10tCx2−x1 = 15tDx2−x1 = 20t
One stone is dropped from a tower from rest and simultaneously another stone is projected vertically upwards from the tower with some initial velocity. The graph of distance (s) between the two stones varies with time (t) as (before either stone hits the ground).
View SolutionTwo stones are projected simultaneously from a tower a differenct angles of projection with same speed 'u'. The distance between two stones is increasing at constant rate 'u'. Then the angle between the initial velocity vectors of the two stones is :
View SolutionA stone A is dropped from rest from a height h above the ground.A second stone B is simultaneously thrown vertically up from a point on the ground with velocity v.The line of motion of both the stones is same .Find if the value of v which would enable the stone B to meet the stone A midway between their initial positions =

View SolutionA heavy stone is thrown from a cliff of height h with a speed v. The stone will hit the ground with maximum speed if it is thrown
View SolutionA man standing on the edge of a cliff throws a stone straight up with initial speed (u) and then throws another stone straight down with same initial speed and from the same position. Find the ratio of the speeds. The stones would have attained when they hit ground at the base of the cliff.
View SolutionA stone falls freely rest. The distance covered by it in the last second is equal to the distance covered by it in the first 2 s. The time taken by the stone to reach the ground is
View SolutionAssertion:- Two stones are simultaneously projected from level ground from same point with same speeds but different angles with horizontal Both stones move in same vertical plane. Then the two stones may collide in mid air.
Reason:- For two stones projected simultaneously from same point with same speed at different angles with horizontal, their trajectories must intersect at some point except projection point.
View Solution