A group of identical cells (all in parallel) are connected to an external resistance R1. Current through R1 is i1. Another group of identical cells (all in series) are connected to some other resistance R2. Current through R2 is i2. Now one cell is removed from both the groups. Then
i1 will decrease
i2 may decrease or increase depending on the value E and r
both (a) and (b) are correct
both (a) and (b) wrong
The correct Answer is:A
To solve the problem, we need to analyze the situation before and after removing one cell from both groups of cells connected to resistances R1 and R2.
1. Identify the Initial Conditions:
- Let n be the number of identical cells in the parallel group connected to R1.
- Let n′ be the number of identical cells in the series group connected to R2.
- The EMF of each cell is E and the internal resistance of each cell is r.
2. Calculate the Equivalent EMF and Resistance for the Parallel Group:
- For n cells in parallel, the equivalent EMF Eeq1 remains E.
- The equivalent internal resistance Req1 for n cells in parallel is given by:
Req1=rn
- The total resistance in the circuit is R1+Req1=R1+rn.
- The current i1 through R1 can be expressed using Ohm's law:
i1=ER1+rn
3. Calculate the Equivalent EMF and Resistance for the Series Group:
- For n′ cells in series, the equivalent EMF Eeq2 is:
Eeq2=n′E
- The equivalent internal resistance Req2 for n′ cells in series is:
Req2=n′r
- The total resistance in the circuit is R2+Req2=R2+n′r.
- The current i2 through R2 can be expressed as:
i2=n′ER2+n′r
4. Removing One Cell from Each Group:
- After removing one cell from the parallel group, the number of cells becomes n−1:
- New equivalent internal resistance:
R′eq1=rn−1
- New current i′1:
i′1=ER1+rn−1
- After removing one cell from the series group, the number of cells becomes n′−1:
- New equivalent internal resistance:
R′eq2=(n′−1)r
- New current i′2:
i′2=(n′−1)ER2+(n′−1)r
5. Analyze the Effect of Removing One Cell:
- In both cases, removing one cell increases the equivalent internal resistance, which in turn decreases the current through the respective resistances:
- For the parallel group:
i′1<i1
- For the series group:
i′2<i2
- Let n be the number of identical cells in the parallel group connected to R1.
- Let n′ be the number of identical cells in the series group connected to R2.
- The EMF of each cell is E and the internal resistance of each cell is r.
- For n cells in parallel, the equivalent EMF Eeq1 remains E.
- The equivalent internal resistance Req1 for n cells in parallel is given by:
Req1=rn
- The total resistance in the circuit is R1+Req1=R1+rn.
- The current i1 through R1 can be expressed using Ohm's law:
i1=ER1+rn
- For n′ cells in series, the equivalent EMF Eeq2 is:
Eeq2=n′E
- The equivalent internal resistance Req2 for n′ cells in series is:
Req2=n′r
- The total resistance in the circuit is R2+Req2=R2+n′r.
- The current i2 through R2 can be expressed as:
i2=n′ER2+n′r
- After removing one cell from the parallel group, the number of cells becomes n−1:
- New equivalent internal resistance:
R′eq1=rn−1
- New current i′1:
i′1=ER1+rn−1
- After removing one cell from the series group, the number of cells becomes n′−1:
- New equivalent internal resistance:
R′eq2=(n′−1)r
- New current i′2:
i′2=(n′−1)ER2+(n′−1)r
- In both cases, removing one cell increases the equivalent internal resistance, which in turn decreases the current through the respective resistances:
- For the parallel group:
i′1<i1
- For the series group:
i′2<i2
Topper's Solved these Questions
Similar Questions
When a celll is connected to an external resistance R1, current through it is i1. When the same cell is connected to an external resistance R2, current through it is i2. Calculate the emf and internal resisance of the cell.
View SolutionA cell supplies a current i1 trhough a resistnace R1 and a current i2 through a resistance R2. The internal resistance of this cell is
View Solution
Knowledge Check
In a circuit a cell with internal resistance r is connected to an external resistance R. The condition for the maximum current that drawn from the cell is
AR=rBR<rCR>rDR=0
N identical cells whether joined together in series or in parallel, give the same current, when connected to an external resistance of 'R'. The internal resistance of each cell is:-
View SolutionN cells each of e.m.f. E & internal resistance r are grouped into sets of K cells connected in series. The (N/K) sets ae connected in parallel to a load of resistance R, then,
View SolutionTwo cells of the same e.mf. e but different internal resistances r1andr2 are connected in series with an external resistance 'R'. The potential drop across the first cell is found to be zero. The external resistance Ris
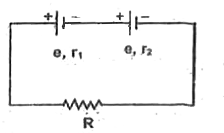
View SolutionWhen two identical cells are connected either in series or in parallel across a 4 ohm resistor, they send the same current through it. The internal resistance of the cell in ohm is
View SolutionA group of six identical cells each of emf E and internal resistance r are joined in series to form a loop. The terminal voltage across each cells is
View SolutionA cell of emf ε and internal resistance r is charged by a current I, then
View Solutionn identical cells each of emf 6 V , connected in series with an external resistor of 5Ω, carry a current of 10A. If two cells are connected wrongly in series with the same external resistor, the current flowing through the cells will be 8A. Find the value of n and r the internal resistance of each cell.
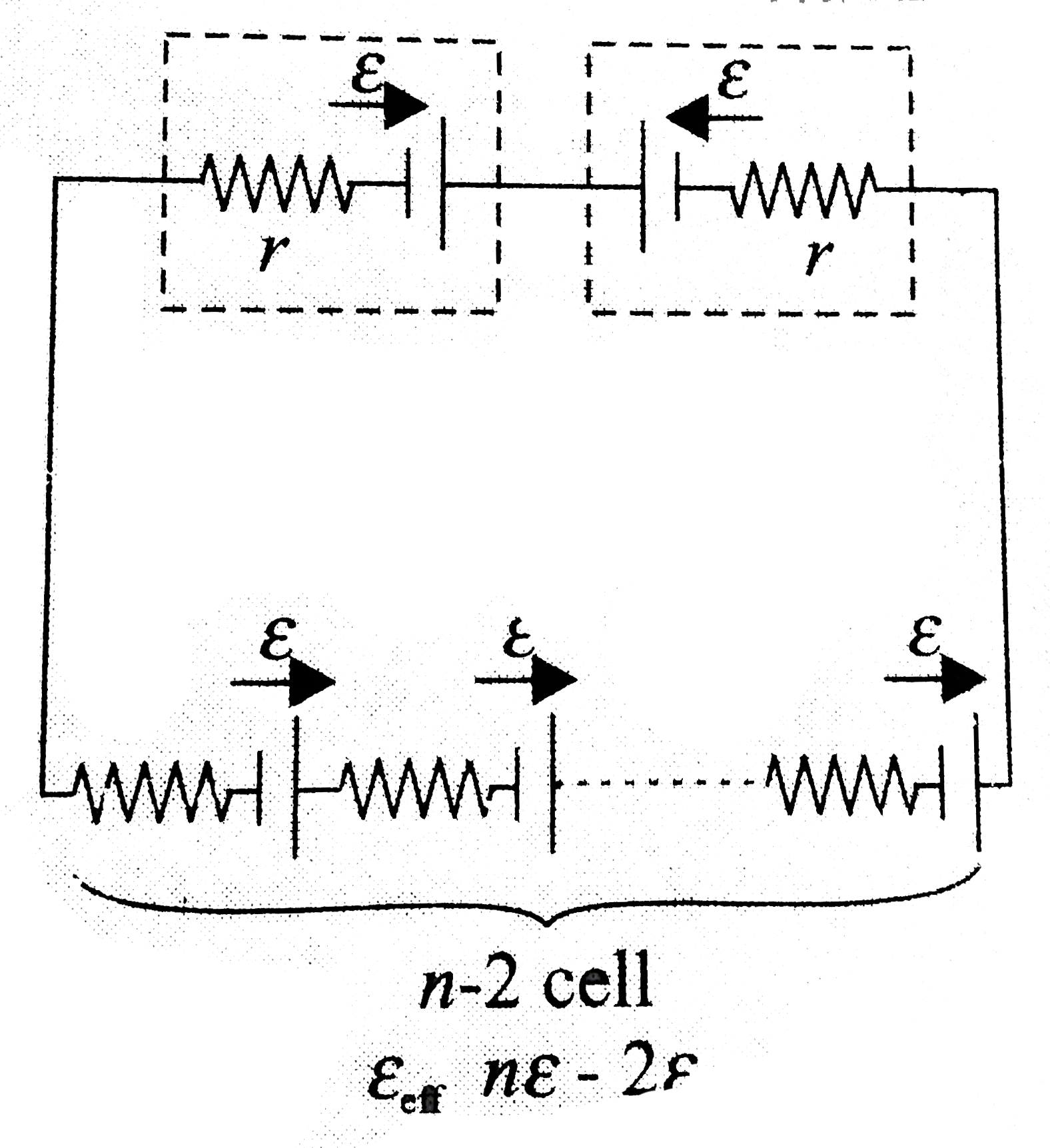
View Solution