Five identical cells are connected in parallel. Now, polarity of one of the cells is reversed. Percentage change in equivalent emf will be
-0.1
-0.2
-0.4
-0.6
The correct Answer is:C
To solve the problem of finding the percentage change in equivalent EMF when one of the five identical cells connected in parallel has its polarity reversed, we can follow these steps:
1. Understanding the Initial Configuration:
- We have five identical cells connected in parallel.
- Let the EMF of each cell be E and the internal resistance of each cell be r.
2. Calculating the Equivalent EMF for All Cells in Parallel:
- When all five cells are connected in parallel, the equivalent EMF (Einitial) is given by:
Einitial=E
- This is because in parallel, the EMF remains the same as that of one cell.
3. Reversing the Polarity of One Cell:
- When one cell's polarity is reversed, we effectively have four cells contributing positively and one cell contributing negatively.
- The equivalent EMF (Efinal) can be calculated as:
Efinal=E+E+E+E−E=4E−E=3E
- Since they are in parallel, we can express the equivalent EMF as:
Efinal=3E5
4. Calculating the Percentage Change in EMF:
- The percentage change in equivalent EMF can be calculated using the formula:
Percentage Change=Efinal−EinitialEinitial×100
- Substituting the values:
Percentage Change=3E5−EE×100
- Simplifying the equation:
=3E5−5E5E×100=−2E5E×100=−40%
5. Conclusion:
- The percentage change in equivalent EMF when one of the cells has its polarity reversed is −40%. The negative sign indicates a decrease in EMF.
- We have five identical cells connected in parallel.
- Let the EMF of each cell be E and the internal resistance of each cell be r.
- When all five cells are connected in parallel, the equivalent EMF (Einitial) is given by:
Einitial=E
- This is because in parallel, the EMF remains the same as that of one cell.
- When one cell's polarity is reversed, we effectively have four cells contributing positively and one cell contributing negatively.
- The equivalent EMF (Efinal) can be calculated as:
Efinal=E+E+E+E−E=4E−E=3E
- Since they are in parallel, we can express the equivalent EMF as:
Efinal=3E5
- The percentage change in equivalent EMF can be calculated using the formula:
Percentage Change=Efinal−EinitialEinitial×100
- Substituting the values:
Percentage Change=3E5−EE×100
- Simplifying the equation:
=3E5−5E5E×100=−2E5E×100=−40%
- The percentage change in equivalent EMF when one of the cells has its polarity reversed is −40%. The negative sign indicates a decrease in EMF.
Topper's Solved these Questions
Similar Questions
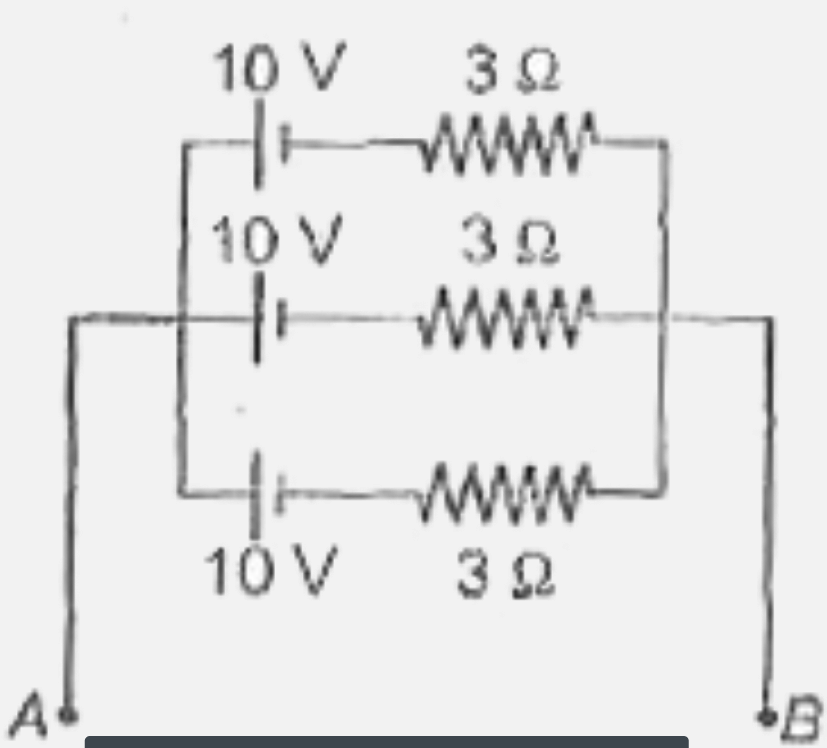
Three identical cells are connected in parallel across AB. Net across AB is
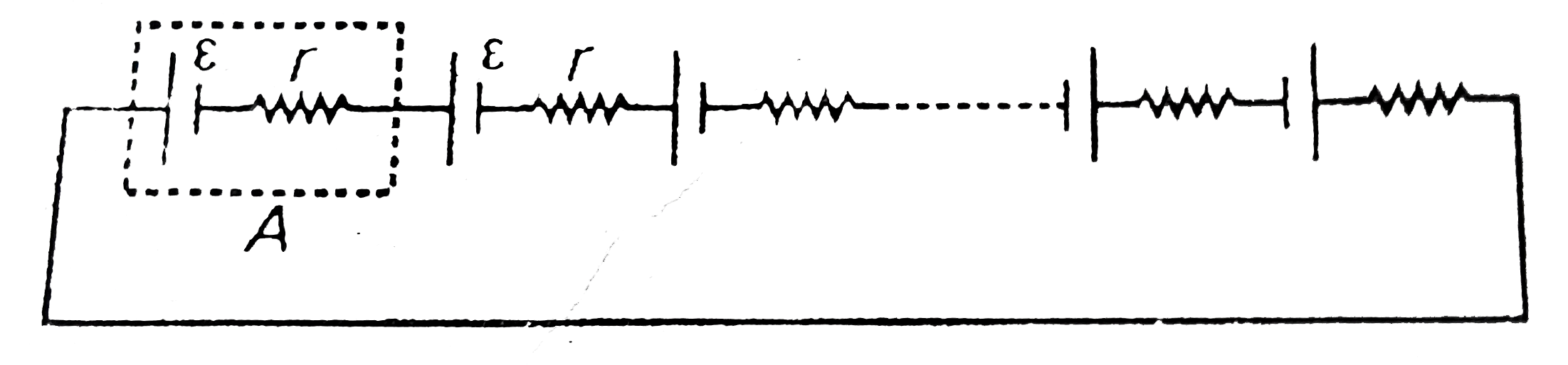
View SolutionIn a arrangement, 3n cells of emf ε and internal resistance r are connected in series. Out of 3n cells polarity of n cells is reversed. Current in the circuit is
View SolutionFour identical cells of emf ε and internal resistance r are to be connected in series. Suppose, if one of the cell is connected wrongly, then the equivalent emf and effective internal resistance of the combination is
View SolutionTwo cells when connected in series are balanced on 8m on a potentiometer. If cells are connected with polarities of one the cellis reversed, they balance on 2m. The ratio of e.m.f.'s of the two cellsis
View SolutionFive cells each of emf E and internal resistance r are connecte in series. Due to oversight one cell is connected wrongly . The equivalent emf and internal resistance of the combination is
View SolutionAssertion : Two non-ideal batteries are connected in parallel with same polarities on same: side. The equivalent emf is smaller than either of the two emfs.
Reason : Two non-ideal batteries are connected in parallel, the equivalent inter resistance is smaller than either of the two internal resistances.
View SolutionTwo cells of same emf but different internal resistance are connected in parallel so as to send current in same direction.
STATEMENT-1 : The equivalent emf of the combination is equal to individual emf of each cell.
and
STATEMENT-2 : The equivalent emf is the arithmetic mean of the individual emfs.
View SolutionTwo cells of emfs 1.5 V and 2.0v having internal resistance 0.2Σ and 0.3 Σ respectively are connected in parallel . Calculate the emf and internal resistance of the equivalent cell .
View SolutionTwo nonideal batteries are connected in parallel. Consider the following statements:
(A)The equivalent emf is smaller than either of the two emfs.
(B) The equivalent internal resistance is smaller than either of the two internal resistances.
View SolutionTwo nonideal batteries are connected in parallel. Consider the following statements:
(A)The equivalent emf is smaller than either of the two emfs.
(B) The equivalent internal resistance is smaller than either of the two internal resistances.
View Solution
