A body of mass m is thrown upwards at an angle θ with the horizontal with velocity v. While rising up the velocity of the mass after t second will be
√(vcosθ2)+(vsinθ)2
√(vcosθ−vsinθ)2−gt
√v2+g2t2−(2bsinθ)gt
√v2+g2t2−(2vcosθ)gt
The correct Answer is:C
To find the velocity of a body of mass m thrown upwards at an angle θ with an initial velocity v after t seconds, we can break down the problem into the horizontal and vertical components of the motion.
1. Identify the Components of Initial Velocity:
- The initial velocity v can be resolved into two components:
- Horizontal component: vx=vcosθ
- Vertical component: vy=vsinθ
2. Determine the Horizontal Velocity:
- The horizontal motion has no acceleration (assuming air resistance is negligible), so the horizontal component of velocity remains constant:
vx=vcosθ
3. Determine the Vertical Velocity After t Seconds:
- The vertical motion is affected by gravity. The acceleration due to gravity g acts downward, which we take as negative:
- The vertical velocity after t seconds can be calculated using the kinematic equation:
vy=uy+at
where uy=vsinθ (initial vertical velocity), a=−g (acceleration due to gravity), and t is the time.
- Substituting the values:
vy=vsinθ−gt
4. Calculate the Magnitude of the Resultant Velocity:
- The resultant velocity v can be found using the Pythagorean theorem since the horizontal and vertical components are perpendicular to each other:
v=√v2x+v2y
- Substituting the expressions for vx and vy:
v=√(vcosθ)2+(vsinθ−gt)2
5. Simplify the Expression:
- Expanding the squares:
v=√v2cos2θ+(vsinθ−gt)2
- This expands to:
v=√v2cos2θ+(v2sin2θ−2vsinθ⋅gt+g2t2)
- Combine v2cos2θ and v2sin2θ:
v=√v2(cos2θ+sin2θ)+g2t2−2vsinθ⋅gt
- Using the identity cos2θ+sin2θ=1:
v=√v2+g2t2−2vsinθ⋅gt
Final Result:
The final velocity of the mass after t seconds is given by:
v=√v2+g2t2−2vgtsinθ
- The initial velocity v can be resolved into two components:
- Horizontal component: vx=vcosθ
- Vertical component: vy=vsinθ
- The horizontal motion has no acceleration (assuming air resistance is negligible), so the horizontal component of velocity remains constant:
vx=vcosθ
- The vertical motion is affected by gravity. The acceleration due to gravity g acts downward, which we take as negative:
- The vertical velocity after t seconds can be calculated using the kinematic equation:
vy=uy+at
where uy=vsinθ (initial vertical velocity), a=−g (acceleration due to gravity), and t is the time.
- Substituting the values:
vy=vsinθ−gt
- The resultant velocity v can be found using the Pythagorean theorem since the horizontal and vertical components are perpendicular to each other:
v=√v2x+v2y
- Substituting the expressions for vx and vy:
v=√(vcosθ)2+(vsinθ−gt)2
- Expanding the squares:
v=√v2cos2θ+(vsinθ−gt)2
- This expands to:
v=√v2cos2θ+(v2sin2θ−2vsinθ⋅gt+g2t2)
- Combine v2cos2θ and v2sin2θ:
v=√v2(cos2θ+sin2θ)+g2t2−2vsinθ⋅gt
- Using the identity cos2θ+sin2θ=1:
v=√v2+g2t2−2vsinθ⋅gt
The final velocity of the mass after t seconds is given by:
v=√v2+g2t2−2vgtsinθ
Topper's Solved these Questions
- MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - MOTIONEXERCISE - B. Medical entrance
1 Video
- MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - MOTIONEXERCISE - Medical entrance
12 Videos
- MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - MOTIONEXERCISE - A. Taking it together
1 Video
- MEASUREMENT AND ERRORSBOOK - DC PANDEY ENGLISHCHAPTER - MEASUREMENT AND ERRORSEXERCISE - Subjective
19 Videos
- MOTION IN A PLANEBOOK - DC PANDEY ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - (C )Medical entrances gallery
32 Videos
Similar Questions
Knowledge Check
A ball of mass M is thrown vertically upwards. Another ball of mass 2M is thrown at an angle θ with the vertical. Both of them stay in air for the same period of time. The heights attained by the two are in the ratio
A1:2B2:1C1:1D1:cosθ
A body of mass m is projected with a velocity u at an angle θ with the horizontal. The angular momentum of the body, about the point of projection, when it at highest point on its trajectory is
View SolutionA particle (a mud pallet, say) of mass m strikes a smooth stationary wedge of mass M with as velocity v0 at an angle θ with horizontal. If the collision is perfectly inelastic, find the
a. velocity of the wedge just after the collision.
b. Change in KE of the system (M+m) in collision.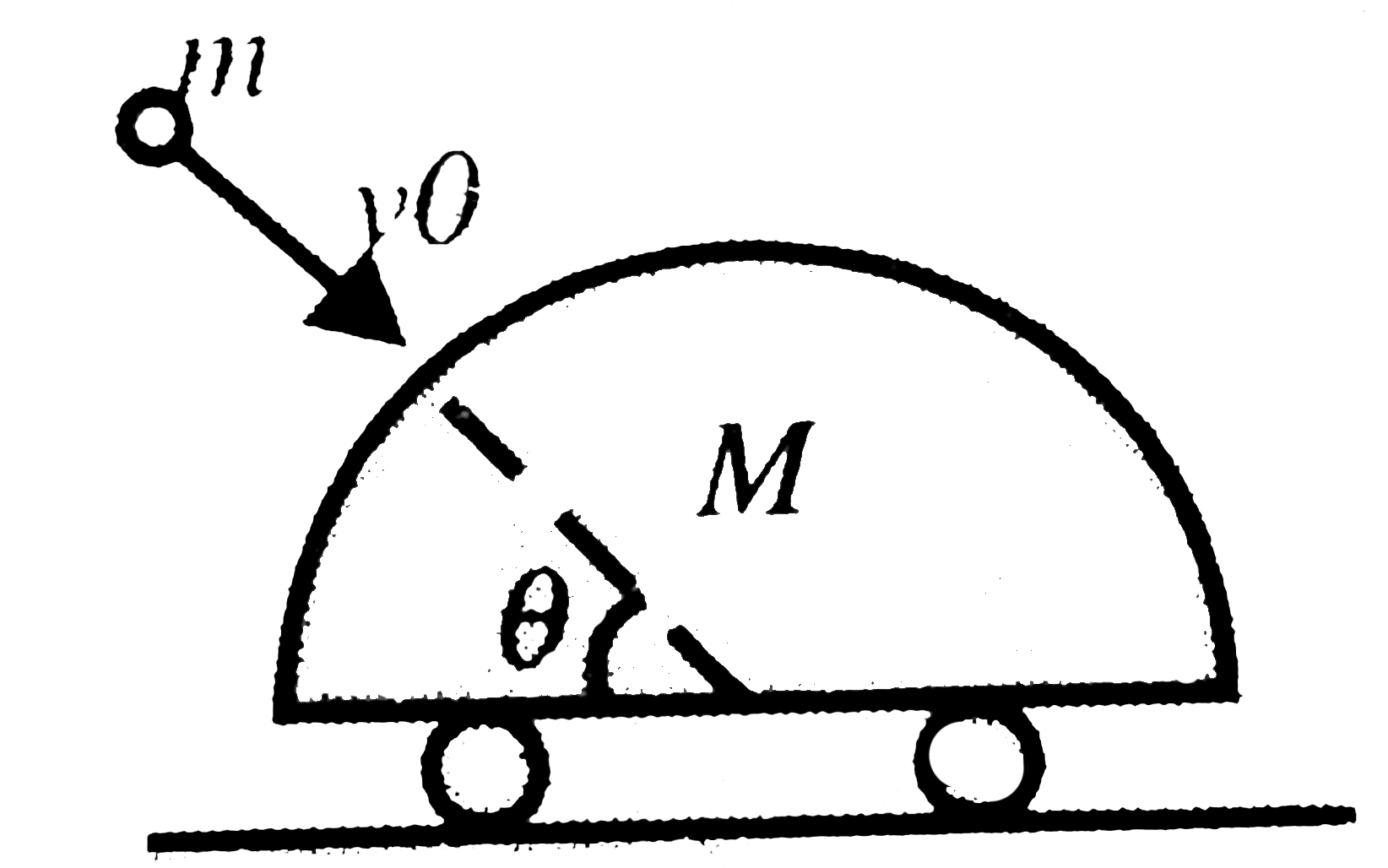
View SolutionA particle of mass 1 kg is projected at an angle of 30∘ with horizontal with velocity v = 40 m/s . The change in linear momentum of the particle after time t = 1 s will be (g = 10 m/s2)
View SolutionA solid cylinder of mass M and radius R rolls from rest down a plane inclined at an angle θ to the horizontal. The velocity of the centre of mass of the cylinder after it has rolled down a distance d is :
View SolutionA ball is thrown up with a certain velocity at angle θ to the horizontal. The kinetic energy varies with height h of the particle as:
View SolutionA body is projected with velocity u at an angle of projection θ with the horizontal. The direction of velocity of the body makes angle 30∘ with the horizontal at t=2s and then after 1s it reaches the maximum height. Then
View SolutionIf a body of mass m collides head on, elastically with velocity u with another identical boday at rest. After collision velocty of the second body will be
View Solution
