A packet is dropped from a helicopter rising up with velocity 2ms−1. The velocity of the packet after 2 seconds will be
18 m/s
10 m/s
8 m/s
2 m/s
The correct Answer is:A
To solve the problem, we need to determine the velocity of the packet after it is dropped from the helicopter. Here’s a step-by-step solution:
Step 1: Identify the initial conditions
- The helicopter is rising with a velocity of u=2m/s (upward).
- The packet is dropped from the helicopter, so its initial velocity is also u=2m/s (upward).
- The acceleration due to gravity is g=10m/s2 (downward).
Step 2: Set the direction
- We will take the upward direction as positive. Hence, the acceleration due to gravity will be negative: a=−g=−10m/s2.
Step 3: Use the kinematic equation
We will use the kinematic equation:
v=u+at
Where:
- v is the final velocity,
- u is the initial velocity,
- a is the acceleration,
- t is the time.
Step 4: Substitute the values
Substituting the known values into the equation:
v=2m/s+(−10m/s2)(2s)
v=2m/s−20m/s
v=−18m/s
Step 5: Interpret the result
The negative sign indicates that the velocity is directed downward. Therefore, the magnitude of the velocity is 18m/s and the direction is downward.
Final Answer
The velocity of the packet after 2 seconds is 18m/s downward.
---
- The helicopter is rising with a velocity of u=2m/s (upward).
- The packet is dropped from the helicopter, so its initial velocity is also u=2m/s (upward).
- The acceleration due to gravity is g=10m/s2 (downward).
- We will take the upward direction as positive. Hence, the acceleration due to gravity will be negative: a=−g=−10m/s2.
We will use the kinematic equation:
v=u+at
Where:
- v is the final velocity,
- u is the initial velocity,
- a is the acceleration,
- t is the time.
Substituting the known values into the equation:
v=2m/s+(−10m/s2)(2s)
v=2m/s−20m/s
v=−18m/s
The negative sign indicates that the velocity is directed downward. Therefore, the magnitude of the velocity is 18m/s and the direction is downward.
The velocity of the packet after 2 seconds is 18m/s downward.
Topper's Solved these Questions
Similar Questions
A packet is dropped from a balloon rising up with uniform velocity 9.8ms−1. If the balloon is at a height of 39.2 m from the ground at the time of dropping the stone, the stone reaches the ground after
View SolutionA helicopter rises from rest on the ground vertically upwards with a constant acceleration g. A food packet is dropped from the helicopter when is at a height h. The time taken by the packet to reach the ground is close to [ g is the acceeration due to gravity] :
View SolutionA body of mass m is thrown upwards at an angle θ with the horizontal with velocity v. While rising up the velocity of the mass after t second will be
View SolutionA body is projected horizontally from a height of 78.4 m with a velocity 10 ms−1 . Its velocity after 3 seconds is [g = 10 ms−2] (Take direction of projection on →i and vertically upward direction on j)
View SolutionA body is dropped from the top of the tower and falls freely.
The velocity of the body after n seconds is proportional to .
View SolutionA body is projected horizontally from the top of a tower with a velocity of 30 m/s. The velocity of the body 4 seconds after projection is (g = 10ms−2)
View SolutionHeight of the body from the ground can be calculated by using the formula h=−ut+(1/2)(gt2) in
(a) A body projected vertically with velocity 'u' from the top of tower, reaches the ground in 't' sec.
( b) A body dropped from a balloon moving up with uniform velocity, reaches the ground in 't' sec
(c) A body dropped from a helicopter moving up with uniform velocity, reaches the ground in 't' sec
(d) A body projected vertically from the ground reaches the ground in 't' sec.
View SolutionA helicopter is flying at 200m and flying at 25ms−1 at an angle 37∘ above the horizontal when a package is dropped from it.
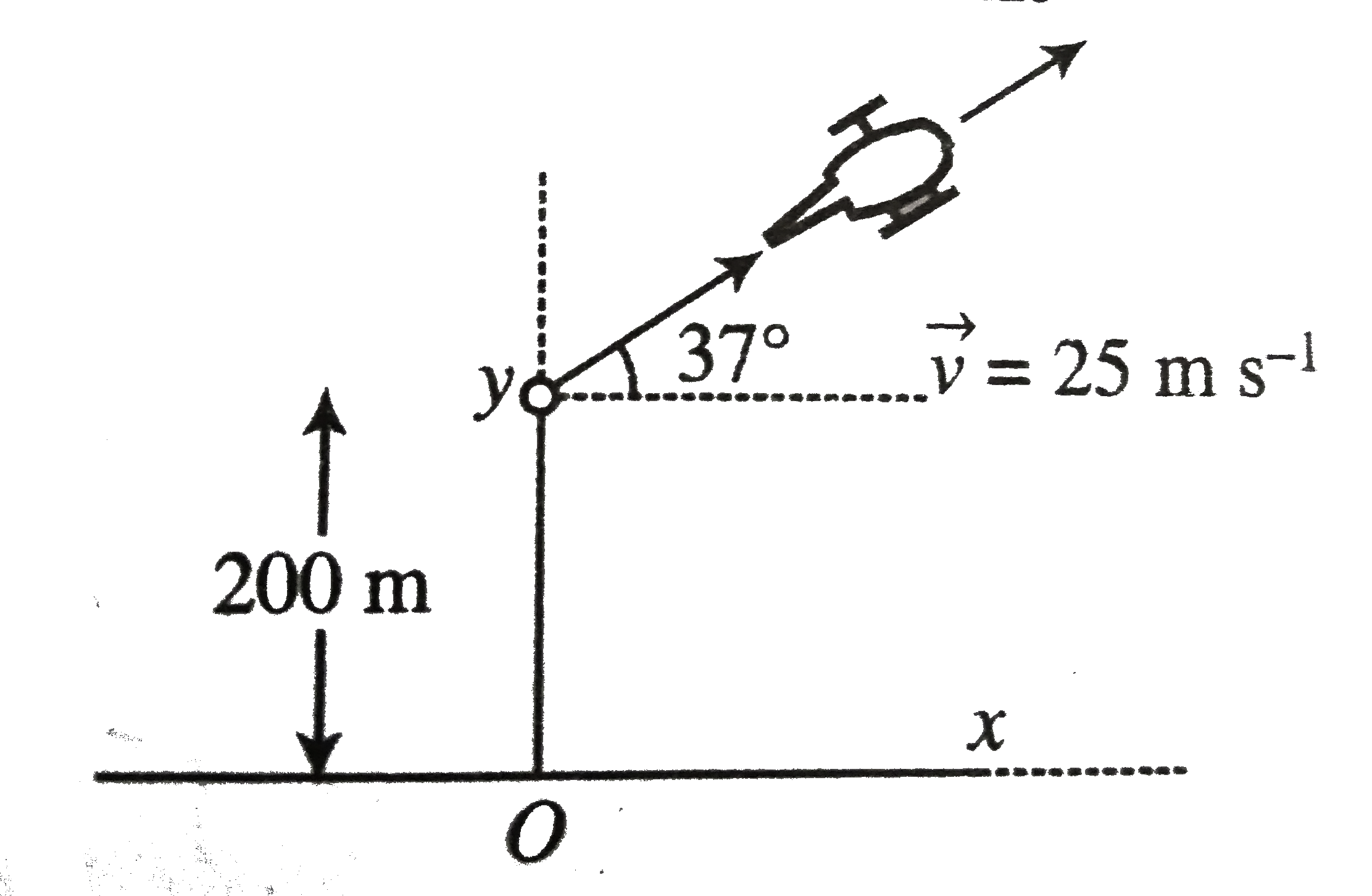
If the helicopter flies at constant velocity, find the x and y coordinates of the location of the helicopter when the package lands.
View SolutionIn the above question, the value of its angular velocity after 2 seconds will be:
View SolutionA food packet is dropped from a plane going at an altitude of 100 m. What is the path of the packet as seen from the plane? What is the path as seen from the ground? If someone asks what is the actual path, what will you answer?
View Solution
