Two particles are simultaneously projected in opposite directions horizontally from a given point in space where gravity g is uniform. If u1 and u2 be their initial speeds, then the time t after which their velocitites are mutually perpendicular is given by
√u1u2g
√u21+u22g
√u1(u1+u2)g
√u2(u1+u2)g
The correct Answer is:A
To solve the problem of finding the time t after which the velocities of two particles projected in opposite directions are mutually perpendicular, we can follow these steps:
Step 1: Understand the Motion of the Particles
Two particles are projected horizontally from a point with initial speeds u1 and u2 in opposite directions. Due to gravity, both particles will experience vertical acceleration downwards.
Step 2: Determine the Velocity Components
For each particle, we can break down the velocity into horizontal and vertical components:
- Particle 1 (projected with speed u1):
- Horizontal component: v1x=−u1 (to the left)
- Vertical component: v1y=gt (downwards, starting from rest)
- Particle 2 (projected with speed u2):
- Horizontal component: v2x=u2 (to the right)
- Vertical component: v2y=gt (downwards, starting from rest)
Step 3: Write the Velocity Vectors
The velocity vectors for the two particles can be expressed as:
- →v1=−u1^i+gt^j
- →v2=u2^i+gt^j
Step 4: Condition for Perpendicular Velocities
The velocities are mutually perpendicular when their dot product is zero:
→v1⋅→v2=0
Calculating the dot product:
(−u1^i+gt^j)⋅(u2^i+gt^j)=−u1u2+(gt)(gt)=0
Step 5: Simplify the Equation
From the dot product equation, we have:
−u1u2+g2t2=0
Rearranging gives:
g2t2=u1u2
Step 6: Solve for Time t
Taking the square root of both sides, we find:
t2=u1u2g2
Thus,
t=√u1u2g
Conclusion
The time t after which the velocities of the two particles are mutually perpendicular is given by:
t=√u1u2g
Two particles are projected horizontally from a point with initial speeds u1 and u2 in opposite directions. Due to gravity, both particles will experience vertical acceleration downwards.
For each particle, we can break down the velocity into horizontal and vertical components:
- Horizontal component: v1x=−u1 (to the left)
- Vertical component: v1y=gt (downwards, starting from rest)
- Horizontal component: v2x=u2 (to the right)
- Vertical component: v2y=gt (downwards, starting from rest)
The velocity vectors for the two particles can be expressed as:
- →v1=−u1^i+gt^j
- →v2=u2^i+gt^j
The velocities are mutually perpendicular when their dot product is zero:
→v1⋅→v2=0
(−u1^i+gt^j)⋅(u2^i+gt^j)=−u1u2+(gt)(gt)=0
From the dot product equation, we have:
−u1u2+g2t2=0
Rearranging gives:
g2t2=u1u2
Taking the square root of both sides, we find:
t2=u1u2g2
Thus,
t=√u1u2g
The time t after which the velocities of the two particles are mutually perpendicular is given by:
t=√u1u2g
Topper's Solved these Questions
- MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - MOTIONEXERCISE - B. Medical entrance
1 Videos
- MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - MOTIONEXERCISE - Medical entrance
12 Videos
- MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - MOTIONEXERCISE - A. Taking it together
1 Videos
- MEASUREMENT AND ERRORSBOOK - DC PANDEY ENGLISHCHAPTER - MEASUREMENT AND ERRORSEXERCISE - Subjective
19 Videos
- MOTION IN A PLANEBOOK - DC PANDEY ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - (C )Medical entrances gallery
32 Videos
Similar Questions
Two particles are thrown horizontally in opposite directions with velocities u and 2u from the top of a high tower. The time after which their radius of curvature will be mutually perpendicular is
View SolutionTwo particles are simultaneously projected in the same vertical plane from the same point with velocities u and v at angles αandβ with horizontal. Find the time that elapses when their velocities are parallel.
View SolutionTwo particles are projected from a tower horizontally in opposite directions with velocities 10m/sand20m/s. Find the time when their velocity vectors are mutually perpendicular. Take g=10m/s2.
View SolutionA particle is projected with speed u at angle θ with horizontal from ground . If it is at same height from ground at time t1andt2 , then its average velocity in time interval t1 to t2 is
View SolutionA particle of mass m is projected with speed u at angle θ with horizontal from ground. The work done by gravity on it during its upward motion is
View SolutionTwo particles are separated at a horizontal distance x as shown in (Fig. 5.57). They are projected at the same time as shown in the figure with different initial speed. Find the time after which the horizontal distance between the particles becomes zero.
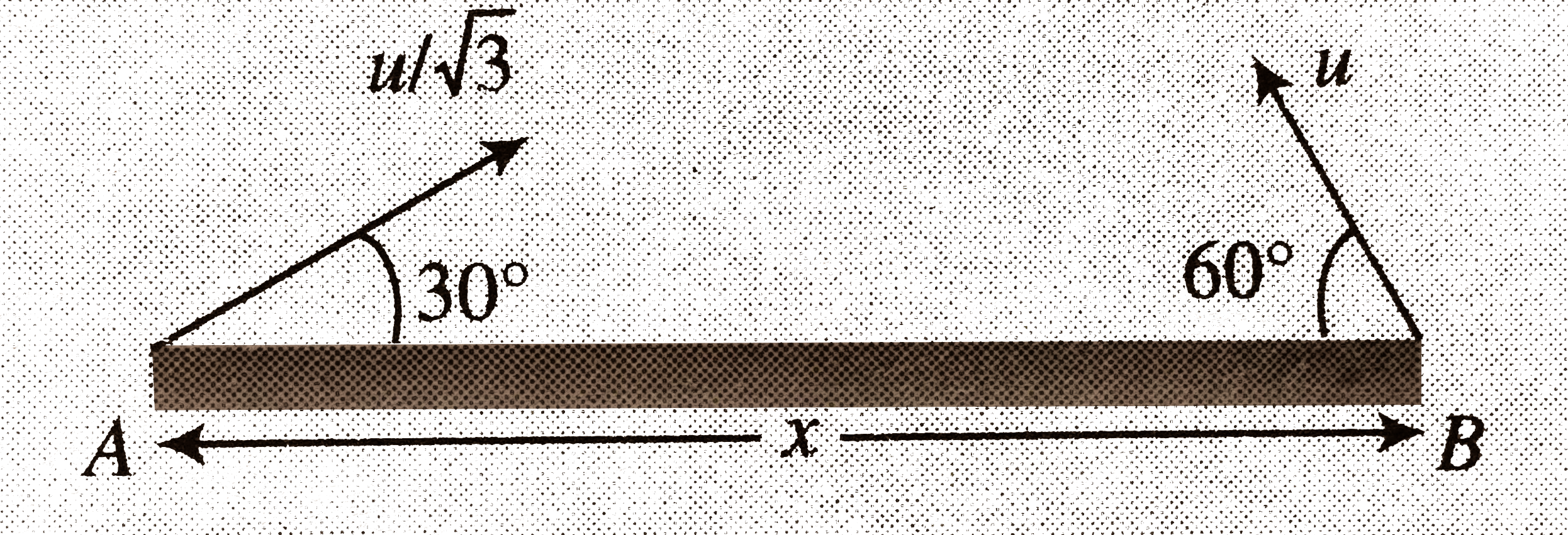 .
.
View SolutionTwo particles are projected with speeds u1 and u2 in mutually opposite directions from top of a tower horizontally. Find the time after which their velocity becomes perpendicular to each other.
View SolutionTwo particles move in a uniform gravitational field with an acceleration g. At the intial moment the particles were located at one point and moved with velocity v1=1 ms−1 and v2=4 ms−1 horizontally in opposite directions. Find the time interval after their velocity vectors become mutually perpendicular
View SolutionA particle A is projected with an initial velocity of 60m/s at an angle 30∘ to the horizontal. At the same time a second particle B is projected in opposite direction with initial speed of 50m/s from a point at a distance of 100 m from A. If the particles collide in air, find (a)the angle of projection α of particle B, (b) time when the collision takes place and (c) the distance of P from A, where collision occurs. (g=10m/s2)
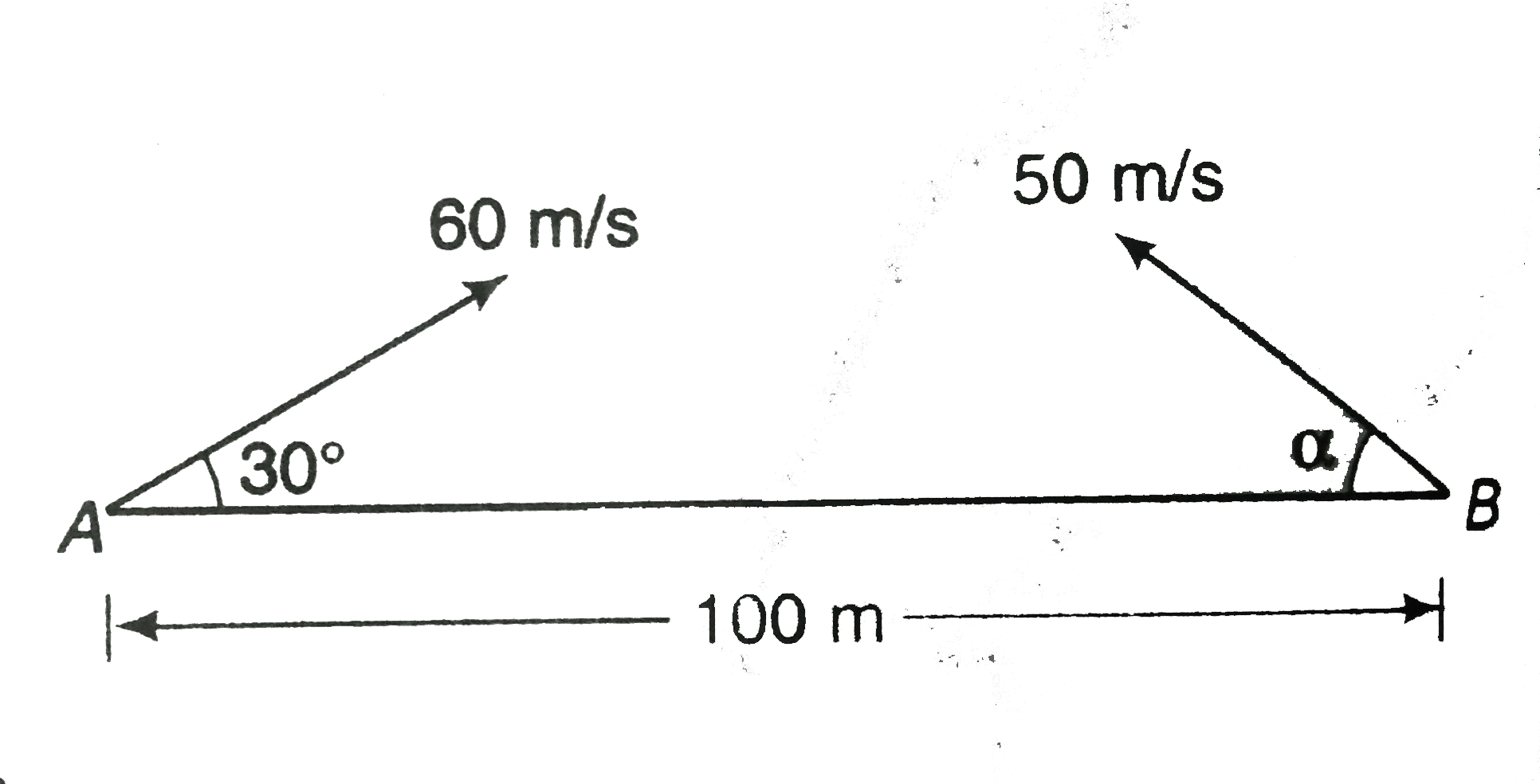
View SolutionThree charged particles are initially in position 1, "They are free to move and they come in position" 2 "after some time. Let" U1 and U2 be the electrostatic potential energies in position 1and2. Then
View Solution
