Two capacitors of and are connected to 200 V and 100 V sources, respectively. If they are connected by the wire, then what is the common potential of the capacitors?
133.3 V
150 V
300 V
400 V
The correct Answer is:A
To solve the problem of finding the common potential of two capacitors connected together after being charged by different voltage sources, we can follow these steps:
1. Identify the Given Values:
- Capacitor 1 (C1) = 10 pF
- Capacitor 2 (C2) = 20 pF
- Voltage across C1 (V1) = 200 V
- Voltage across C2 (V2) = 100 V
2. Calculate the Initial Charges:
- The charge on capacitor 1 (Q1) is calculated using the formula:
Q1=C1×V1=10pF×200V=2000pC
- The charge on capacitor 2 (Q2) is calculated using the formula:
Q2=C2×V2=20pF×100V=2000pC
3. Total Initial Charge:
- The total initial charge (Q_initial) when the capacitors are connected to their respective voltage sources is:
Qinitial=Q1+Q2=2000pC+2000pC=4000pC
4. Connecting the Capacitors:
- Once the batteries are removed and the capacitors are connected together, they will share the total charge. Let V be the common potential across both capacitors when they are connected.
5. Using Charge Conservation:
- The total charge after connecting the capacitors (Q_final) is given by:
Qfinal=Q1′+Q2′=C1×V+C2×V
- This can be expressed as:
Qfinal=(C1+C2)×V
6. Setting Up the Equation:
- Since charge is conserved, we can set the initial charge equal to the final charge:
4000pC=(C1+C2)×V
- Substituting the values of C1 and C2:
4000pC=(10pF+20pF)×V
- This simplifies to:
4000pC=30pF×V
7. Solving for the Common Potential (V):
- Rearranging the equation to solve for V gives:
V=4000pC30pF=400030V≈133.33V
Final Answer:
The common potential of the capacitors when connected together is approximately 133.33 V.
---
- Capacitor 1 (C1) = 10 pF
- Capacitor 2 (C2) = 20 pF
- Voltage across C1 (V1) = 200 V
- Voltage across C2 (V2) = 100 V
- The charge on capacitor 1 (Q1) is calculated using the formula:
Q1=C1×V1=10pF×200V=2000pC
- The charge on capacitor 2 (Q2) is calculated using the formula:
Q2=C2×V2=20pF×100V=2000pC
- The total initial charge (Q_initial) when the capacitors are connected to their respective voltage sources is:
Qinitial=Q1+Q2=2000pC+2000pC=4000pC
- Once the batteries are removed and the capacitors are connected together, they will share the total charge. Let V be the common potential across both capacitors when they are connected.
- The total charge after connecting the capacitors (Q_final) is given by:
Qfinal=Q1′+Q2′=C1×V+C2×V
- This can be expressed as:
Qfinal=(C1+C2)×V
- Since charge is conserved, we can set the initial charge equal to the final charge:
4000pC=(C1+C2)×V
- Substituting the values of C1 and C2:
4000pC=(10pF+20pF)×V
- This simplifies to:
4000pC=30pF×V
- Rearranging the equation to solve for V gives:
V=4000pC30pF=400030V≈133.33V
The common potential of the capacitors when connected together is approximately 133.33 V.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (B) Chapter exercises
17 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Medical entrances gallery
37 Videos
Similar Questions
Two capacitors having capacitances C1 and C2 are charged with 120 V and 200 V batteries respectively. When they are connected in parallel now, it is found that the potential on each one of them is zero. Then,
View SolutionTwo capacitors having capacitances C1 and C2 are charged with 120 V and 200 V batteries respectively. When they are connected in parallel now, it is found that the potential on each one of them is zero. Then,
View Solution
Knowledge Check
A 5μF capacitor is connected to a 200 V, 100 Hz ac source. The capacitive reactance is
A212ΩB312ΩC318ΩD412Ω
Two capacitors of 2μF and 3μF are charged to 150V and 120V, respectively. The plates of capacitor are connected as shown in the figure. An uncharged capacitor of capacity 1.5μF falls to the free end of the wire. Then
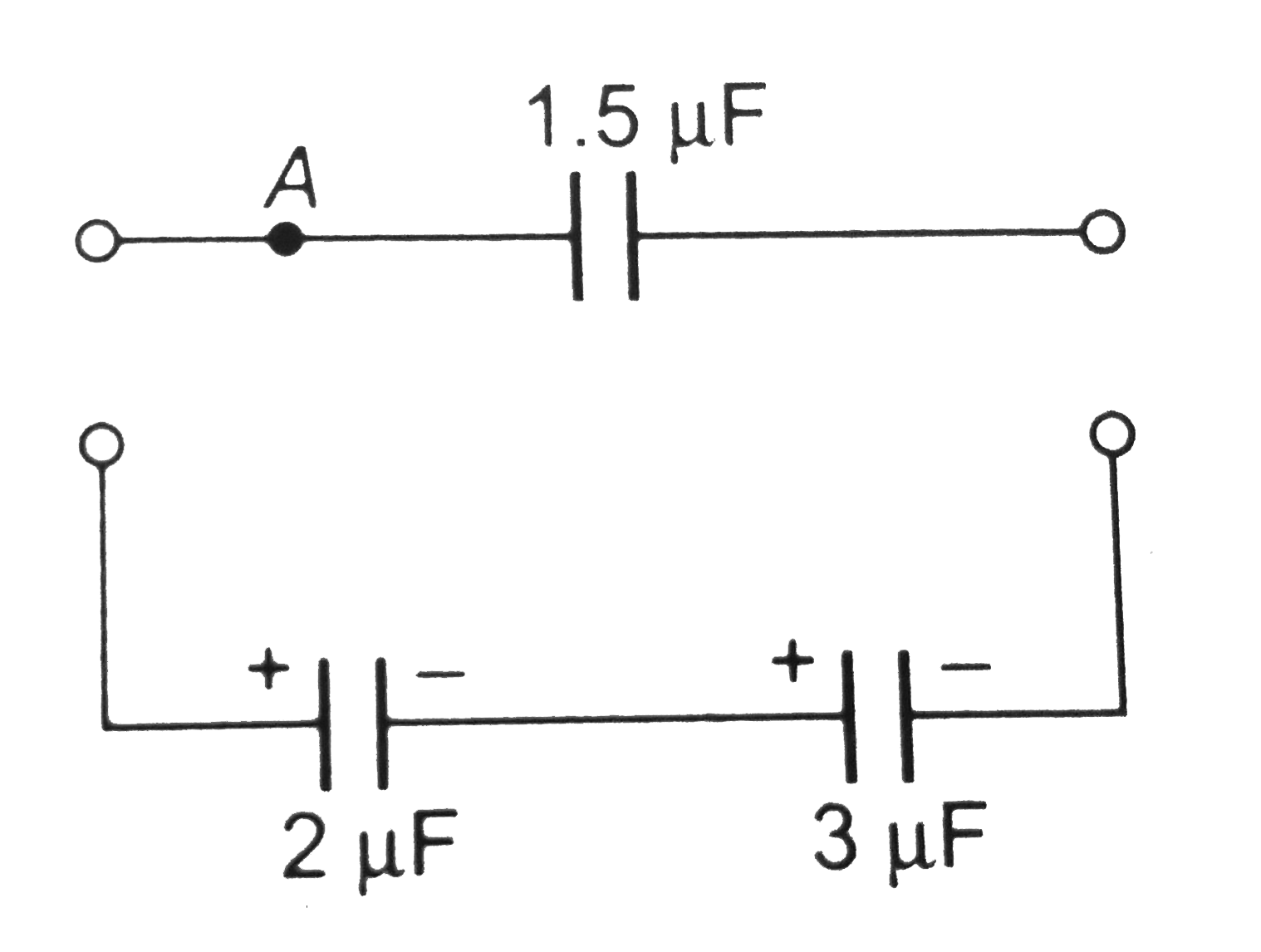
View SolutionTwo capacitors of 2μF and 3μF are charged to 150V and 120V, respectively. The plates of capacitor are connected as shown in the figure. An uncharged capacitor of capacity 1.5μF falls to the free end of the wire. Then

View SolutionTwo capacitors of 10μF and 20μF are connected in series with a 30V battery. The charge on the capacitors will be, respectively
View SolutionTwo capacitors C1 and C2 are charged to 120V and 200V respectively. It is found that connecting them together the potential on each one can be made zero. Then
View SolutionTwo capacitors of capacitance 2μFand5μF are charged to a potential difference 100V and 50 V respectively and connected such that the positive plate of one capacitor is connected to the negative plate of the other capacitor after the switch is closed, the initial current in the circuit is 50 mA. the total resistance of the connecting wires is (in Ohm):
View SolutionIf the two plates of the charged capacitor are connected by a wire, then
View SolutionThree capcaitors of capacities 1μF,2μF and 3μF are charged by 10V,20V and 30V respectively. Now positive plates of first two capacitors are connected with the negative plate of third capacitor on one side and negative plates of first wo capacitors are connectes with positive of third capacitor on the other side. Find
a. common potential V
b. final charges on different capacitors.
View Solution
