A straight wire of length 30 cm and mass 60 mg lies in a direction 30∘ east of north.The earth's magnetic field at this is horizontal and has a magnitude of 0.8 G.What current must bre passed through the wire,so that it may float in air ?
To solve the problem, we need to determine the current that must flow through a straight wire so that it can float in the air, balancing the gravitational force acting on it with the magnetic force due to the Earth's magnetic field.
1. Identify the Given Values:
- Length of the wire, L=30cm=30×10−2m
- Mass of the wire, m=60mg=60×10−6kg
- Acceleration due to gravity, g=9.8m/s2
- Magnetic field strength, B=0.8G=0.8×10−4T
- Angle θ=30∘
2. Calculate the Weight of the Wire:
The weight FW of the wire can be calculated using the formula:
FW=m⋅g
Substituting the values:
FW=(60×10−6kg)⋅(9.8m/s2)=5.88×10−5N
3. Set Up the Equation for Floating Condition:
For the wire to float, the magnetic force FB must equal the weight FW:
FB=I⋅B⋅L⋅sin(θ)
Thus, we set:
FW=FB
m⋅g=I⋅B⋅L⋅sin(θ)
4. Rearranging to Find Current I:
We can rearrange the equation to solve for I:
I=m⋅gB⋅L⋅sin(θ)
5. Substituting the Values:
Now, substitute the known values into the equation:
- m=60×10−6kg
- g=9.8m/s2
- B=0.8×10−4T
- L=30×10−2m
- sin(30∘)=0.5
Substituting these values:
I=(60×10−6)⋅(9.8)(0.8×10−4)⋅(30×10−2)⋅(0.5)
6. Calculating the Current:
First, calculate the numerator:
60×10−6⋅9.8=5.88×10−5N
Then calculate the denominator:
0.8×10−4⋅30×10−2⋅0.5=0.8×30×0.5×10−6=12×10−6=1.2×10−5N
Now, substituting back:
I=5.88×10−51.2×10−5=4.9A
7. Final Calculation:
The final result for the current I that must flow through the wire is approximately:
I≈50A
Conclusion:
The current that must be passed through the wire so that it may float in air is 50 A.
- Length of the wire, L=30cm=30×10−2m
- Mass of the wire, m=60mg=60×10−6kg
- Acceleration due to gravity, g=9.8m/s2
- Magnetic field strength, B=0.8G=0.8×10−4T
- Angle θ=30∘
The weight FW of the wire can be calculated using the formula:
FW=m⋅g
Substituting the values:
FW=(60×10−6kg)⋅(9.8m/s2)=5.88×10−5N
For the wire to float, the magnetic force FB must equal the weight FW:
FB=I⋅B⋅L⋅sin(θ)
Thus, we set:
FW=FB
m⋅g=I⋅B⋅L⋅sin(θ)
We can rearrange the equation to solve for I:
I=m⋅gB⋅L⋅sin(θ)
Now, substitute the known values into the equation:
- m=60×10−6kg
- g=9.8m/s2
- B=0.8×10−4T
- L=30×10−2m
- sin(30∘)=0.5
I=(60×10−6)⋅(9.8)(0.8×10−4)⋅(30×10−2)⋅(0.5)
First, calculate the numerator:
60×10−6⋅9.8=5.88×10−5N
Then calculate the denominator:
0.8×10−4⋅30×10−2⋅0.5=0.8×30×0.5×10−6=12×10−6=1.2×10−5N
Now, substituting back:
I=5.88×10−51.2×10−5=4.9A
The final result for the current I that must flow through the wire is approximately:
I≈50A
The current that must be passed through the wire so that it may float in air is 50 A.
Topper's Solved these Questions
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Check point
54 Videos
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Taking it together
75 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Level 2 Subjective
5 Videos
- MAGNETICSBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETICSEXERCISE - MCQ_TYPE
1 Videos
Similar Questions
A straight wire of mass 400 g and length 2m carries a current of 2A. It is suspended in mid air by a uniform horizontal field B. What is the magnitude of the magnetic field ?
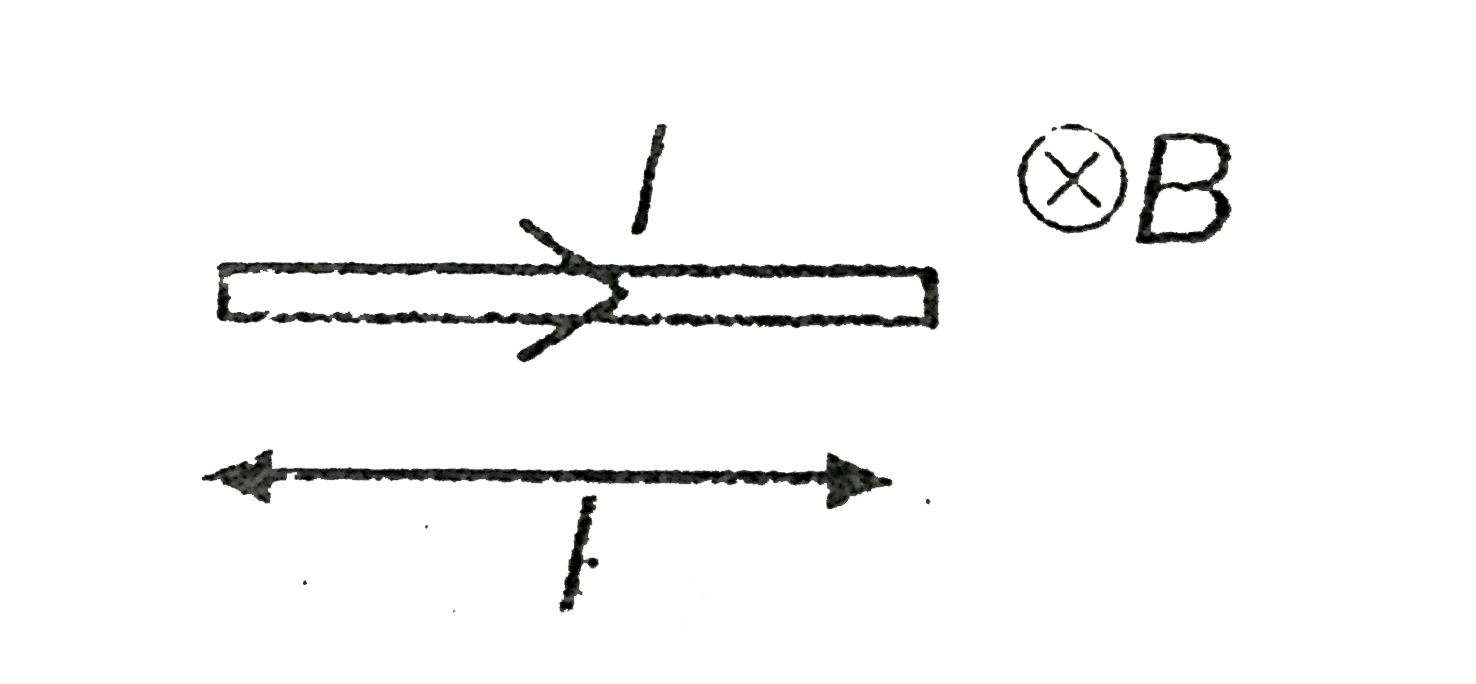
View SolutionA straight wire of mass 400 g and length 2m carries a current of 2A. It is suspended in mid air by a uniform horizontal field B. What is the magnitude of the magnetic field ?

View Solution
Knowledge Check
A telephonic cable at a place has four long straight horizontal wires carrying a current of 1.0amp. in the same direction east to west. The earth's magnetic field at the place is 0.39G and the angle of dip is 35∘. The magnetic declination is almost zero. What are the resultant magnetic fields at points 4.0cm below and above the cable?
A0.25GB0.50GC1.25GD2.50G
A loop of flexible conducting wire of length l lies in magnetic field B which is normal to the plane of loop. A current l is passed through the loop. The tension developed in the wire to open up is
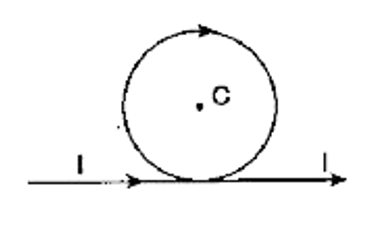
View SolutionA long straight wire is bent as shown below. Find the resultant magnetic field B at the centre C of the circular path of radius 2 cm if a current I of 5A is passed through the wire.
View SolutionA conducting rod of 1 m length and 1 kg mass is suspended by two verticle wires through its ends. An external magnetic field of 2T is applied normal to the rod. Now the current to be passed through the rod so as to make the tension in the wire zero is
[Take g=10ms−2]
View SolutionA straight wire of mass 200 g and length 1.5 m carries a current of 2 A. It is suspended in mid-air by a uniform horizontal magnetic field B. What is the magnitude of the magnetic field?
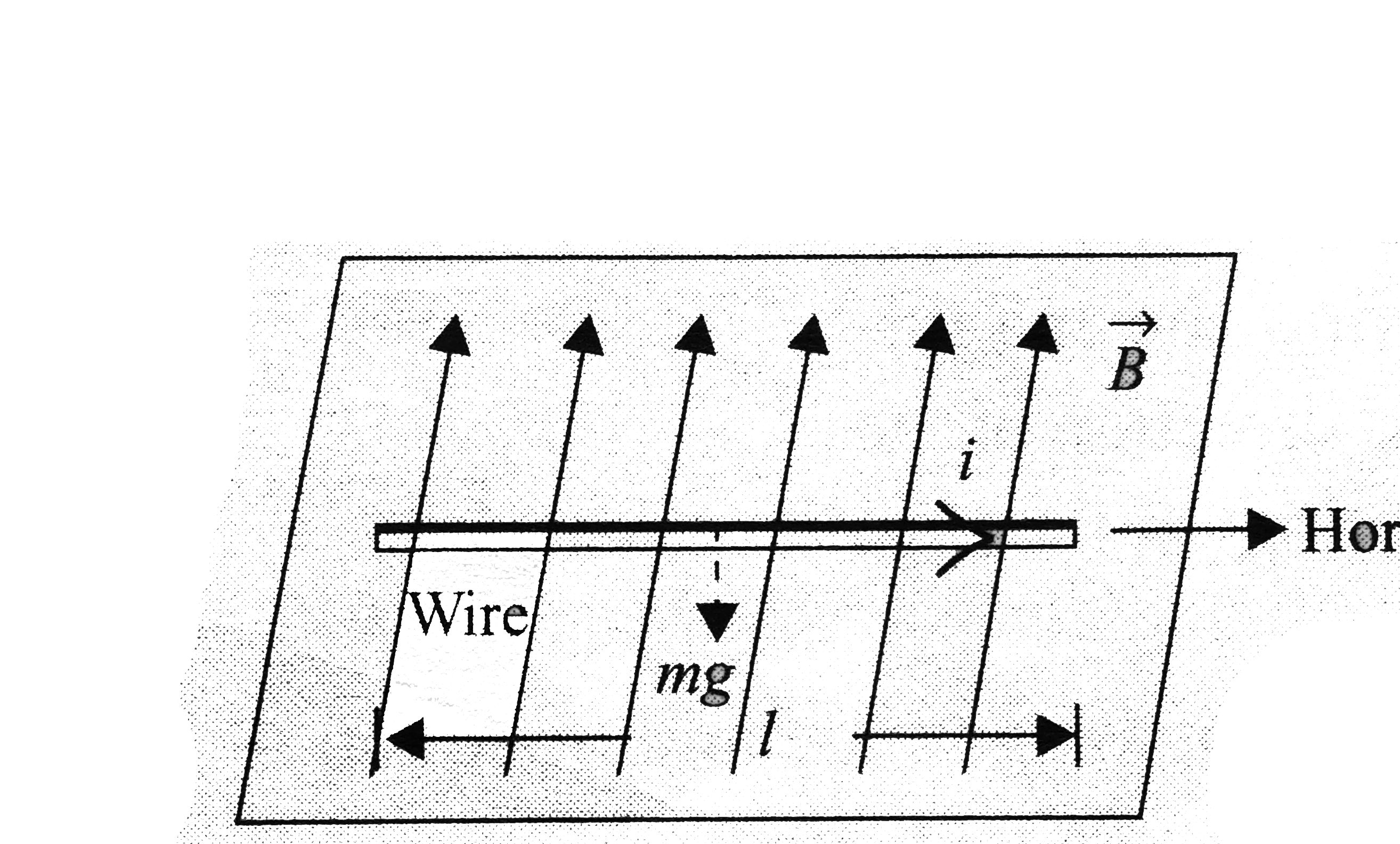
View SolutionA straight wire of mass 200 g and length 1.5 m carries a current of 2 A. It is suspended in mid-air by a uniform horizontal magnetic field B. What is the magnitude of the magnetic field?

View SolutionA straight wire of mass 200 g and length 1.5 m carries a current of 2 A. It is suspended in mid-air by a uniform horizontal magnetic field B. What is the magnitude of the magnetic field?

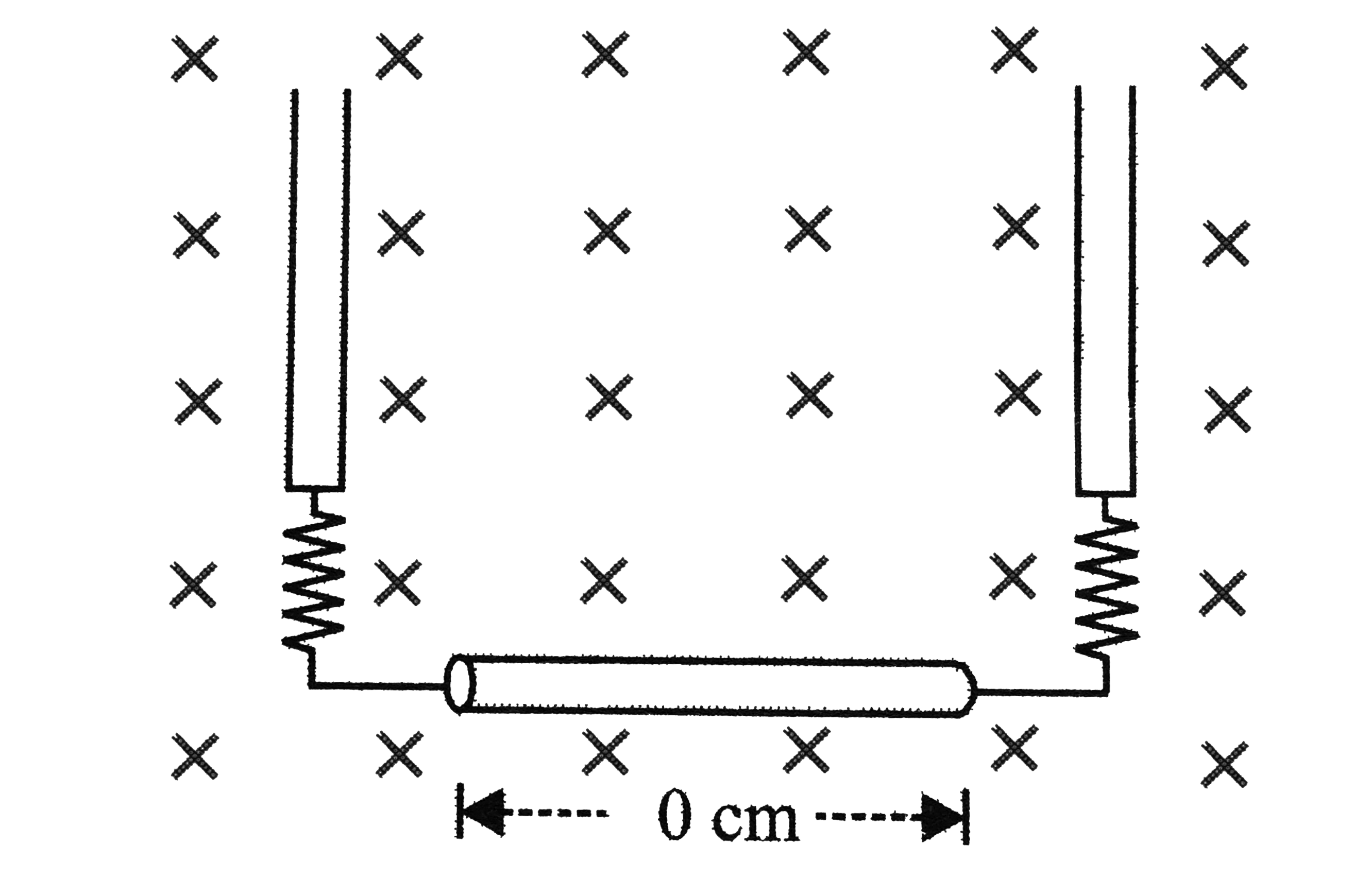
View SolutionA wire of 60 cm length and mass 16 gm is suspended by a pair of flexible leads in a magnetic field of induction 0.40 T. What are the magnitude and direction of the current required to remove the tension in the supporting leads?
View Solution
