A long straight wire is bent as shown below. Find the resultant magnetic field B at the centre C of the circular path of radius 2 cm if a current I of 5A is passed through the wire.
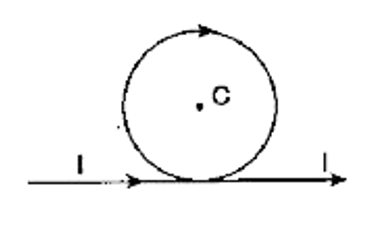
B=μ0I2R
μ0=4π×10−7 H
I = 5A
R=2 cm = 0.02 m
B=4π×10−7×52×0.02 Tesla
B = 1.570×10−4 Tesla
Similar Questions

A conducting wire carrying a current I is bent into the shape as shown. The net magnetic field at the centre O of the circular arc of radius RView SolutionA long current carrying wire is bent as shown. The magnetic field induction at O is
 View Solution
View SolutionFind the magnetic field B due to a semicircular wire of radius 10.0 cm carrying a current of 5.0A at its centre of curvature .
View SolutionA long straight wire carrying current i=10A lies along y-axis. Find the magnetic field at P(3cm, 2cm,4cm).
 View Solution
View SolutionA part of a long wire carrying a current i is bent into a circle of radius r as shown in figure. The net magnetic field at the centre O of the circular loop is :
 View Solution
View SolutionA part of a long wire carrying a current i is bent into a circle of radius r as shown in figure. The net magnetic field at the centre O of the circular loop is
 View Solution
View SolutionA long wire carrying current 'I' is bent into the shape as shown in the figure. The net magnetic field intensity at the centre 'O' is
 View Solution
View SolutionA long wire bent as shown in fig. carries current I. If the radius of the semicircular portion is a, the magnetic field at centre C is
 View Solution
View SolutionA long wire carrying a steady current is bent into a circular loop of one turn. The magnetic field at the centre of the loop is B. it is then bent into a circular coil of n turns. The magnetic field at the centre of this coil of n turns will be
View SolutionTwo very long, straight wires carrying, currents as shown in Fig. Find all locations where the net magnetic field is zero.
 View Solution
View Solution

