The magnetic field existing in a region is given by ,B=B0(xL+x2L2)ˆk.A square loop edge L carrying a current I is placed with its parallel to the X and Y-axis Find the net magnetic force experienced by the loop
To solve the problem of finding the net magnetic force experienced by a square loop carrying a current in a magnetic field defined by B=B0(xL+x2L2)^k, we will follow these steps:
Step 1: Understand the Configuration
- The magnetic field B is in the z-direction (along ^k).
- The square loop has edges of length L and is positioned in the xy-plane.
- The current I flows through the loop.
Step 2: Identify the Forces on Each Edge
The square loop has four edges: AB, BC, CD, and DA. We will calculate the magnetic force on each edge using the formula:
→F=I→L×→B
where →L is the length vector of the edge.
Step 3: Calculate the Force on Edge AB
- Edge AB is along the x-axis from (0,0) to (L,0).
- The length vector →LAB=L^i.
- The magnetic field B at any point x on this edge is:
B=B0(xL+x2L2)^k
- The force on edge AB is:
→FAB=I→LAB×→B=IL^i×B0(xL+x2L2)^k
- Integrate over x from 0 to L:
FAB=I∫L0LB0(xL+x2L2)dx
Calculating the integral:
FAB=IB0(x22+x33L)L0=IB0(L22+L33L)=IB0(L22+L23)=IB0(5L26)
Step 4: Calculate the Force on Edge CD
- Edge CD is parallel to AB and experiences the same magnetic field.
- Thus, FCD=−FAB=−IB05L26 (acting in the opposite direction).
Step 5: Calculate the Force on Edge BC
- Edge BC is along the y-axis from (L,0) to (L,L).
- The length vector →LBC=L^j.
- The magnetic field at x=L:
B=B0(LL+L2L2)=2B0
- The force on edge BC is:
→FBC=IL^j×2B0^k=2IB0L^i
Step 6: Calculate the Force on Edge DA
- Edge DA is parallel to BC and experiences the same magnetic field.
- At x=0, the magnetic field is B=0, so FDA=0.
Step 7: Sum the Forces
Now, we can sum the forces:
Net Force=FAB+FBC+FCD+FDA=(IB05L26−IB05L26+2IB0L+0)=2IB0L
Final Answer
The net magnetic force experienced by the loop is:
→Fnet=2IB0L^i
- The magnetic field B is in the z-direction (along ^k).
- The square loop has edges of length L and is positioned in the xy-plane.
- The current I flows through the loop.
The square loop has four edges: AB, BC, CD, and DA. We will calculate the magnetic force on each edge using the formula:
→F=I→L×→B
where →L is the length vector of the edge.
- Edge AB is along the x-axis from (0,0) to (L,0).
- The length vector →LAB=L^i.
- The magnetic field B at any point x on this edge is:
B=B0(xL+x2L2)^k
- The force on edge AB is:
→FAB=I→LAB×→B=IL^i×B0(xL+x2L2)^k
- Integrate over x from 0 to L:
FAB=I∫L0LB0(xL+x2L2)dx
Calculating the integral:
FAB=IB0(x22+x33L)L0=IB0(L22+L33L)=IB0(L22+L23)=IB0(5L26)
- Edge CD is parallel to AB and experiences the same magnetic field.
- Thus, FCD=−FAB=−IB05L26 (acting in the opposite direction).
- Edge BC is along the y-axis from (L,0) to (L,L).
- The length vector →LBC=L^j.
- The magnetic field at x=L:
B=B0(LL+L2L2)=2B0
- The force on edge BC is:
→FBC=IL^j×2B0^k=2IB0L^i
- Edge DA is parallel to BC and experiences the same magnetic field.
- At x=0, the magnetic field is B=0, so FDA=0.
Now, we can sum the forces:
Net Force=FAB+FBC+FCD+FDA=(IB05L26−IB05L26+2IB0L+0)=2IB0L
The net magnetic force experienced by the loop is:
→Fnet=2IB0L^i
Topper's Solved these Questions
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Check point
54 Videos
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Taking it together
75 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Level 2 Subjective
5 Videos
- MAGNETICSBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETICSEXERCISE - MCQ_TYPE
1 Videos
Similar Questions
The magnetic field existing in a region is given by →B=B0(1+xl)→k. A square loop of edge l and carrying a current I, is placed with its edges parallel to the x-y axes. Find the magnitude of the net magnetic force experienced by the loop.
View SolutionThe magnetic field existing in a region is gicen by →B=B0(1+xl)→k. A square loop of edge l and carrying a current I, is placed with its edges parallel to the x-y axes. Find the magnitude of the net magnetic force experienced by the loop.
View SolutionThe magnetic field in a region is given by →B=B0(1+xa)ˆk. A square loop of edge length 'd' is placed with its edge along X-axis and Y-axis. The loop is moved with a constant velocity →V=V0ˆi. The emf induced in the loop is
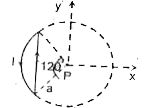
View SolutionA wire loop carrying current I is placed in the x-y plane as shown. Magnetic induction at P is
View SolutionA square loop OABCO of side of side l carries a current i. It is placed as shown in figure. Find the magnetic moment of loop.

View SolutionA square loop OABCO of side of side l carries a current i. It is placed as shown in figure. Find the magnetic moment of loop.

View SolutionA hypthetical magnetic field existing in a region is given by →B=B0→er, where →–––r denotes the uit vector along the redial direction. A circular loop of radus a, carrying a current I, is placed with its plane parallel to the x-y plane and the centre at (0,0, d) .Find the magnitude of the magnetic force acting on the loop.
View SolutionThe magnetic field I nan reigion is given by B=B0XaK. A Squrae edges along the x and y axis. The loop is moved with a constant velocity . The emf induced in the loop is

View SolutionA circular flexible loop of wire of radius r carrying a current I is placed in a uniform magnetic field B . If B is doubled, then tension in the loop
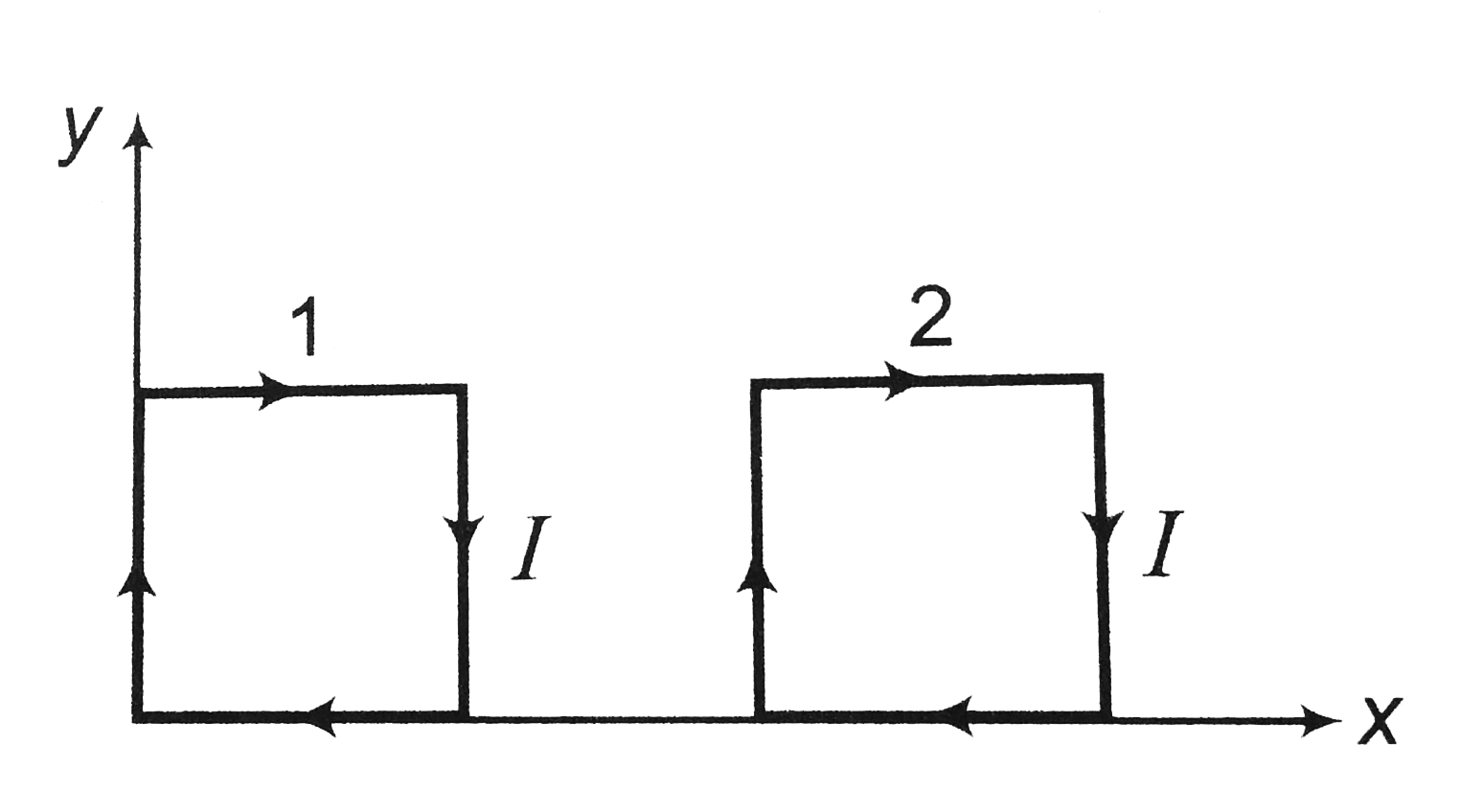
View SolutionMagnetic field in a region is given by B=B0xˆk. Two loops each of side a is placed in the magnetic region in the xy-plane with one of its sides on x-axis. If F1 is the force on loop 1 and dF2 be the force on loop 2, then
View Solution
