A proton of mass m and charge q is moving in a plane with kinetic energy E. if there exists a uniform magnetic field B, perpendicular to the plane motion. The proton will move in a circular path of radius
3EmqB
√2EmqB
√Em2qB
√2EqqB
The correct Answer is:b
To find the radius of the circular path of a proton moving in a magnetic field, we can follow these steps:
Step 1: Understand the Forces Acting on the Proton
When a charged particle like a proton moves in a magnetic field, it experiences a magnetic force that acts as the centripetal force required for circular motion. The magnetic force FB is given by:
FB=qvB
where:
- q is the charge of the proton,
- v is the velocity of the proton,
- B is the magnetic field strength.
Step 2: Set Up the Equation for Circular Motion
For an object moving in a circular path, the centripetal force Fc is given by:
Fc=mv2R
where:
- m is the mass of the proton,
- R is the radius of the circular path.
Step 3: Equate the Forces
Since the magnetic force provides the necessary centripetal force, we can set the two forces equal to each other:
qvB=mv2R
Step 4: Solve for the Radius R
Rearranging the equation to solve for R:
R=mvqB
Step 5: Relate Velocity to Kinetic Energy
The kinetic energy E of the proton is related to its mass and velocity by the equation:
E=12mv2
From this, we can express the velocity v in terms of kinetic energy:
v=√2Em
Step 6: Substitute Velocity into the Radius Equation
Now, substituting v back into the equation for R:
R=m√2EmqB
This simplifies to:
R=√2mEqB
Final Answer
Thus, the radius of the circular path of the proton is given by:
R=√2mEqB
When a charged particle like a proton moves in a magnetic field, it experiences a magnetic force that acts as the centripetal force required for circular motion. The magnetic force FB is given by:
FB=qvB
where:
- q is the charge of the proton,
- v is the velocity of the proton,
- B is the magnetic field strength.
For an object moving in a circular path, the centripetal force Fc is given by:
Fc=mv2R
where:
- m is the mass of the proton,
- R is the radius of the circular path.
Since the magnetic force provides the necessary centripetal force, we can set the two forces equal to each other:
qvB=mv2R
Rearranging the equation to solve for R:
R=mvqB
The kinetic energy E of the proton is related to its mass and velocity by the equation:
E=12mv2
From this, we can express the velocity v in terms of kinetic energy:
v=√2Em
Now, substituting v back into the equation for R:
R=m√2EmqB
This simplifies to:
R=√2mEqB
Thus, the radius of the circular path of the proton is given by:
R=√2mEqB
Topper's Solved these Questions
- MAGNETIC FIELD AND FORCESBook:DC PANDEY ENGLISHChapter:MAGNETIC FIELD AND FORCESExercise:Match the following
Explore 4 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBook:DC PANDEY ENGLISHChapter:INTERFERENCE AND DIFFRACTION OF LIGHTExercise:Level 2 Subjective
Explore 5 Videos
- MAGNETICSBook:DC PANDEY ENGLISHChapter:MAGNETICSExercise:MCQ_TYPE
Explore 1 Video
Similar Questions
An electron of mass m and charge e is accelerated by a potential difference V. It then enters a uniform magnetic field B applied perpendicular to its path. The radius of the circular path of the electron is
View SolutionAn electron having mass 'm' and kinetic energy E enter in uniform magnetic field B perpendicularly, then its frequency will be : -
View SolutionAn electron and a proton are moving with the same kinetic energy along the same direction, When they pass through a uniform magnetic field perpendicular to the direction of their motion, they describe circular path of the same radius. Is this statement true or false?
View SolutionA charged particle of mass m and charge q is accelerated through a potential differences of V volts. It enters of uniform magnetic field B which is directed perpendicular to the direction of motion of the particle. The particle will move on a circular path of radius
View SolutionA stream of protons and deuterons in a vacuum chamber enters a uniform magnetic field. Both protons and deuterons have been subjected to same accelerating potential, hence the kinetic energies of the particles are the same. If the ion-stream is perpendicular to the magnetic field and the protons move in a circular path of radius 15 cm, find the radius of the path traversed by the deuterons. Given that mass of deuteron is twice that of a proton.
View SolutionA proton of mass m and charge q enters a region of uniform magnetic field of a magnitude B with a velocity v directed perpendicular to the magnetic field. It moves in a circular path and leaves the magnetic field after completing a quarter of a circle. The time spent by the proton inside the magnetic field is proportional to:
View SolutionThe counductor AD moves to the right in a uniform magnetic field directed into the plane of the paper.
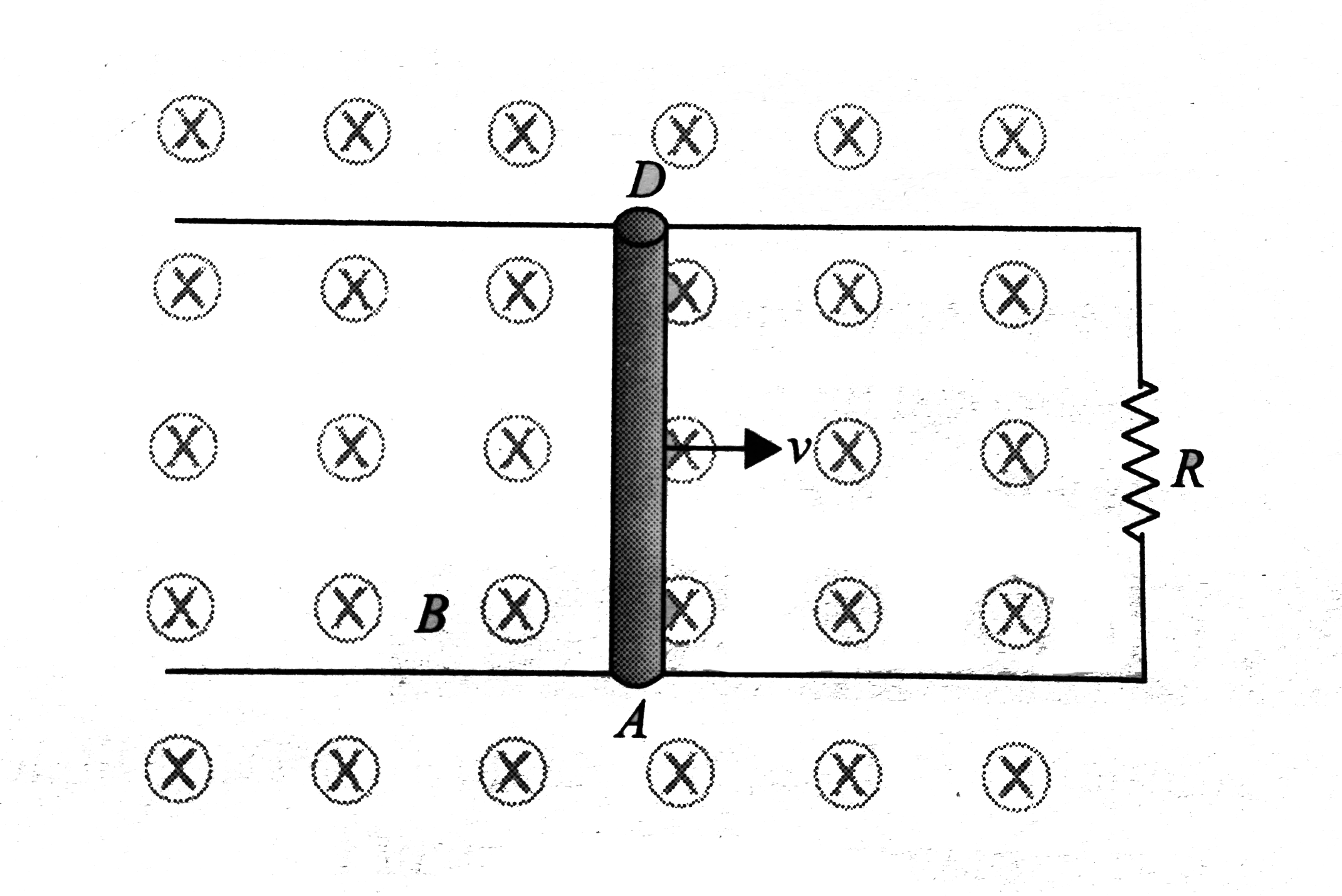
View SolutionThe change in the kinetic energy of a charged particle moving in a uniform magnetic field will be zero when its velocity is
View SolutionA proton and an α−particle, moving with the same velocity, enter a uniform magnetic field, acting normal to the plane of their motion. The ratio of the radii of the circular paths descirbed by the proton and α-particle is
View SolutionA proton an an α−particle, moving with the same velocity, enter a uniform magnetic field, acting normal to the plane of their motion. The ratio of the radii of the circular paths descirbed by the proton and α-particle is
View Solution
