Figure represents the moment of inertia of the solid sphere about an axis parallel to the diameter of the solid sphere and at a distance x from t. Which one of the following represents the variations of I with x ?

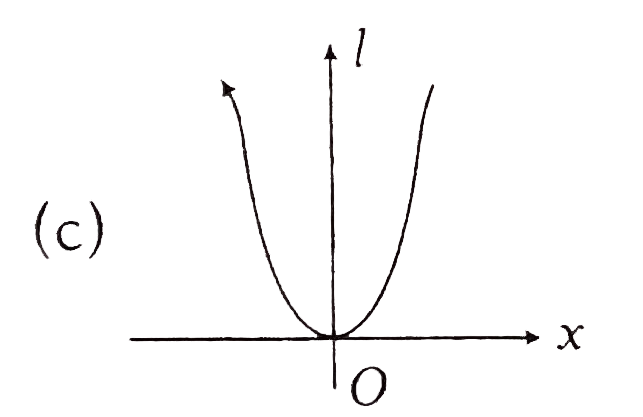
The correct Answer is:D
To solve the problem regarding the variation of the moment of inertia I of a solid sphere about an axis parallel to its diameter and at a distance x from it, we can follow these steps:
Step 1: Understand the Moment of Inertia Formula
The moment of inertia I about an axis parallel to the diameter of the sphere can be expressed using the parallel axis theorem. The formula is given by:
I=ICM+md2
where:
- ICM is the moment of inertia about the center of mass,
- m is the mass of the sphere,
- d is the distance from the center of mass to the new axis of rotation.
Step 2: Identify the Variables
In this case, the distance d is equal to x (the distance from the center of the sphere to the new axis). Thus, we can rewrite the equation as:
I=ICM+mx2
Step 3: Determine the Moment of Inertia about the Center of Mass
For a solid sphere, the moment of inertia about its center of mass is given by:
ICM=25mr2
where r is the radius of the sphere.
Step 4: Substitute ICM into the Equation
Substituting ICM into our equation for I:
I=25mr2+mx2
Step 5: Analyze the Equation
The equation I=25mr2+mx2 shows that I is a quadratic function of x. The term mx2 indicates that as x increases, I will increase quadratically.
Step 6: Graph the Relationship
The graph of I versus x will be a parabola that opens upwards. Since the constant term 25mr2 is present, the parabola does not pass through the origin.
Conclusion
Thus, the variation of the moment of inertia I with respect to the distance x is represented by a parabola that does not pass through the origin.
Final Answer
The correct representation of the variation of I with x is a parabola not passing through the origin.
---
The moment of inertia I about an axis parallel to the diameter of the sphere can be expressed using the parallel axis theorem. The formula is given by:
- ICM is the moment of inertia about the center of mass,
- m is the mass of the sphere,
- d is the distance from the center of mass to the new axis of rotation.
In this case, the distance d is equal to x (the distance from the center of the sphere to the new axis). Thus, we can rewrite the equation as:
For a solid sphere, the moment of inertia about its center of mass is given by:
Substituting ICM into our equation for I:
The equation I=25mr2+mx2 shows that I is a quadratic function of x. The term mx2 indicates that as x increases, I will increase quadratically.
The graph of I versus x will be a parabola that opens upwards. Since the constant term 25mr2 is present, the parabola does not pass through the origin.
Thus, the variation of the moment of inertia I with respect to the distance x is represented by a parabola that does not pass through the origin.
The correct representation of the variation of I with x is a parabola not passing through the origin.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - (B) Chapter Exercises25videos
ROTATION
- BOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - (C) Chapter Exercises39videos
ROTATION
- BOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - Check point 9.315videos
ROTATION
- BOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Integer type q.14videos
RAY OPTICS
- BOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Subjective Questions2videos
ROTATIONAL MECHANICS
Similar Questions
The moment of inertia of a solid sphere, about an axis parallel to its diameter and at a distance of x form it is, 'I(x)'. Which one of the graphs represents the variation of 'I(x) with x correctly ?
View SolutionMoment of inertia I of a solid sphere about an axis parallel to a diameter and at a distance x from it varies as:
View SolutionWhat is the moment of inertia of a solid sphere of density ρ and radius R about its diameter ?
View SolutionWhat is the moment of inertia of solid sphere of density ρ and radius R about its diameter ?
View SolutionThe moment of inertia of cylinder of radius a, mass M and height h about an axis parallel to the axis of the cylinder and distance b from its centre is :
View SolutionFind the moment of inertia of a solid cylinder of mass M and radius R about a line parallel to the axis of the cylinder and on the surface of the cylinder.
View SolutionThe moment of inertia of a solid sphere of radius R about its diameter is same as that of a disc of radius 2R about its diameter. The ratio of their masses is
View SolutionWhich one of the following is correctly matched ?
View SolutionA square plate has a moment of inertia l0 about an axis lying in its plane, passing through its centre and making an angle θ with one of the sides. Which graph represents the variation of l with θ ?
View SolutionThe moment of inertia of a solid sphere about an axis passing through the centre radius is 25MR2 , then its radius of gyration about a parallel axis t a distance 2R from first axis is
View Solution

