Column IColumn II(A)GMeMs(p)[M2L2T−3](B)3RTM(q)[ML3T−2](C)F2q2B2(r)[L2T−2](D)GMeRe(s)None
To solve the problem of matching the expressions in Column I with their corresponding dimensions in Column II, we will analyze each option step by step.
Step 1: Analyze Option A - GMeMs
- Expression: GMeMs
- Given Dimensions: (M2L2T−3)
Calculation:
1. The gravitational constant G has dimensions of (M−1L3T−2).
2. Me and Ms are both masses, so their dimensions are (M).
3. Therefore, the dimensions of GMeMs are:
(M−1L3T−2)⋅(M)⋅(M)=(M1L3T−2)
4. This does not match the given dimensions of (M2L2T−3).
Step 2: Analyze Option B - 3RTM
- Expression: 3RTM
- Given Dimensions: (ML3T−2)
Calculation:
1. The ideal gas constant R has dimensions of (ML2T−2K−1).
2. Temperature T has dimensions of (K).
3. Therefore, the dimensions of 3RT are:
(ML2T−2K−1)⋅(K)=(ML2T−2)
4. Dividing by mass M gives:
(ML2T−2)(M)=(L2T−2)
5. This does not match the given dimensions of (ML3T−2).
Step 3: Analyze Option C - F2q2B2
- Expression: F2q2B2
- Given Dimensions: (L2T−2)
Calculation:
1. The force F has dimensions of (MLT−2).
2. Therefore, the dimensions of F2 are:
(M2L2T−4)
3. The charge q has dimensions of (IT) (where I is current).
4. The magnetic field B has dimensions of (MT−2I−1).
5. Therefore, the dimensions of q2 are:
(I2T2)
6. The dimensions of B2 are:
(M2T−4I−2)
7. Thus, the dimensions of F2q2B2 are:
(M2L2T−4)(I2T2)⋅(M2T−4I−2)=(L2T−2)
8. This matches the given dimensions.
Step 4: Analyze Option D - GMeRe
- Expression: GMeRe
- Given Dimensions: "None"
Calculation:
1. Using the dimensions of G as (M−1L3T−2), Me as (M), and Re (a distance) as (L):
(M−1L3T−2)⋅(M)(L)=(M0L2T−2)=(L2T−2)
2. This does not match "None".
Final Matching:
- A: Does not match.
- B: Does not match.
- C: Matches with (L2T−2).
- D: Matches with (L2T−2) but is labeled "None".
Conclusion:
- A: None
- B: None
- C: Matches with (L2T−2)
- D: Matches with (L2T−2) but is labeled "None".
Final Answer:
- A: None
- B: None
- C: Matches with (L2T−2)
- D: Matches with "None"
- Expression: GMeMs
- Given Dimensions: (M2L2T−3)
1. The gravitational constant G has dimensions of (M−1L3T−2).
2. Me and Ms are both masses, so their dimensions are (M).
3. Therefore, the dimensions of GMeMs are:
(M−1L3T−2)⋅(M)⋅(M)=(M1L3T−2)
4. This does not match the given dimensions of (M2L2T−3).
- Expression: 3RTM
- Given Dimensions: (ML3T−2)
1. The ideal gas constant R has dimensions of (ML2T−2K−1).
2. Temperature T has dimensions of (K).
3. Therefore, the dimensions of 3RT are:
(ML2T−2K−1)⋅(K)=(ML2T−2)
4. Dividing by mass M gives:
(ML2T−2)(M)=(L2T−2)
5. This does not match the given dimensions of (ML3T−2).
- Expression: F2q2B2
- Given Dimensions: (L2T−2)
1. The force F has dimensions of (MLT−2).
2. Therefore, the dimensions of F2 are:
(M2L2T−4)
3. The charge q has dimensions of (IT) (where I is current).
4. The magnetic field B has dimensions of (MT−2I−1).
5. Therefore, the dimensions of q2 are:
(I2T2)
6. The dimensions of B2 are:
(M2T−4I−2)
7. Thus, the dimensions of F2q2B2 are:
(M2L2T−4)(I2T2)⋅(M2T−4I−2)=(L2T−2)
8. This matches the given dimensions.
- Expression: GMeRe
- Given Dimensions: "None"
1. Using the dimensions of G as (M−1L3T−2), Me as (M), and Re (a distance) as (L):
(M−1L3T−2)⋅(M)(L)=(M0L2T−2)=(L2T−2)
2. This does not match "None".
- A: Does not match.
- B: Does not match.
- C: Matches with (L2T−2).
- D: Matches with (L2T−2) but is labeled "None".
- A: None
- B: None
- C: Matches with (L2T−2)
- D: Matches with (L2T−2) but is labeled "None".
- A: None
- B: None
- C: Matches with (L2T−2)
- D: Matches with "None"
Topper's Solved these Questions
- UNITS, DIMENSIONS & ERROR ANALYSIS BOOK - DC PANDEY ENGLISHCHAPTER - UNITS, DIMENSIONS & ERROR ANALYSIS EXERCISE - Medical entrances gallery
32 Videos
- UNITS, DIMENSIONS & ERROR ANALYSIS BOOK - DC PANDEY ENGLISHCHAPTER - UNITS, DIMENSIONS & ERROR ANALYSIS EXERCISE - Assertion and reason
15 Videos
- UNIT AND DIMENSIONSBOOK - DC PANDEY ENGLISHCHAPTER - UNIT AND DIMENSIONSEXERCISE - Assertion And Reason
2 Videos
- VECTORSBOOK - DC PANDEY ENGLISHCHAPTER - VECTORSEXERCISE - Medical enrances gallery
9 Videos
Similar Questions
Column IColumn II(A)Work(p)[A1/2T−1](B)Moment of inertia(q)[FA1/2](C)Velocity(r)[FA1/2T2]
View SolutionColumn IColumn II(A)Electrical resistance(p)[M−1L−2T4A2](B)Capacitance(q)[ML2T−2A−2](C)Magnetic field(r)[ML2T−3A−2](D)Inductance(s)[MT−2A−1]
View SolutionLet Rt represents activity of a sample at an insant and Nt represent number of active nuclei in the sample at the instant. T1/2 represents the half life.
Column IColumn II(A)t=T1/2(p)Rt=R02(B)t=T1/2ln2(q)N0−Nt=N02(C)t=32T1/2(r)Rt−R0R0=1−ee(s)Nt=N02√2
View SolutionMatch the following columns.
Column IColumn IIA.Teslap[ML2A−2T−2]B.Weberq[MLA−2T−1]C.Weber m−2r.[MA−1T−2]D.Henrys.None
View SolutionMatch the following
Table-1Table-2(A)L(P)[M0L0T−2](B)Magnetic Flux(Q)[ML2T−2A−1](C)LC(R)[ML2T−2A−2](D)CR2(S)None
View SolutionColumn IColumn II(A)R/L(p)Time(B)C/R(q)Frequency(C)E/B(r)Speed(D)√ε0μ0(s)None
View SolutionMatch the following two columns.
Column IColumn IIA.Electrical resistance1.[ML3T−3A−2]B.Electrical potential2.[ML2T−3A−2]C.Specific resistance3.[ML2T−3A−1]D.Specific conductance4.None
View SolutionColumnIColumnII(A)K.E.P.E.(P)2(B)P.E+2K.E.(Q)−12(C)P.E.T.E.(R)1(D)K.E.T.E.(S)0
View SolutionFour rods of equal length l and mass m each forms a square as shown in figure
Moment of inertia about three axes 1,2 and 3 are say I1,I2andI3. Then, match the following columns
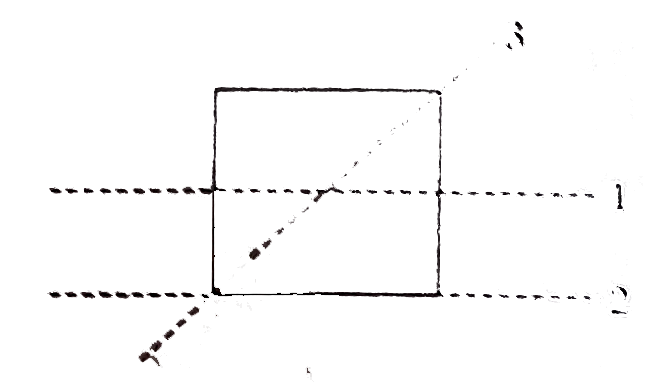
Column-IColumn-II(A)I1(p)43ml2(B)I2(q)23ml2(C)I3(r)12ml2(s)None
View SolutionA.Spring constant1.[M1L2T−2]B.Pascal2.[M0L0T−1]CHertz3.[M1L0T−2]D.Joule4.[M1L−1T−2]
View Solution
