Match the following
Table-1Table-2(A)L(P)[M0L0T−2](B)Magnetic Flux(Q)[ML2T−2A−1](C)LC(R)[ML2T−2A−2](D)CR2(S)None
The correct Answer is:(A) R, (B) Q, (C) S, (D) R
To solve the problem of matching the items from Table-1 with Table-2, we need to analyze each item in Table-1 and determine its dimensional formula.
1. Identify the Dimensional Formula for Self-Inductance (L):
- The formula for the electromotive force (EMF) across an inductor is given by EMF=Ldidt.
- Rearranging gives L=EMF⋅dtdi.
- EMF can be expressed as voltage, which is work done per unit charge: EMF=WQ.
- Therefore, L=W⋅dtQ⋅di.
- The dimensions of work done (W) are (ML2T−2), charge (Q) is (IT), and current (I) is (A).
- Thus, L can be expressed as:
L=(ML2T−2)⋅(T)(IT)⋅(I)=(ML2T−2A−2)
- Therefore, (A) L matches with (R).
2. Identify the Dimensional Formula for Magnetic Flux (Φ):
- Magnetic flux can be expressed as Φ=L⋅I.
- From the previous calculation, we know L has dimensions (ML2T−2A−2).
- Therefore, the dimensions of magnetic flux are:
Φ=(ML2T−2A−2)⋅(A)=(ML2T−2A−1)
- Thus, (B) Magnetic Flux matches with (Q).
3. Identify the Dimensional Formula for LC:
- The relationship between angular frequency (ω) and inductance (L) and capacitance (C) is given by ω=1√LC.
- Rearranging gives LC=1ω2.
- The dimensions of angular frequency ω are (T−1), so:
ω2=(T−2)⟹LC=(T2)
- Therefore, (C) LC matches with (S).
4. Identify the Dimensional Formula for CR²:
- The time constant τ for an RC circuit is given by τ=RC.
- The dimensions of capacitance (C) are (C)=(Q/V) where V=(ML2T−2A−1).
- Thus, C=(IT)(ML2T−2A−1)=(M−1L−2T3A2).
- Therefore, the dimensions of CR2 can be calculated as:
CR2=(M−1L−2T3A2)⋅(T)=(M−1L−2T4A2)
- Since this does not match any specific option, we conclude that (D) CR² matches with (S).
Final Matches:
- (A) L matches with (R)
- (B) Magnetic Flux matches with (Q)
- (C) LC matches with (S)
- (D) CR² matches with (S)
- The formula for the electromotive force (EMF) across an inductor is given by EMF=Ldidt.
- Rearranging gives L=EMF⋅dtdi.
- EMF can be expressed as voltage, which is work done per unit charge: EMF=WQ.
- Therefore, L=W⋅dtQ⋅di.
- The dimensions of work done (W) are (ML2T−2), charge (Q) is (IT), and current (I) is (A).
- Thus, L can be expressed as:
L=(ML2T−2)⋅(T)(IT)⋅(I)=(ML2T−2A−2)
- Therefore, (A) L matches with (R).
- Magnetic flux can be expressed as Φ=L⋅I.
- From the previous calculation, we know L has dimensions (ML2T−2A−2).
- Therefore, the dimensions of magnetic flux are:
Φ=(ML2T−2A−2)⋅(A)=(ML2T−2A−1)
- Thus, (B) Magnetic Flux matches with (Q).
- The relationship between angular frequency (ω) and inductance (L) and capacitance (C) is given by ω=1√LC.
- Rearranging gives LC=1ω2.
- The dimensions of angular frequency ω are (T−1), so:
ω2=(T−2)⟹LC=(T2)
- Therefore, (C) LC matches with (S).
- The time constant τ for an RC circuit is given by τ=RC.
- The dimensions of capacitance (C) are (C)=(Q/V) where V=(ML2T−2A−1).
- Thus, C=(IT)(ML2T−2A−1)=(M−1L−2T3A2).
- Therefore, the dimensions of CR2 can be calculated as:
CR2=(M−1L−2T3A2)⋅(T)=(M−1L−2T4A2)
- Since this does not match any specific option, we conclude that (D) CR² matches with (S).
- (A) L matches with (R)
- (B) Magnetic Flux matches with (Q)
- (C) LC matches with (S)
- (D) CR² matches with (S)
Similar Questions
Match the following.
∣∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣∣Table-1Table-2(A)Coefficient of viscosity(P)[M2L−1T−2](B)Surface tension(Q)[ML0T−2](C)Modulus of elasticity(R)[ML−1T−2](D)Energy per unit volume(S)Noneof a fiuid∣∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣∣
View SolutionMatch the following columns.
Column IColumn IIA.Teslap[ML2A−2T−2]B.Weberq[MLA−2T−1]C.Weber m−2r.[MA−1T−2]D.Henrys.None
View SolutionFour rods of equal length l and mass m each forms a square as shown in figure
Moment of inertia about three axes 1,2 and 3 are say I1,I2andI3. Then, match the following columns
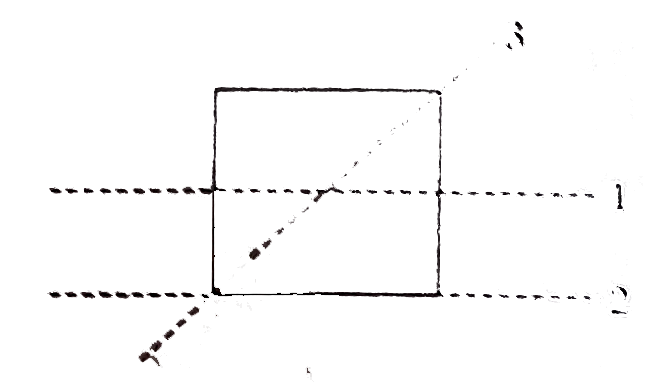
Column-IColumn-II(A)I1(p)43ml2(B)I2(q)23ml2(C)I3(r)12ml2(s)None
View SolutionColumn IColumn II(A)GMeMs(p)[M2L2T−3](B)3RTM(q)[ML3T−2](C)F2q2B2(r)[L2T−2](D)GMeRe(s)None
View SolutionMatch the following
Table - 1Table - 2(A)σ2/ε0(P)C2/J−m(B)ε0(Q)Farad(C)ampere - secondVolt(R)J/m3(D)VE(S)metre
View SolutionColumn IColumn II(A)Electrical resistance(p)[M−1L−2T4A2](B)Capacitance(q)[ML2T−2A−2](C)Magnetic field(r)[ML2T−3A−2](D)Inductance(s)[MT−2A−1]
View SolutionComparing L-C oscillation with the oscillation if spring-block-system, match the following table.
Comparing L-C oscillations with the oscillations of spring-block sytem, match the following table Table-1(LC oscillations)Table-2(Spring-block oscillations)(A)L(P)k(B)C(Q)m(C)i(R)v(D)didt(S)x(T)None
View SolutionMatch the following :
Column-IColumn-II(A)3s1(p)n=3(B)3p1(q)l=0(C)4s1(r)l=1(D)4d1(s)m=0(t)m=±1
View SolutionA.Spring constant1.[M1L2T−2]B.Pascal2.[M0L0T−1]CHertz3.[M1L0T−2]D.Joule4.[M1L−1T−2]
View SolutionMatch the following two columns.
Column IColumn IIA.Electrical resistance1.[ML3T−3A−2]B.Electrical potential2.[ML2T−3A−2]C.Specific resistance3.[ML2T−3A−1]D.Specific conductance4.None
View Solution
