Two blocks of masses 2 kg and 1 kg are in contact with each other on a frictionless table. When a horizontal force of N is applied to the block of mass 2 kg the value of the force of contact between the two blocks is:
4 N
3 N
5 N
1 N
The correct Answer is:D
To solve the problem, we need to find the force of contact between the two blocks when a horizontal force of 3 N is applied to the block of mass 2 kg. Let's break it down step by step.
Step 1: Understand the System
We have two blocks:
- Block A (mass = 2 kg)
- Block B (mass = 1 kg)
They are in contact with each other on a frictionless table. A horizontal force of 3 N is applied to Block A.
Step 2: Calculate the Total Mass
The total mass of the system (both blocks) is:
mtotal=mA+mB=2kg+1kg=3kg
Step 3: Calculate the Acceleration of the System
Using Newton's second law, the acceleration a of the entire system can be calculated as:
F=mtotal⋅a
Where F is the applied force (3 N). Thus:
3N=3kg⋅a
Solving for a:
a=3N3kg=1m/s2
Step 4: Analyze the Forces on Block B
Now, let's analyze the forces acting on Block B (1 kg). The only horizontal force acting on Block B is the contact force N exerted by Block A. According to Newton's second law for Block B:
N=mB⋅a
Substituting the values:
N=1kg⋅1m/s2=1N
Step 5: Conclusion
The force of contact between the two blocks is:
N=1N
Thus, the value of the force of contact between the two blocks is 1 N.
We have two blocks:
- Block A (mass = 2 kg)
- Block B (mass = 1 kg)
The total mass of the system (both blocks) is:
mtotal=mA+mB=2kg+1kg=3kg
Using Newton's second law, the acceleration a of the entire system can be calculated as:
F=mtotal⋅a
Where F is the applied force (3 N). Thus:
3N=3kg⋅a
Solving for a:
a=3N3kg=1m/s2
Now, let's analyze the forces acting on Block B (1 kg). The only horizontal force acting on Block B is the contact force N exerted by Block A. According to Newton's second law for Block B:
N=mB⋅a
Substituting the values:
N=1kg⋅1m/s2=1N
The force of contact between the two blocks is:
N=1N
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Check point 5.4
20 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Chapter exercises (A) Taking it together
71 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Check point 5.2
15 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
- LAWS OF THERMODYNAMICSBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Subjective
18 Videos
Similar Questions
Two blocks of masses M1andM2 are placed in contact with each other on a frictionless horizontal surface. If a force F is applied on the block of mass M1, what is the contact force between the two blocks?
View SolutionTwo block of masses 3 kg and 1 kg are kept in contact with each other on a frictionless horizontal surface. If a force of 10 N is applied on the larger block what is the acceleration of the system? What is the contact force between the two blocks?
View SolutionTwo blocks of masses 5 kg and 2 kg are kept in contact with each other on a frictionless horizontal surface. If a force of 14 N is applied on the larger block what is the acceleration of the system ? What is the contact force between the two blocks ?
View SolutionTwo blocks of mass = 2 kg and M = 5 kg are in contact on a frictionless table. A horizonatal force F (= 35 N) is applies to m. Find the force of contact between the block, will the force of contact remain same if F is applied to M ?

View SolutionTwo blocks are in contact on a frictionless table. A horizontal force is applied to one block as shown in fig. If m1=10kgandm2=5kgandF=15N. Find the force of contact between the two bodies.

View SolutionTwo blocks of mass 2 kg and 4 kg are kept in contact with each other on a smooth horizontal surface. A horizontal force of 12 N is applied on the first block due to which they move with certain constant acceleration. Calculate the force between the blocks.
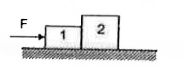
View SolutionTwo blocks of masses M = 3 kg and m = 2 kg are in contact on a horizontal table. A constant horizontal force F = 5 N is applied to block M as shown. There is a constant frictional force of 2 N between the table and the block m but no frictional force between the table and the first block M, then acceleration of the two blocks is
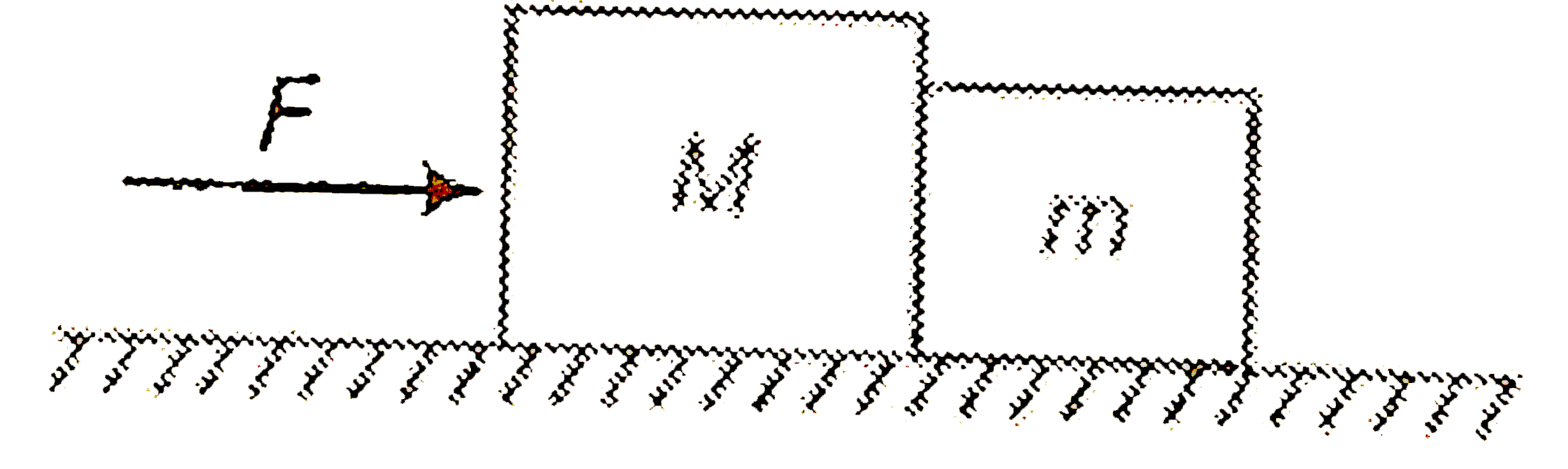
View SolutionThree block of masses m1,m2 and m3 kg are placed in contact with each other on a frictionless table. A force F is applied on the heaviest mass m1, the acceleration of m2 will be
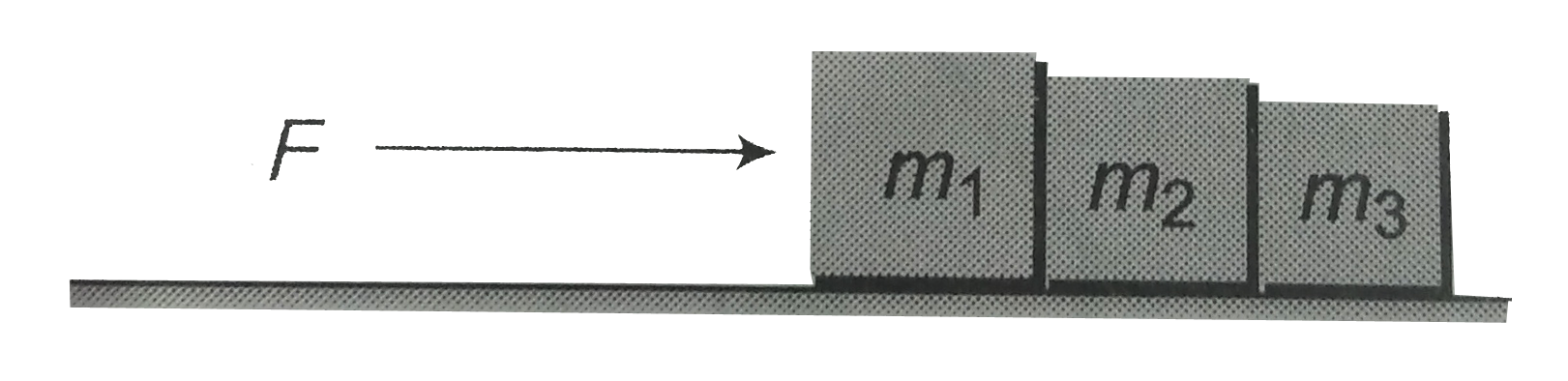
View SolutionTwo blocks of masses 1 kg and 2 kg are placed in contact on a smooth horizontal surface as shown in fig. A horizontal force of 6N is applied d(a) on a 1-kg block. Find the force of interaction of the blocks.

 .
.
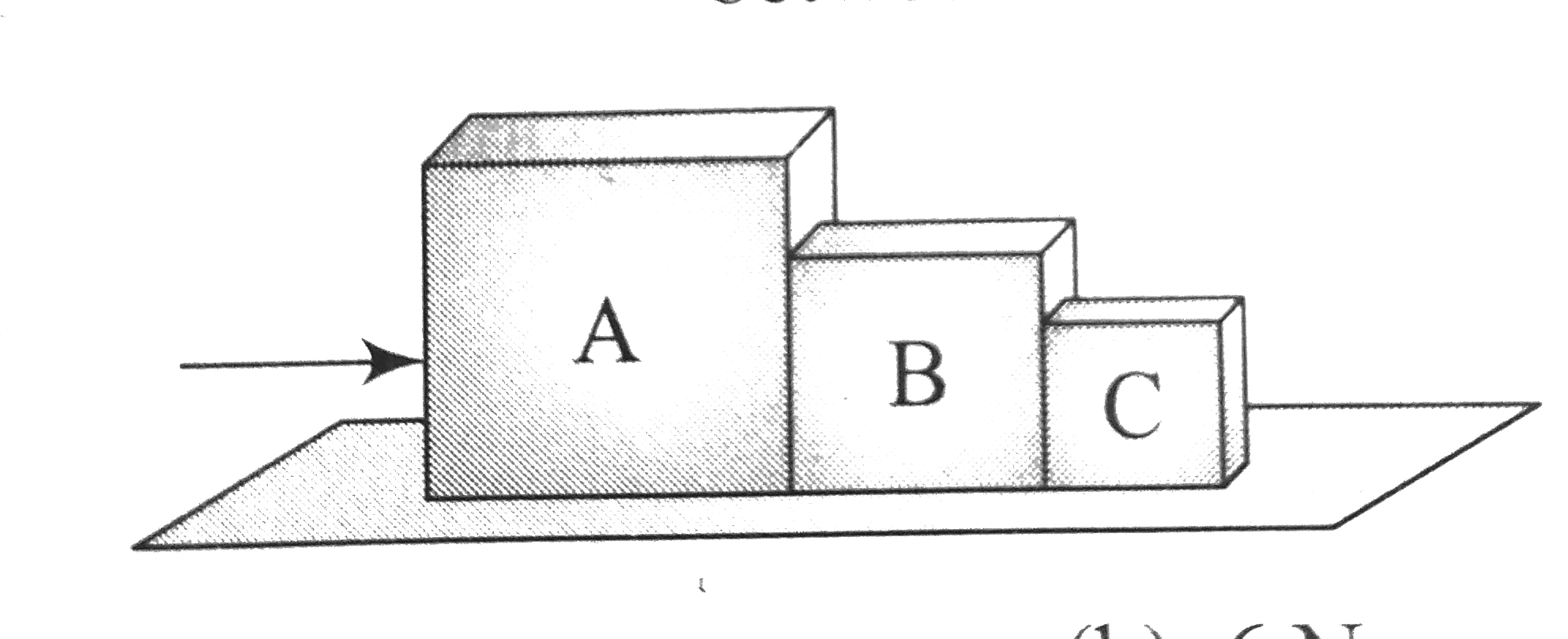
View SolutionThree blocks A , B and C of masses 4kg , 2kg and 1kg respectively are in contact on a frictionless surface, as shown. If a force of 14Nisappliedonthe4kgblock,thenthecontactforcebetweenAandB is.
View Solution
