A string of negligible mass going over a clamped pulley of mass m supports a block of mass M as shown in the figure. The force on the pulley by the clamp is given by
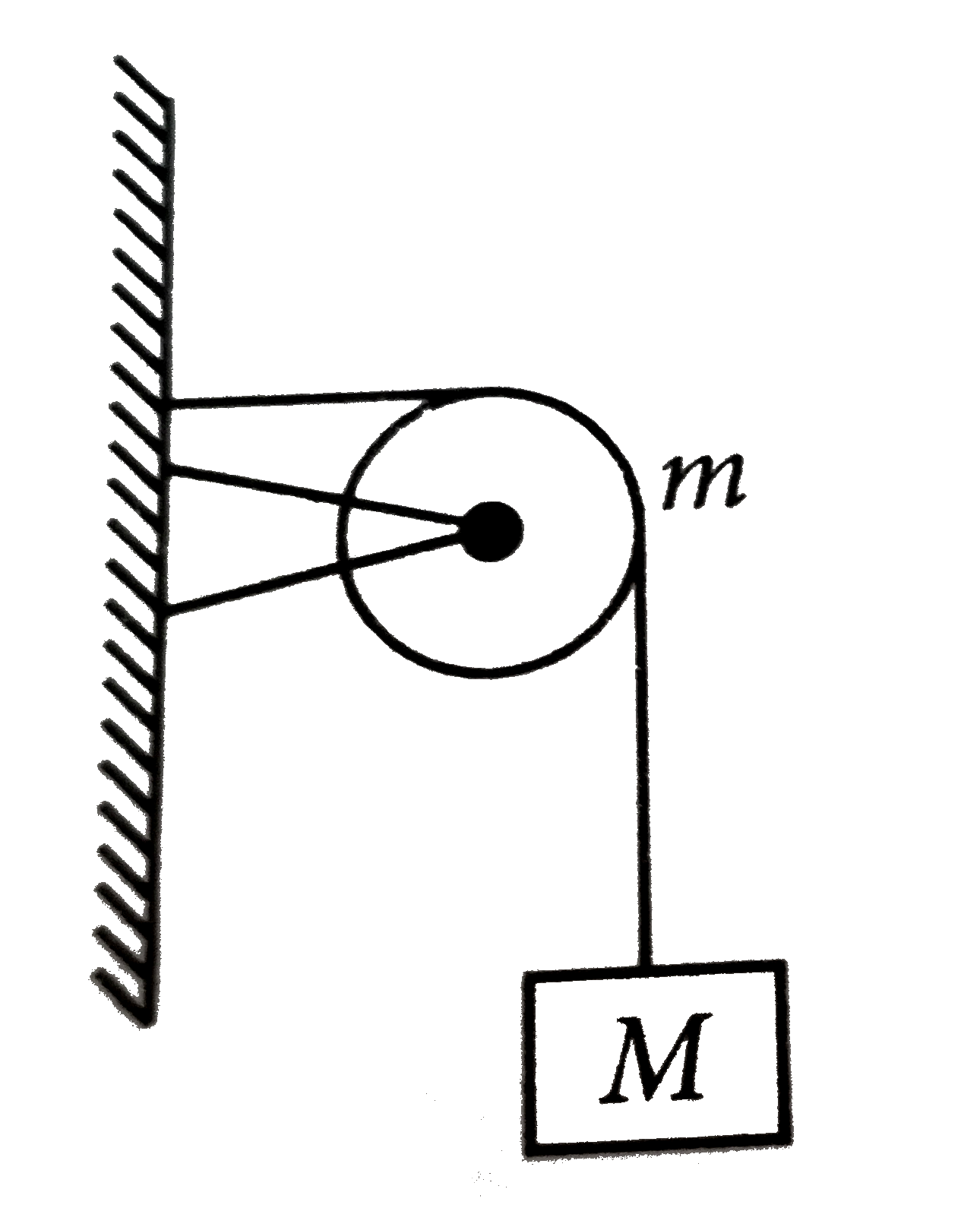
√2Mg
√2mg
g√(M+m)2+m2
g√(M+m)2+M2
The correct Answer is:D
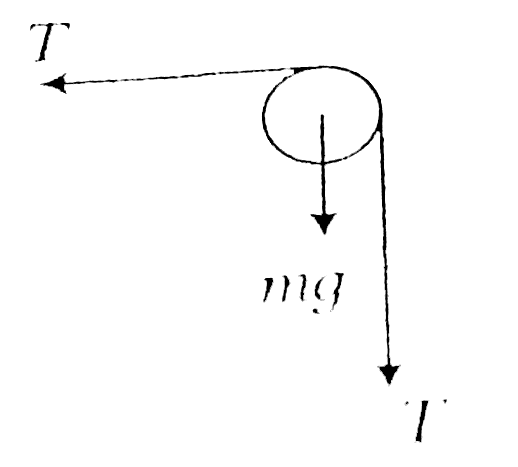
(d) T=mg
Force on the pulley ( other than from clamp)
Fnet=√(T+mg)2+T2
=g√(M+m)2+M2
Since pulley is in equilibrium, clamp will exert the same amount of force in oppsite direction. Or pulley will also exert this much force on clamp.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Check point 5.420videos
LAWS OF MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Chapter exercises (A) Taking it together71videos
LAWS OF MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Check point 5.215videos
LAWS OF MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE15videos
KINEMATICS 1
- BOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Subjective18videos
LAWS OF THERMODYNAMICS
Similar Questions
A spring of negligible mass going over a clamped pulley of mass m supports a block of mass M as shown in the figure. The force on the pulley by the clamp at the time of equilibrium is given by
 View Solution
View SolutionA string of negligible mass passes over a pulley of mass m which is clamped. It supports a block of mass M at its lower free end. What is force exerted on pulley by the support ?
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A string of negligible mass going over a clamped pulley of mass M as shown in Fig. 3.14. The force on pulley by the clamb is given by
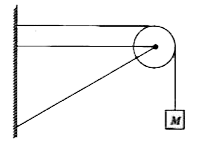 A2 mgB√2 mgC√{(M+m)2+m2} gD√{(M+m)2+M2} g
A2 mgB√2 mgC√{(M+m)2+m2} gD√{(M+m)2+M2} g
A block of mass m hangs by means of a string which goes over a pulley of mass m and moment of inertia l, as shown in the diagram. The string does not realtive to the pulley. Find the frequency of small oscillations.
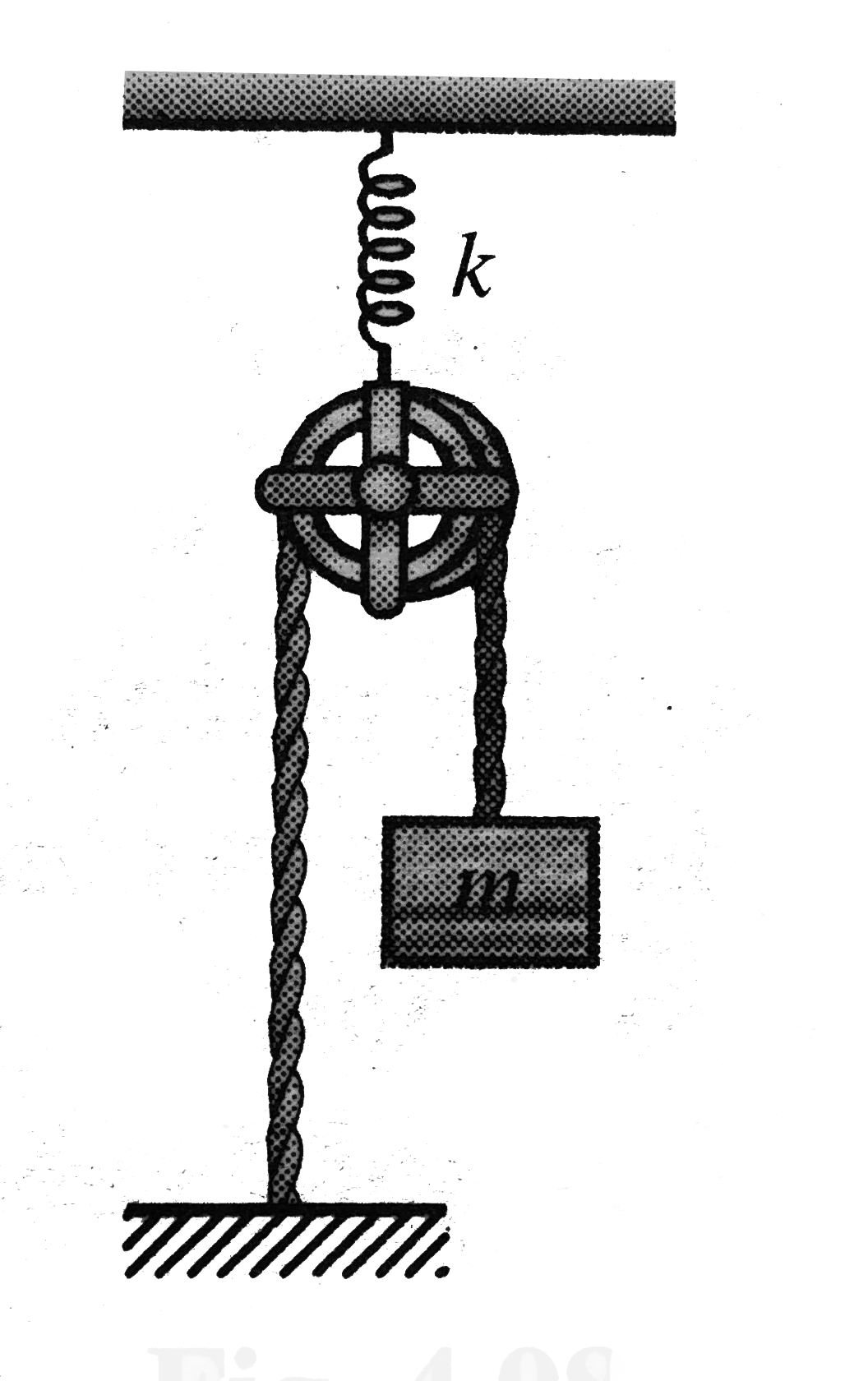 View Solution
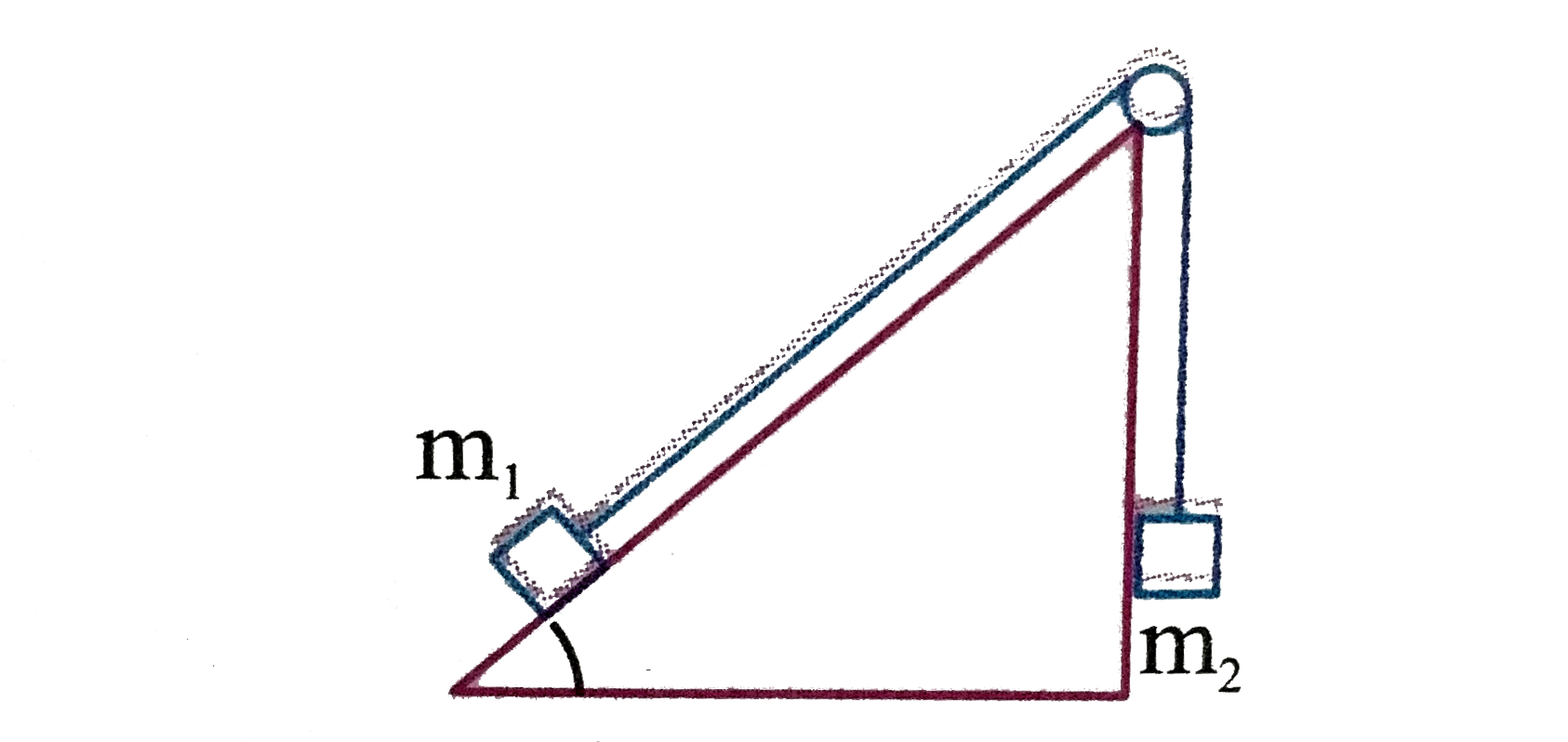
View SolutionTwo blocks of masses m1 and m2 are connected by a string of negligible mass which pass over a frictionless pulley fixed on the top of an inclined plane as shown in figure. The coefficient of friction between m1 and plane is μ.
 View Solution
View SolutionTwo masses m1 and m2 are placed on a smooth horizontal surface and are connected by a string of negligible mass. A horizontal force F is applied on the mass m2 as shown in the figure. The tension in the string is
 View Solution
View SolutionBoth the blocks shown in figure have same mass 'm'. All the pulley and strings are massiess.
 View Solution
View Solutionfind the acceleration of the block of mass M in the situation shown in figure. All the surfaces are frictionless and the pulleys and the string are light.
 View Solution
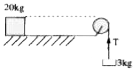
View SolutionA body of mass 20kg is moving on a rough horizontal plane. A block of mass 3kg is connected to the 20 kg mass by a string of negligible mass through a smooth pulley as shown in the figure. The tension in the string is 27 N. The coefficient of kinetic friction between the heavier mass and the surface is (g = 10m/s2)
 View Solution
View SolutionA string is wrapped on a wheel of moment of inertia 0.20 kg-m^2 and radius 10 cm and goes through a light pulley to support a block of mass 2.0 kg as shown in figure. Find the acceleration of the block.
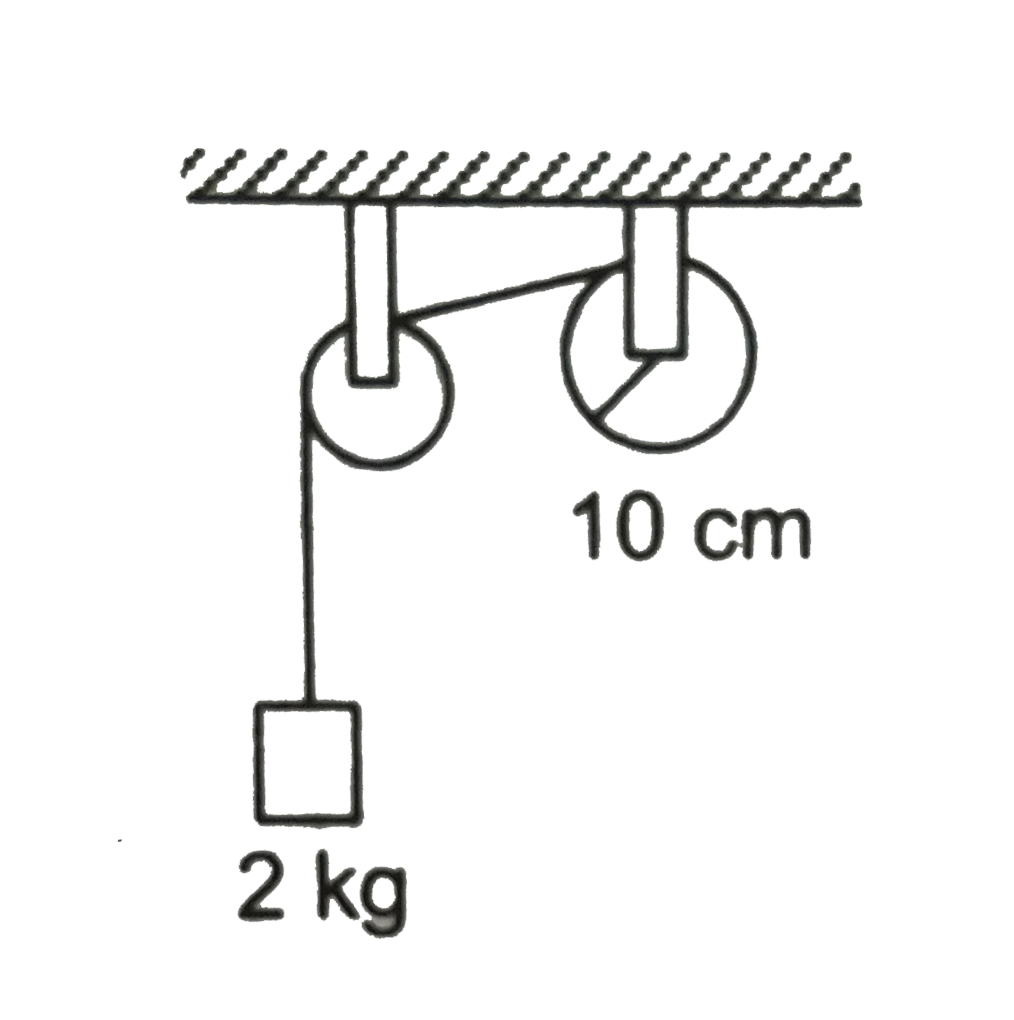 View Solution
View Solution

