These questions consists of two statements each printed as Assertion and Reason. While answering these question you are required to choose any one of the following four responses
Assertion A block of weight 10 N is pushed against a vertical wall by a force of 15 N. The coefficient of friction between the wall and the block is 0.6. Then, the magnitude of maximum frictional force is 9 N.
Reason For given system block will remain stationary.
If both Assertion and reason are true and the reason is a correct explanation of the assertion.
If both Assertion and reason are true and the reason is not the correct explanation of the assertion.
if assertion is true but reason is false
if assertion is false but reason is true
The correct Answer is:C
To solve the problem, we need to analyze the assertion and the reason provided.
Step 1: Understand the Assertion
The assertion states that a block of weight 10 N is pushed against a vertical wall by a force of 15 N. The coefficient of friction between the wall and the block is 0.6. We need to determine if the maximum frictional force is indeed 9 N.
Step 2: Calculate the Normal Force
When the block is pushed against the wall with a force of 15 N, this force creates a normal force acting on the block. The normal force (N) exerted by the wall on the block is equal to the applied force, which is 15 N.
Step 3: Calculate the Maximum Frictional Force
The maximum frictional force (F_friction) can be calculated using the formula:
Ffriction=μ⋅N
where:
- μ = coefficient of friction = 0.6
- N = normal force = 15 N
Substituting the values:
Ffriction=0.6⋅15=9 N
This confirms that the assertion is correct.
Step 4: Analyze the Reason
The reason states that the block will remain stationary. To determine if this is true, we need to analyze the forces acting on the block.
Step 5: Identify the Forces Acting on the Block
1. The weight of the block (W) acting downwards = 10 N
2. The maximum frictional force acting upwards = 9 N
Step 6: Calculate the Net Force
To find out if the block will remain stationary, we calculate the net force (F_net) acting on the block:
Fnet=W−Ffriction
Fnet=10 N−9 N=1 N
Since there is a net force of 1 N acting downwards, the block will not remain stationary; it will accelerate downwards.
Conclusion
- The assertion is true: The maximum frictional force is indeed 9 N.
- The reason is false: The block will not remain stationary due to the net downward force.
Final Answer
The correct option is C: Assertion is true, Reason is false.
---
The assertion states that a block of weight 10 N is pushed against a vertical wall by a force of 15 N. The coefficient of friction between the wall and the block is 0.6. We need to determine if the maximum frictional force is indeed 9 N.
When the block is pushed against the wall with a force of 15 N, this force creates a normal force acting on the block. The normal force (N) exerted by the wall on the block is equal to the applied force, which is 15 N.
The maximum frictional force (F_friction) can be calculated using the formula:
Ffriction=μ⋅N
where:
- μ = coefficient of friction = 0.6
- N = normal force = 15 N
Ffriction=0.6⋅15=9 N
The reason states that the block will remain stationary. To determine if this is true, we need to analyze the forces acting on the block.
1. The weight of the block (W) acting downwards = 10 N
2. The maximum frictional force acting upwards = 9 N
To find out if the block will remain stationary, we calculate the net force (F_net) acting on the block:
Fnet=W−Ffriction
Fnet=10 N−9 N=1 N
- The assertion is true: The maximum frictional force is indeed 9 N.
- The reason is false: The block will not remain stationary due to the net downward force.
The correct option is C: Assertion is true, Reason is false.
Similar Questions
A block of weight 5 N is pushed against a vertical wall by a force 12 N . The coefficient of friction between the wall and block is 0.6 . Find the magnitude of the force exerted by the wall on the block :
View SolutionThese questions consists of two statements each printed as Assertion and Reason. While answering these question you are required to choose any one of the following five responses.
Reason: In an eleastic collision, the linear momentum of the system is conserved.
View SolutionThese questions consists of two statements each printed as Assertion and Reason. While answering these question you are required to choose any one of the following four responses
Assertion The weighing machine measures the weight of a body.
Reason Weightlessness means the absence of weight.
View SolutionThese questions consists of two statements each printed as Assertion and Reason. While answering these question you are required to choose any one of the following four responses
Assertion Friction opposes motion of a body.
Reason Static friction is self adjusting while kinetic friction is constant.
View SolutionThese questions consist of two statements each, printed as
Assertion and Reason. While answering these questions you are
required to choose any one of the following four responses:
Assertion: [Co(NO2)3(NH3)3] does not show optical isomerism.
Reason: It has a plane of symmetry.
View SolutionThese questions consists of two statements each printed as Assertion and Reason. While answering these question you are required to choose any one of the following four responses
Assertion In the shown figure, tension T connected to the ceiling is 10N<T<20N
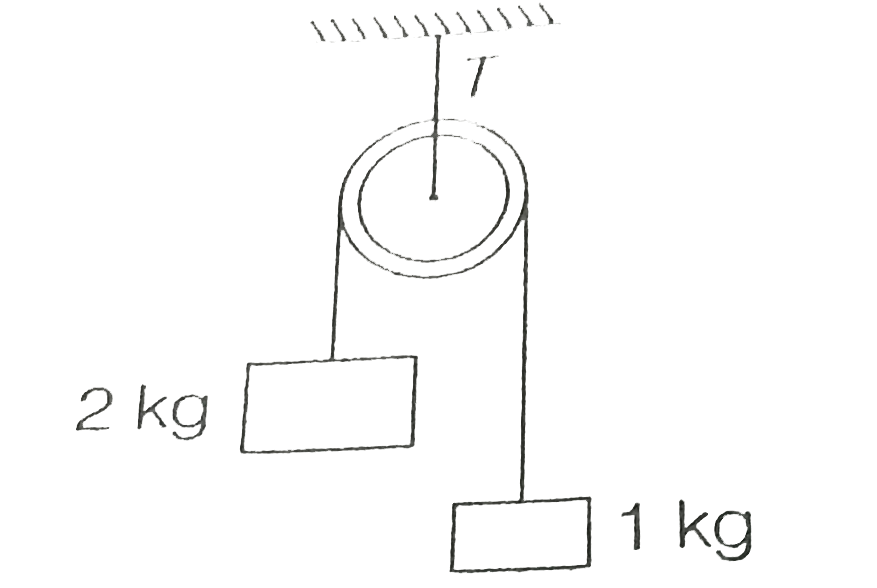
Reason System is not stationary.
View SolutionThe question consist of two statements each, printed as Assertion and Reason. While answering these questions you are required to choose any one of the following four responses:
Assertion: Charge on the complex of ferric ion with EDTA is minus one.
Reason: EDTA is a hexadentate ligand.
View SolutionThese question consists of two statements each printed as Assertion and Reason. While answering these question you are required to choose any one of the following five responses.
Assertion: Emissive power of a perfectly black body is one.
Reason: Absorptive power of perfectly black body is one.
View SolutionThese questions consist of two statements each, printed as Assertion and Reason. While answering these questions you are required to choose any one of the following five responses.
Assertion: Specialization of cells is useful for organism.
Reason: It increases the operational effciency of an organism.
View SolutionThese questions consists of two statements each printed as Assertion and Reason. While answering these question you are required to choose any one of the following four responses
Assertion Static friction acting on a body is always greater than the kinetic friction acting on this body.
Reason Coefficient of static friction is more than the coefficient of kinetic friction.
View Solution
