Two springs with spring constants K1=1500N/mand m K2=3000N/m are stretched by the same force. The ratio of potential energy stored in spring will be
To solve the problem of finding the ratio of potential energy stored in two springs with different spring constants when stretched by the same force, we can follow these steps:
1. Identify the Spring Constants:
- Given: K1=1500N/m and K2=3000N/m.
2. Understand the Formula for Potential Energy:
- The potential energy U stored in a spring is given by the formula:
U=12Kx2
- Where K is the spring constant and x is the displacement from the equilibrium position.
3. Relate Force and Displacement:
- The force F applied to stretch the spring is related to the displacement x by Hooke's Law:
F=Kx
- Rearranging gives us:
x=FK
4. Substitute Displacement into the Potential Energy Formula:
- We can substitute x back into the potential energy formula:
U=12K(FK)2
- This simplifies to:
U=12K⋅F2K2=F22K
5. Calculate the Potential Energy for Each Spring:
- For spring 1:
U1=F22K1
- For spring 2:
U2=F22K2
6. Find the Ratio of Potential Energies:
- The ratio of potential energies U1U2 is given by:
U1U2=F22K1F22K2=K2K1
- Since F2 and 2 cancel out.
7. Substitute the Values of Spring Constants:
- Now substitute the values:
U1U2=K2K1=30001500=2
8. Final Result:
- The ratio of potential energy stored in spring 1 to spring 2 is:
U1:U2=2:1
Final Answer:
The ratio of potential energy stored in the two springs is 2:1.
- Given: K1=1500N/m and K2=3000N/m.
- The potential energy U stored in a spring is given by the formula:
U=12Kx2
- Where K is the spring constant and x is the displacement from the equilibrium position.
- The force F applied to stretch the spring is related to the displacement x by Hooke's Law:
F=Kx
- Rearranging gives us:
x=FK
- We can substitute x back into the potential energy formula:
U=12K(FK)2
- This simplifies to:
U=12K⋅F2K2=F22K
- For spring 1:
U1=F22K1
- For spring 2:
U2=F22K2
- The ratio of potential energies U1U2 is given by:
U1U2=F22K1F22K2=K2K1
- Since F2 and 2 cancel out.
- Now substitute the values:
U1U2=K2K1=30001500=2
- The ratio of potential energy stored in spring 1 to spring 2 is:
U1:U2=2:1
The ratio of potential energy stored in the two springs is 2:1.
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - CHECK POINT 6.1
20 Videos
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - CHECK POINT 6.2
20 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based
2 Videos
- WORK, POWER AND ENERGYBOOK - DC PANDEY ENGLISHCHAPTER - WORK, POWER AND ENERGYEXERCISE - E Integer Type Questions
11 Videos
Similar Questions
Knowledge Check
Two springs of spring constants 1000Nm−1 and 2000Nm−1 are stretched with same force. They will have potential energy in the ratio of
A2:1B22:12C1:2D12:22
Two springs have force constants kA such that kB=2kA. The four ends of the springs are stretched by the same force. If energy stored in spring A is E, then energy stored in spring B is
View SolutionTwo springs have spring constants k1andk2(k1≠k2). Both are extended by same force. If their elastic potential energical are U1andU2, then U1:U2 is
View SolutionTwo springs have spring constants k1andk2(k1≠k2). Both are extended by same force. If their elastic potential energical are U1andU2, then U2 is
View SolutionForce constants K1andK2 of two springs are in the ratio 5:4. They are stretched by same length. If potential energy stored in one spring is 25 J then potential energy stored in second spring is
View SolutionA 5 kg block in connected with two spring A and B having force constant, K1=200Nm and K2=300Nm respectively as shown in the figure . The block is released from rest with both the springs in natural length. The maximum energy stored in the spring A will be
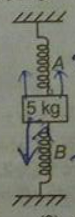
View SolutionTwo springs A and B (KA=2KB) are stretched by same suspended weights then ratio of workdone in stretching is -
View SolutionA force of 10 N holds an ideal spring with a 20Nm spring constant in compression. The potential energy stored in the spring is
View Solution
