A rod of mass and length is lying on a horizontal table. Work done in making it stand on one end will be
mgl
2mgl
The correct Answer is:B
To find the work done in making a rod of mass m and length l stand on one end, we can follow these steps:
Step 1: Understand the Problem
We have a rod lying horizontally on a table, and we want to calculate the work done to lift it to a vertical position.
Step 2: Identify Initial Potential Energy
When the rod is lying horizontally, the center of mass is at its midpoint. Since the height (h) of the center of mass from the ground is zero in this position, the initial potential energy (PE_initial) can be calculated using the formula:
PEinitial=mgh
Here, h=0:
PEinitial=mg⋅0=0
Step 3: Identify Final Potential Energy
When the rod is standing vertically on one end, the center of mass is at a height of l2 (half the length of the rod). The final potential energy (PE_final) can be calculated as:
PEfinal=mgh
Here, h=l2:
PEfinal=mg⋅l2=mgl2
Step 4: Calculate Work Done
The work done (W) in lifting the rod can be found by calculating the change in potential energy:
W=PEfinal−PEinitial
Substituting the values we found:
W=mgl2−0=mgl2
Conclusion
The work done in making the rod stand on one end is:
W=mgl2
We have a rod lying horizontally on a table, and we want to calculate the work done to lift it to a vertical position.
When the rod is lying horizontally, the center of mass is at its midpoint. Since the height (h) of the center of mass from the ground is zero in this position, the initial potential energy (PE_initial) can be calculated using the formula:
PEinitial=mgh
Here, h=0:
PEinitial=mg⋅0=0
When the rod is standing vertically on one end, the center of mass is at a height of l2 (half the length of the rod). The final potential energy (PE_final) can be calculated as:
PEfinal=mgh
Here, h=l2:
PEfinal=mg⋅l2=mgl2
The work done (W) in lifting the rod can be found by calculating the change in potential energy:
W=PEfinal−PEinitial
Substituting the values we found:
W=mgl2−0=mgl2
The work done in making the rod stand on one end is:
W=mgl2
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - MEDICAL ENTRANCE SPECIAL QUESTIONS16videos
WORK, ENERGY AND POWER
- BOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - MATCH THE COLUMNS7videos
WORK, ENERGY AND POWER
- BOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - CHECK POINT 6.310videos
WORK, ENERGY AND POWER
- BOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based2videos
WORK, ENERGY & POWER
- BOOK - DC PANDEY ENGLISHCHAPTER - WORK, POWER AND ENERGYEXERCISE - E Integer Type Questions11videos
WORK, POWER AND ENERGY
Similar Questions
A uniform chain of mass m and length l is lying on a horizontal table with one-third of its length hanging over the edge of the table.

What is the speed of the chain, when it just loses contact with the table?View SolutionA uniform chain of mass m and length l is lying on a horizontal table with one-third of its length hanging over the edge of the table.

If the chain is limiting equlibrium, what is the coefficient of friction for the contact between table and chain?View SolutionA uniform chain of mass m and length l is lying on a horizontal table with one-third of its length hanging over the edge of the table.

If a gentle push is given to hanging end, it will start moving down. What is the change in gravitational potential energy of the chain, as it just leaves the table?View SolutionA unifrom rod of mass m and length L is kept on a horizontal table with L4 length on the table. The end B is tied to a string as shown in the figure. The string attached to the end B is cut and the rod starts rotating about point C. Find the normal reaction from the table on the earth as soon as the string is cut.
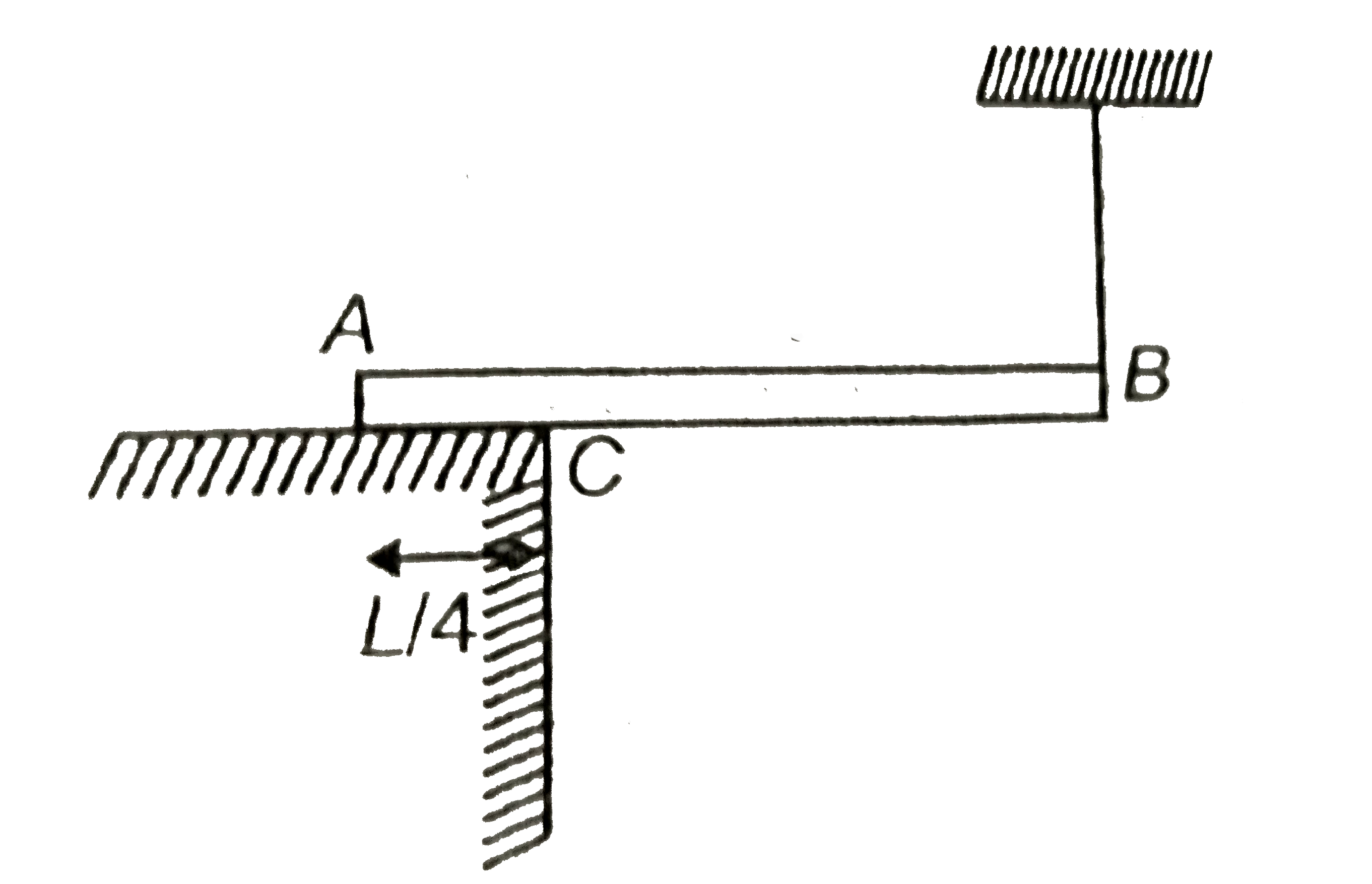 View Solution
View SolutionA uniform rod of mass m and length L is kept on a horizontal table with L4 length on the table as shown in figure. As the string attached to the end B is cut, the angular acceleration of the rod about point C will be
_PHY_E05_242_Q01.png) View Solution
View SolutionA uniform thin rod of mass m and length l is standing on a smooth horizontal suface. A slight disturbance causes the lower end to slip on the smooth surface and the rod starts falling. Find the velocity of centre of mass of the rod at the instant when it makes an angle θ with horizontal.
View SolutionA uniform rod of mass m and length l is lying on a smooth table. An impulse J acts on the rod momentarily as shown in figure at point R. Just after that:

 View Solution
View SolutionA thin uniform rod AB of mass m and length L is placed on a smooth horizontal table. A constant horizontal force of magnitude F starts acting on the rod at one of the ends AB, Initially, the force is perpendicular to the length of the rod. Taking the moment at which force starts acting as t=0, find.
(a) Distance moved by centre of mass of the rod in time t0.
(b) Magnitude of initial acceleration of the end A of the rod.View SolutionA uniform rod AB of mass m and length l is at rest on a smooth horizontal surface. An impulse J is applied to the end B, perpendicular to the rod in the horizontal direction. Speed of particlem P at a distance l6 from the centre towards A of the rod after time t=πml12J is.
View SolutionA slender rod of mass m and length L is pivoted about a horizontal axis through one end and released from rest at an angle of 30∘ above the horizontal. The force exerted by the pivot on the rod at the instant when the rod passes through a horizontal position is
 View Solution
View Solution

