Calculate the work done in streching a steel wire of Young's modulus of 2×1011Nm−2 mass of 40kg,length of 200 cm and area of cross-section is 0.06 cm2 slowly applied without the elastic limit being reached.
To calculate the work done in stretching a steel wire, we will follow these steps:
Step 1: Convert all measurements to SI units
- Length of the wire: L=200cm=200×10−2m=2m
- Area of cross-section: A=0.06cm2=0.06×10−4m2=6×10−6m2
- Mass of the wire: m=40kg
Step 2: Calculate the force applied on the wire
The force F due to the mass is given by:
F=m⋅g
where g (acceleration due to gravity) is approximately 9.8m/s2.
F=40kg×9.8m/s2=392N
Step 3: Use Young's modulus to find the extension ΔL
Young's modulus Y is defined as:
Y=StressStrain=F/AΔL/L
Rearranging this gives:
ΔL=F⋅LA⋅Y
Substituting the known values:
- F=392N
- L=2m
- A=6×10−6m2
- Y=2×1011N/m2
ΔL=392N⋅2m6×10−6m2⋅2×1011N/m2
Calculating ΔL:
ΔL=78412×105=7841200000≈0.00065333m≈0.65333mm
Step 4: Calculate the work done in stretching the wire
The work done W in stretching the wire is given by:
W=12⋅F⋅ΔL
Substituting the values:
W=12⋅392N⋅0.00065333m
Calculating W:
W=0.128J
Final Answer
The work done in stretching the steel wire is approximately 0.128J.
---
- Length of the wire: L=200cm=200×10−2m=2m
- Area of cross-section: A=0.06cm2=0.06×10−4m2=6×10−6m2
- Mass of the wire: m=40kg
The force F due to the mass is given by:
F=m⋅g
where g (acceleration due to gravity) is approximately 9.8m/s2.
F=40kg×9.8m/s2=392N
Young's modulus Y is defined as:
Y=StressStrain=F/AΔL/L
Rearranging this gives:
ΔL=F⋅LA⋅Y
Substituting the known values:
- F=392N
- L=2m
- A=6×10−6m2
- Y=2×1011N/m2
ΔL=78412×105=7841200000≈0.00065333m≈0.65333mm
The work done W in stretching the wire is given by:
W=12⋅F⋅ΔL
Substituting the values:
W=12⋅392N⋅0.00065333m
Calculating W:
W=0.128J
The work done in stretching the steel wire is approximately 0.128J.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - ELASTICITYEXERCISE - Solved Examples6videos
ELASTICITY
- BOOK - DC PANDEY ENGLISHCHAPTER - ELASTICITYEXERCISE - MiscellaneousExamples4videos
ELASTICITY
- BOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - All Questions469videos
CURRENT ELECTRICITY
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Integer17videos
ELECTROSTATICS
Similar Questions
Knowledge Check
- Question 1 - Select One
The area of a cross-section of steel wire is 0.1cm−2 and Young's modulus of steel is 2x1011Nm−2. The force required to stretch by 0.1% of its length is
A1000 NB2000 NCÂ 4000 ND5000N - Question 2 - Select One
The self inductance of a solenoid that has a cross-sectional area of 1cm2, a length of 10 cm and 1000 turns of wire is
A0.86mHB1.06mHC1.26mHD1.46mH
Young 's modulus of steel is 2.0×1011Nm/(2). Express it is dynecm2.
View SolutionThe elastic limit of steel is 8×108Nm−2 and its Young modulus 2×1011Nm−2. Find the maximum elongation of a half meter steel wire that can be given without exceeding the elastic limit.
View SolutionFigure shows the graph of elastic potential energy (U) stored versus extension, for a steel wire (Y=2×1011Pa) of volume 200cc. If area of cross- section A and original length L, then
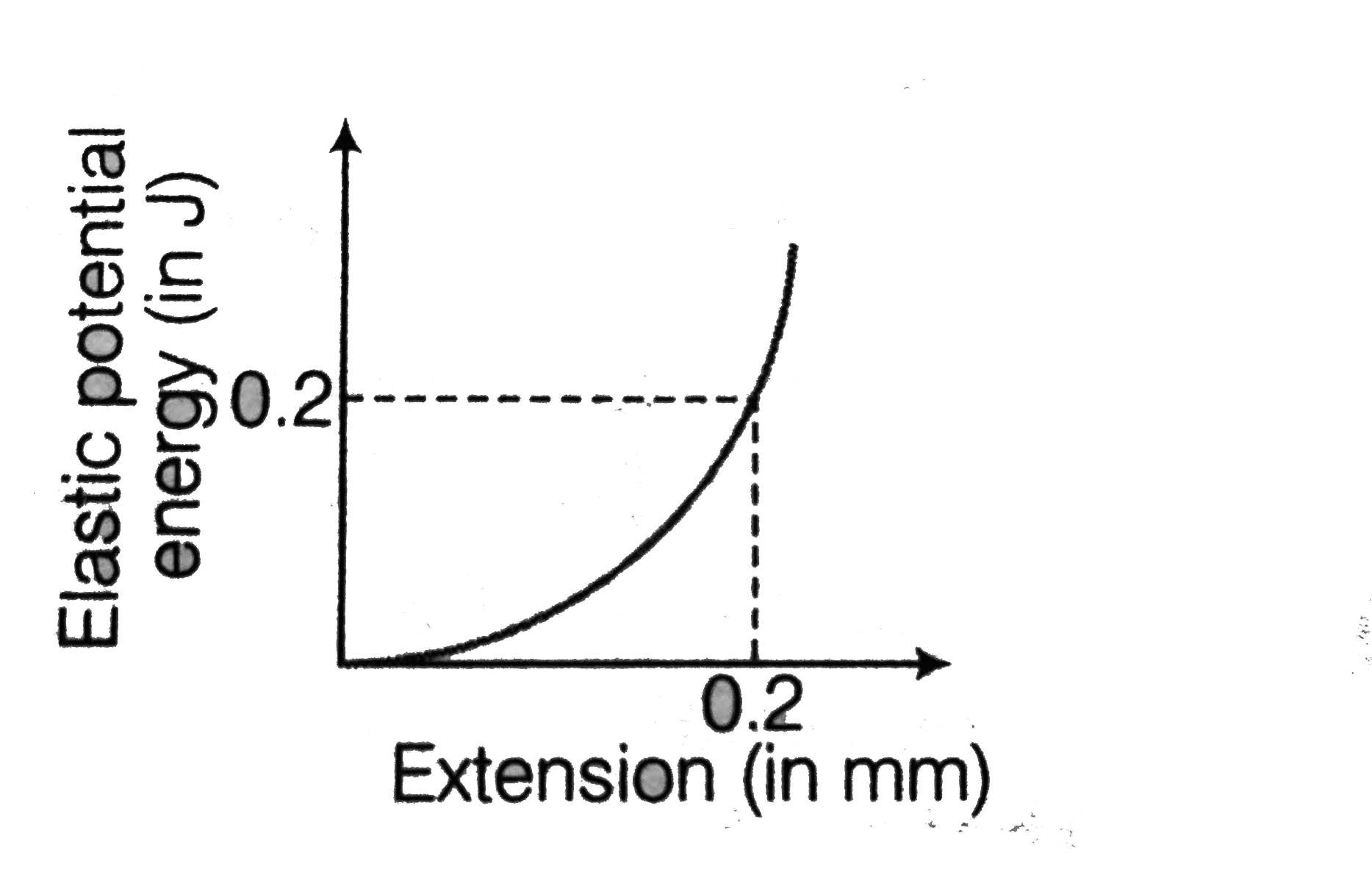 View Solution
View SolutionCalculate the force required to incrase the length of wire of cross-sectional area 10−6m2 by 50% if the Young's modulus of the material of the wire is 90×109Pa.
View SolutionCalculate the stress in a tight wire of a material whose Young's modulus is 19.6×1011dynecm2 so that the speed of the longitudinal waves is 10 times the speed of transverse waves.
View SolutionCalculate the sterss in a tight wire of a material whose Young's modulus is 19.6×1011dynecm2 so that the speed of the longitudinal waves is 10 times the speed of transverse waves.
View Solution

