Assertion Upto the elastic limit, strain ∝ stress.
Reason Upto elastic limit, material returns to its original shape and size, when external force is removed.
If both Assertion and Reason are true and Reason is the correct explanation of Assertion.
If both Assertion and Reason are true but Reason is not the correct explanation of Assertion.
If Assertion is true but Reason is false.
If both Assertion and Reason are false.
The correct Answer is:D
To analyze the given assertion and reason, we will break down the concepts of elasticity, stress, strain, and the definitions of the proportional limit and elastic limit.
1. Understanding the Assertion:
- The assertion states that "Up to the elastic limit, strain is proportional to stress."
- According to Hooke's Law, this is true only up to the proportional limit, not the elastic limit. Beyond the proportional limit, the relationship may not hold true.
2. Understanding the Reason:
- The reason states that "Up to the elastic limit, the material returns to its original shape and size when the external force is removed."
- This statement is partially correct. While materials do return to their original shape and size within the elastic limit, the elastic limit is the maximum extent to which a material can be deformed elastically. Beyond this point, some permanent deformation may occur.
3. Identifying the Proportional Limit and Elastic Limit:
- The proportional limit is the point on the stress-strain curve where stress is directly proportional to strain (Hooke's Law applies).
- The elastic limit is the maximum stress that a material can withstand without permanent deformation. Beyond this point, the material may not return to its original shape.
4. Conclusion:
- Since the assertion incorrectly states that strain is proportional to stress up to the elastic limit (it is only true up to the proportional limit), the assertion is false.
- The reason is also misleading because while materials return to their original shape within the elastic limit, the statement implies that this is true up to the elastic limit without acknowledging the proportional limit distinction.
5. Final Answer:
- Both the assertion and the reason are false. Therefore, the correct conclusion is that both statements are incorrect.
- The assertion states that "Up to the elastic limit, strain is proportional to stress."
- According to Hooke's Law, this is true only up to the proportional limit, not the elastic limit. Beyond the proportional limit, the relationship may not hold true.
- The reason states that "Up to the elastic limit, the material returns to its original shape and size when the external force is removed."
- This statement is partially correct. While materials do return to their original shape and size within the elastic limit, the elastic limit is the maximum extent to which a material can be deformed elastically. Beyond this point, some permanent deformation may occur.
- The proportional limit is the point on the stress-strain curve where stress is directly proportional to strain (Hooke's Law applies).
- The elastic limit is the maximum stress that a material can withstand without permanent deformation. Beyond this point, the material may not return to its original shape.
- Since the assertion incorrectly states that strain is proportional to stress up to the elastic limit (it is only true up to the proportional limit), the assertion is false.
- The reason is also misleading because while materials return to their original shape within the elastic limit, the statement implies that this is true up to the elastic limit without acknowledging the proportional limit distinction.
- Both the assertion and the reason are false. Therefore, the correct conclusion is that both statements are incorrect.
Similar Questions
Figure shows the relationship between tensile stress and strain for a typical material. Below proportional point A, stress is directly proportional to strain which means Young's moudulus (Y) is a constant. In this region the material obeys Hooke's law.

Provided the strain is below the yield point 'B' the material returns to its original shape and size when the force is removed. Beyond the yield point, the material retains a permancnt deformation after the stress is removed. For stresses beyond the yeld point, the material exhibit plastic flow, which means that it continues to elongate for little increases in the stress. Beyond C a local constriction occurs. The material fractures at D (i.e. breaking point). The graph below shows the stress-strain curve for 4 different materials.

If you bough a new shoe which bites in the beginning and later on fits perfectly, then the material used to making the shoe is
View SolutionFigure shows the relationship between tensile stress and strain for a typical material. Below proportional point A, stress is directly proportional to strain which means Young's moudulus (Y) is a constant. In this region the material obeys Hooke's law.

Provided the strain is below the yield point 'B' the material returns to its original shape and size when the force is removed. Beyond the yield point, the material retains a permancnt deformation after the stress is removed. For stresses beyond the yeld point, the material exhibit plastic flow, which means that it continues to elongate for little increases in the stress. Beyond C a local constriction occurs. The material fractures at D (i.e. breaking point). The graph below shows the stress-strain curve for 4 different materials.

If you bough a new shoe which bites in the beginning and later on fits perfectly, then the material used to making the shoe is
View Solution
Knowledge Check
Assertion : The elastic spring force arises due to the net attraction or repulsion between the neighbouring atoms of the spring when it is elongated of compressed.
Reason : The laws of derived forces such as spring force, friction force are independent of the laws of fundamental forces in nature.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true but reason is false.DIf both assertion and reason are false.
Figure shows the relationship between tensile stress and strain for a typical material. Below proportional point A, stress is directly proportional to strain which means Young's moudulus (Y) is a constant. In this region the material obeys Hooke's law.

Provided the strain is below the yield point 'B' the material returns to its original shape and size when the force is removed. Beyond the yield point, the material retains a permancnt deformation after the stress is removed. For stresses beyond the yeld point, the material exhibit plastic flow, which means that it continues to elongate for little increases in the stress. Beyond C a local constriction occurs. The material fractures at D (i.e. breaking point). The graph below shows the stress-strain curve for 4 different materials.
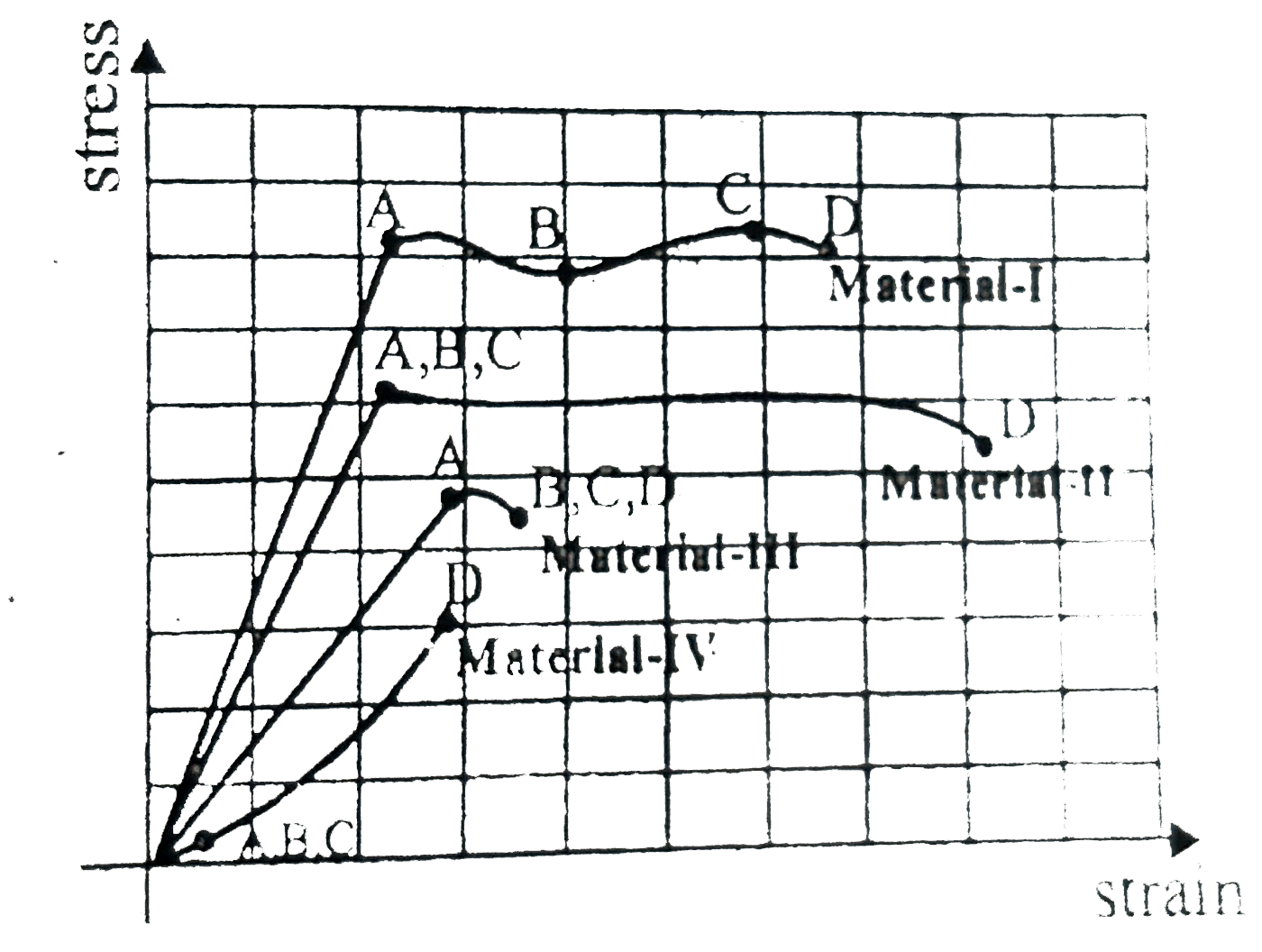
Material which is good for making wires by stretching is
View SolutionFigure shows the relationship between tensile stress and strain for a typical material. Below proportional point A, stress is directly proportional to strain which means Young's moudulus (Y) is a constant. In this region the material obeys Hooke's law.

Provided the strain is below the yield point 'B' the material returns to its original shape and size when the force is removed. Beyond the yield point, the material retains a permancnt deformation after the stress is removed. For stresses beyond the yeld point, the material exhibit plastic flow, which means that it continues to elongate for little increases in the stress. Beyond C a local constriction occurs. The material fractures at D (i.e. breaking point). The graph below shows the stress-strain curve for 4 different materials.

If you bough a new shoe which bites in the beginning and later on fits perfectly, then the material used to making the shoe is
View SolutionAssertion Upto elastic limit of a stress-strain curve, the steel wire tends to regain its original shape when stress is removed.
Reason Within elastic limit, the wire follows Hooke's law.
View SolutionAssertion : steel is more elastic than rubber.
Reason : For same strain , steel requires more stress to be produced in it.
View SolutionThe stress strain graph for a metal wire is shown in the fig. Up to the point E, the wire returns to its original state O along the curve EPO, when it is gradually unloaded. Point B corresponds to the fracture of the wire.
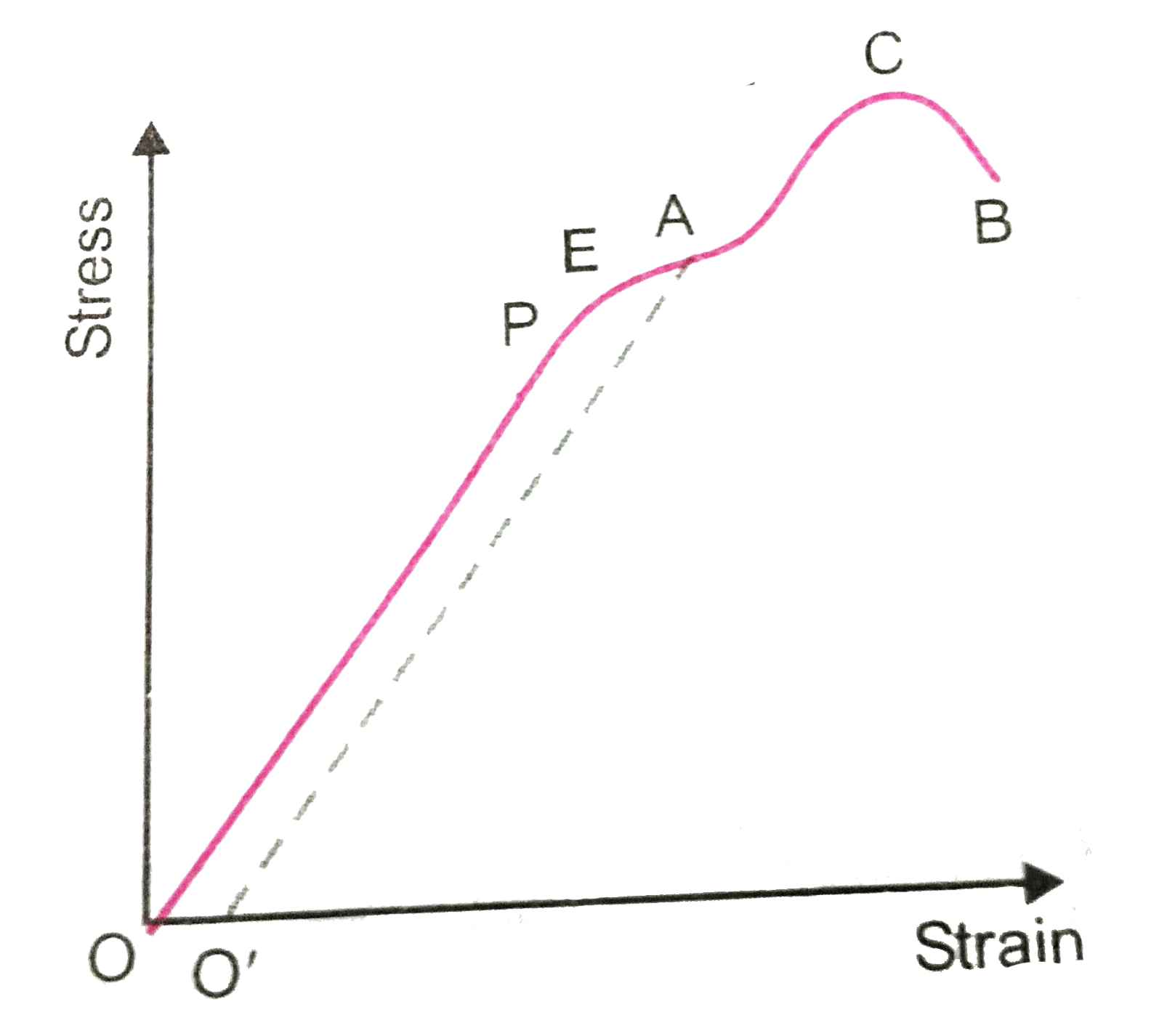
(a) Up to what point on the curve is Hooke's law obeyed? (this point some times called proportional limits).
(b) Which point on the curve corresponding to elastic limit or yield point of the wire?
(c ) Indicate the elastic and plastic regions of the stress-strain graph.
(d) Describe what happens when the wire is loaded up to a stress corresponding to the point A on the graph and then unloaded gradually. In particular explain the dotted curve.
(e) What is peculiar about the portion of the stress- strain graph from C to B? Up to what stress can the wire be subjected without causing fracture?
View SolutionWithin the elastic limit, stress is directly proportional to strain produced in a body is the statement of
View SolutionA ball of density d is dropped on to a horizontal solid surface. It bounces elastically from the surface and returns to its original position in a time t1. Next, the ball is released and it falls through the same height before striking the surface of a liquid of density of dL
(a) If d<dL, obtain an expression (in terms of d, t1 and dL) for the time t2 the ball takes to come back to the position from which it was released.
(b) Is the motion of the ball simple harmonic?
(c) If d=dL, how does the speed of the ball depend on its depth inside the liquid? Neglect all frictional and other dissipative forces. Assume the depth of the liquid to be large.
View Solution
