A tank is filled to a height H. The range of water coming out of a hole which is a depth from the surface of water level is
The correct Answer is:B
To solve the problem of finding the range of water coming out of a hole located at a depth of H4 from the surface of the water in a tank filled to a height H, we can follow these steps:
1. Identify the Depth of the Hole:
The hole is located at a depth of h=H4 from the surface of the water.
2. Determine the Height of Water Above the Hole:
The height of water above the hole is given by:
H−h=H−H4=3H4
3. Use the Formula for Range:
The range R of the water jet coming out of the hole can be calculated using the formula:
R=2√h(H−h)
Substituting h=H4 and H−h=3H4:
R=2√H4⋅3H4
4. Simplify the Expression:
Now, simplify the expression:
R=2√3H216=2⋅√3H4=√3H2
5. Final Result:
Therefore, the range of the water coming out of the hole is:
R=√3H2
The hole is located at a depth of h=H4 from the surface of the water.
The height of water above the hole is given by:
H−h=H−H4=3H4
The range R of the water jet coming out of the hole can be calculated using the formula:
R=2√h(H−h)
Substituting h=H4 and H−h=3H4:
R=2√H4⋅3H4
Now, simplify the expression:
R=2√3H216=2⋅√3H4=√3H2
Therefore, the range of the water coming out of the hole is:
R=√3H2
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Check point 13.310videos
FLUID MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Check point 13.410videos
FLUID MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Check point 13.115videos
FLUID MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - EXPERIMENTSEXERCISE - Subjective15videos
EXPERIMENTS
- BOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE2videos
GENERAL PHYSICS
Similar Questions
A tank is filled with water upto a height H. A hole is punched in one of the walls at a depth h1 below the water surface. (a) Find the distance from the foot of the wall at which the stream strikes the floor. (6) Is it possible to make second hole at another depth so that this second stream also has the same range? If so find its depth ?
View SolutionIn a pond of water, a flame is held 2 m above the surface of the water. A fish is at depth of 4 m from the water surface. Refractive index of water is 43. The apparent height of the flame from the eyes of fish is -
View Solution
Knowledge Check
- Question 1 - Select One
The height of the water in a tank is H. The range of the liquid emerging out from a hole in the wall of the tank at a depth 3H4 from the upper surface of water, will be
AHBH2C3H2D√3H2 - Question 2 - Select One
A large open tank has two holes in the wall. One is square hole of side L at a depth y from the top and the other is a circular hole of radius R at a depth of 4y from the top. When the tank is completely filled with water, the quantities of water flowing out per second from both holes are the same. Then R is equal to
AL/√(2π)B2πLCLDL/2π - Question 3 - Select One
A point source of light is placed at a depth of h below the surface of water of refractive index μ. A floating opaque disc is placed on the surface of water so that light from the source is not visible from the surface. The minimum diameter of the disc is
A2h(μ2−1)1/2B2h(μ2−1)1/2Ch2(μ2−1)1/2Dh(μ2−1)1/2
A squre hole of side length l is made at a depth of h and a circular hole is made at a depht of 4 h from the surface of water in a water tank kept on a horizontal surface (where l ltlt h). Find the radius r of the circular hole if equal amount of water comes out of the vessel through the holes per second.
View SolutionA tank is filled up to a height 2H with a liquid and is placed on a platform of height H from the ground, the distance x from the ground where a small hole is punched to get the maximum range R is
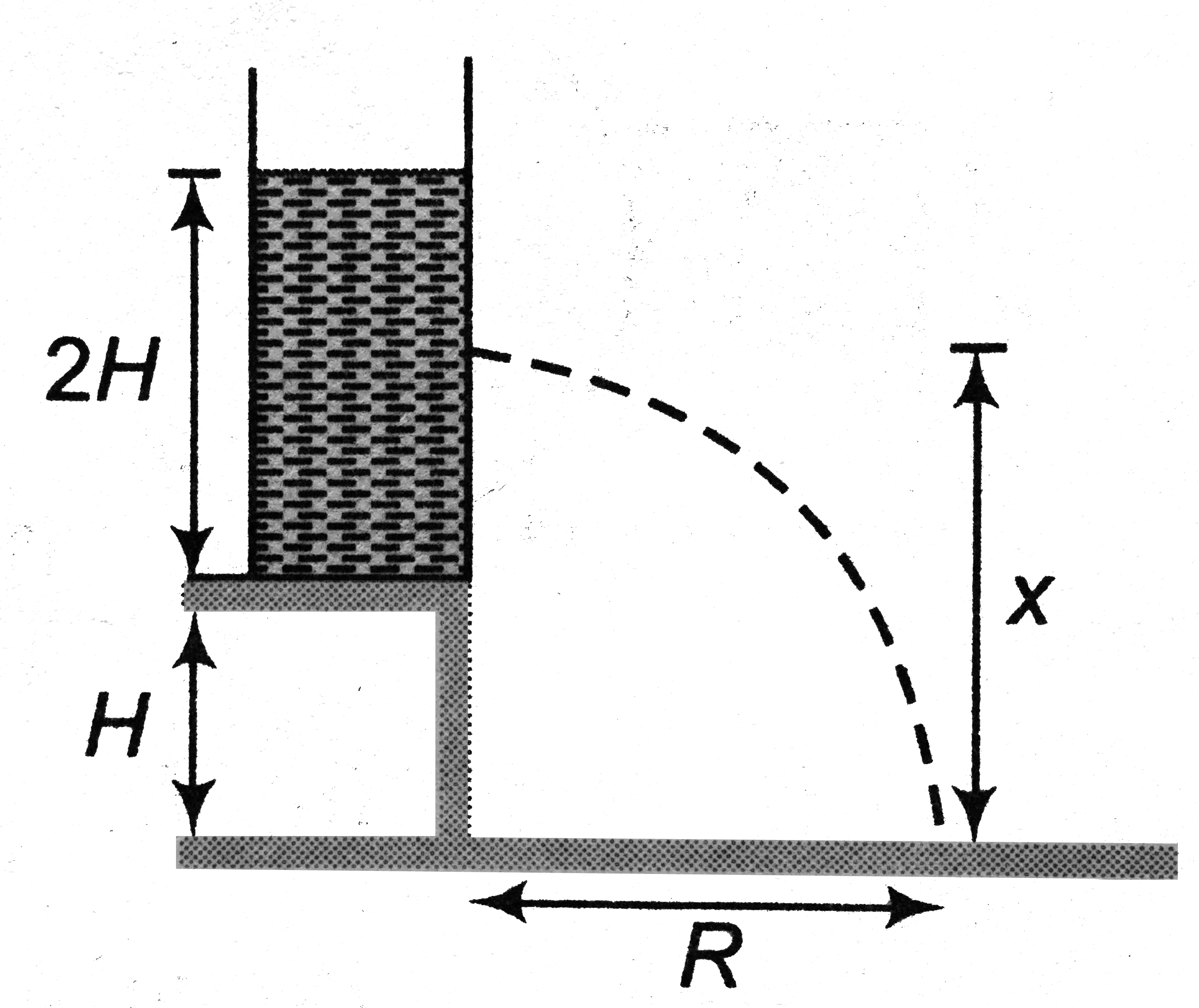 .View Solution
.View SolutionA large tank filled with water to a height h is to be emptied through a small hole at the bottom. The ratio of times taken for the level of water to fall from h to h2 and from h2 to zero is
View SolutionA large open tank has two holes in the wall. One is a square hole of side L at a depth y from the top and the other is a circular hole of radius R at a depth 4y from the top. When the tank is completely filled with water, the quantities of water flowing out per second from both holes are the same. Then, R is equal to
View SolutionA tank if filled with water upto height H. When a hole is made at a distance h below the level of water. What will be the horizontal range of water jet ?
View Solution

