A large open tank has two holes in the wall. One is a square hole of side L at a depth y from the top and the other is a circular hole of radius R at a depth 4y from the top. When the tank is completely filled with water, the quantities of water flowing out per second from both holes are the same. Then, R is equal to
L√2π
2πL
L
L2π
The correct Answer is:A
To solve the problem, we need to find the relationship between the side length L of the square hole and the radius R of the circular hole, given that the quantities of water flowing out per second from both holes are the same.
1. Identify the Areas of the Holes:
- The area of the square hole As is given by:
As=L2
- The area of the circular hole Ac is given by:
Ac=πR2
2. Determine the Velocities of Water Flowing Out:
- The velocity of water flowing out of the square hole at depth y is given by Torricelli's theorem:
Vs=√2gy
- The velocity of water flowing out of the circular hole at depth 4y is:
Vc=√2g(4y)=√8gy=2√2gy
3. Set Up the Flow Rate Equation:
- Since the quantities of water flowing out per second from both holes are the same, we can equate the flow rates:
As⋅Vs=Ac⋅Vc
- Substituting the areas and velocities:
L2⋅√2gy=πR2⋅2√2gy
4. Cancel Common Terms:
- We can cancel √2gy from both sides (assuming y≠0):
L2=πR2⋅2
5. Rearranging the Equation:
- Rearranging gives:
R2=L22π
6. Taking the Square Root:
- Taking the square root of both sides yields:
R=L√2π
Final Answer:
Thus, the value of R is:
R=L√2π
- The area of the square hole As is given by:
As=L2
- The area of the circular hole Ac is given by:
Ac=πR2
- The velocity of water flowing out of the square hole at depth y is given by Torricelli's theorem:
Vs=√2gy
- The velocity of water flowing out of the circular hole at depth 4y is:
Vc=√2g(4y)=√8gy=2√2gy
- Since the quantities of water flowing out per second from both holes are the same, we can equate the flow rates:
As⋅Vs=Ac⋅Vc
- Substituting the areas and velocities:
L2⋅√2gy=πR2⋅2√2gy
- We can cancel √2gy from both sides (assuming y≠0):
L2=πR2⋅2
- Rearranging gives:
R2=L22π
- Taking the square root of both sides yields:
R=L√2π
Thus, the value of R is:
R=L√2π
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Level 2 More Than One Correct10videos
FLUID MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Level 2 Comprehension Based7videos
FLUID MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Subjective Question2videos
FLUID MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - EXPERIMENTSEXERCISE - Subjective15videos
EXPERIMENTS
- BOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE2videos
GENERAL PHYSICS
Similar Questions
A vessel completely filled with water has holes 'A' and 'B' at depths 'h' and '3h' from the top respectively. Hole 'A' is a square of side 'L' and 'B' is circle of radius 'r'. The water flowing out per second from both the holes is same. Then 'L' is equal to
View SolutionA vessel completely filled with water has holes 'A' and 'B' at depths 'h' and '3h' from the top respectively. Hole 'A' is a square of side 'L' and 'B' is circle of radius 'r'. The water flowing out per second from both the holes is same. Then 'L' is equal to
View Solution
Knowledge Check
- Question 1 - Select One
A large open tank has two holes in the wall. One is square hole of side L at a depth y from the top and the other is a circular hole of radius R at a depth of 4y from the top. When the tank is completely filled with water, the quantities of water flowing out per second from both holes are the same. Then R is equal to
AL/√(2π)B2πLCLDL/2π - Question 2 - Select One
A tank filled with fresh water has a hole in its bottom and water is flowing out of it. If the size of the hole is increased, then
Athe volume of water flowing out per second will decreaseBthe velocity of outflow of water remains unchangedCthe volume of water volume out per second remains zeroDBoth (b) and (c)
A tank is filled to a height H. The range of water coming out of a hole which is a depth H/4 from the surface of water level is
View SolutionThe bottom of a cylindrical vessel has a circlular hole of radius r and at depth h below the water] level. If the diameter of the vessel is D, the find then speed with which the water level in the] vessel drops.
View SolutionA squre hole of side length l is made at a depth of h and a circular hole is made at a depht of 4 h from the surface of water in a water tank kept on a horizontal surface (where l ltlt h). Find the radius r of the circular hole if equal amount of water comes out of the vessel through the holes per second.
View SolutionA closed vessel is half filled with water. There is a hole near the top of vessel and air is pumped out from this hole.
View SolutionIf water is ejecting from a hole of radius r at a depth h from the water surface in cylindrical vessel of diameter D, then speed with which the water level in the vessel drops .(Consider area of hole is comparable to area of vessel)
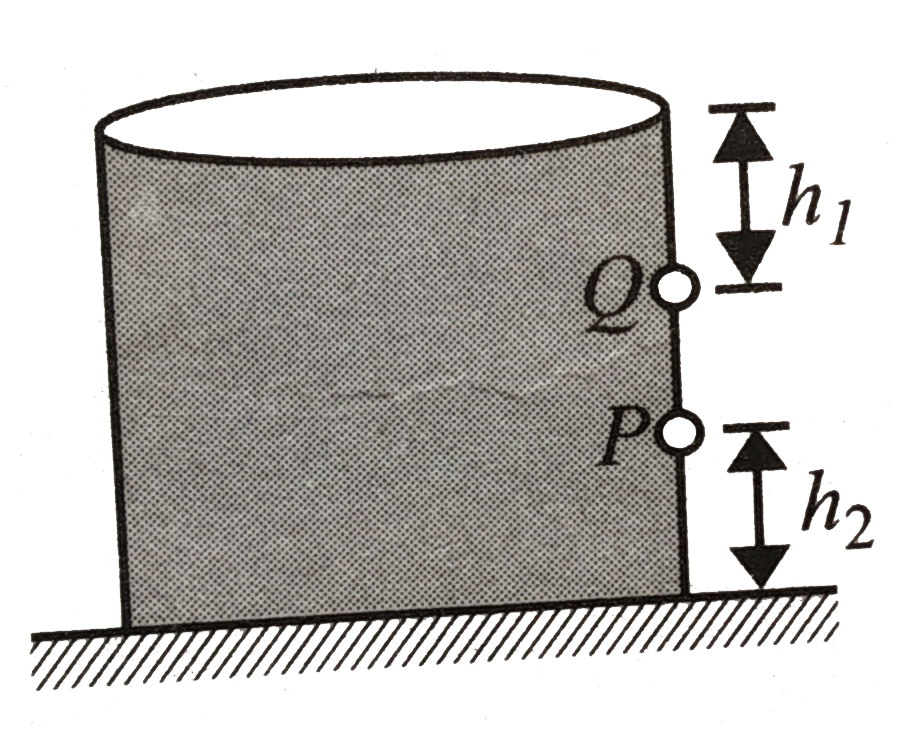
View SolutionIn a cylindrical water tank there are two small holes Q and P on the wall at a depth of h1 from the upper level of water and at a height of h2 from the lower end of the tank, respectively, as shown in the figure. Water coming out from both the holes strike the ground at the same point. The ratio of h1 and h2 is
 View Solution
View Solution

