Question

An ideal gas is initially at temperature T and volume V. Its volume is increased by $\Delta V$ due to an increase in temperature $\Delta T,$ pressure remaining constant. The quantity $\delta =\frac{\Delta V}{V\Delta T}$ varies with temperature as

Options

A.
B.
C.
D.

Answer

C

Explanation

To solve the problem step by step, we will use the ideal gas law and the relationship between volume, temperature, and pressure.

Step 1: Understand the Ideal Gas Law
The ideal gas law is given by the equation:
\( PV = nRT \)
where:
- \( P \) = pressure
- \( V \) = volume
- \( n \) = number of moles of gas
- \( R \) = universal gas constant
- \( T \) = temperature

Step 2: Analyze the Situation
We are given that:
- The initial volume of the gas is \( V \).
- The volume increases by \( \Delta V \) due to an increase in temperature \( \Delta T \).
- The pressure remains constant.

Step 3: Apply the Ideal Gas Law
Since the pressure is constant, we can express the change in volume as:
\( P \Delta V = nR \Delta T \)

Step 4: Rearranging the Equation
Dividing both sides by \( PV \) gives:
\( \frac{\Delta V}{V} = \frac{nR \Delta T}{PV} \)

Step 5: Substitute for Pressure
From the ideal gas law, we can express pressure as:
\( P = \frac{nRT}{V} \)
Substituting this into the equation gives:
\( \frac{\Delta V}{V} = \frac{nR \Delta T}{\left(\frac{nRT}{V}\right)V} \)

Step 6: Simplifying the Equation
This simplifies to:
\( \frac{\Delta V}{V} = \frac{\Delta T}{T} \)
Thus, we can express the change in volume per unit volume and temperature as:
\( \frac{\Delta V}{V \Delta T} = \frac{1}{T} \)

Step 7: Define the Quantity \( \delta \)
Let:
\( \delta = \frac{\Delta V}{V \Delta T} \)
From our previous step, we have:
\( \delta = \frac{1}{T} \)

Step 8: Analyze the Variation of \( \delta \) with Temperature
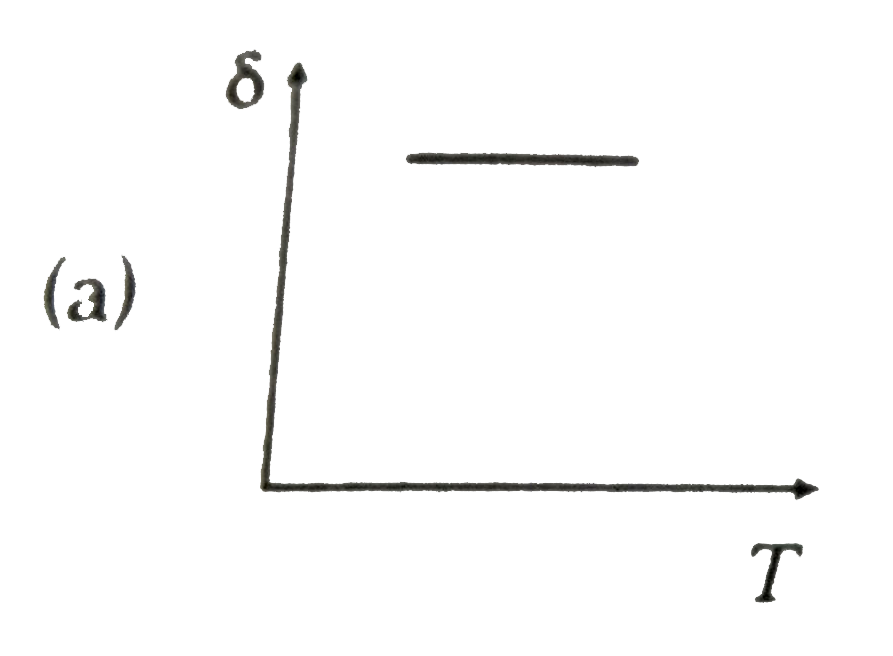
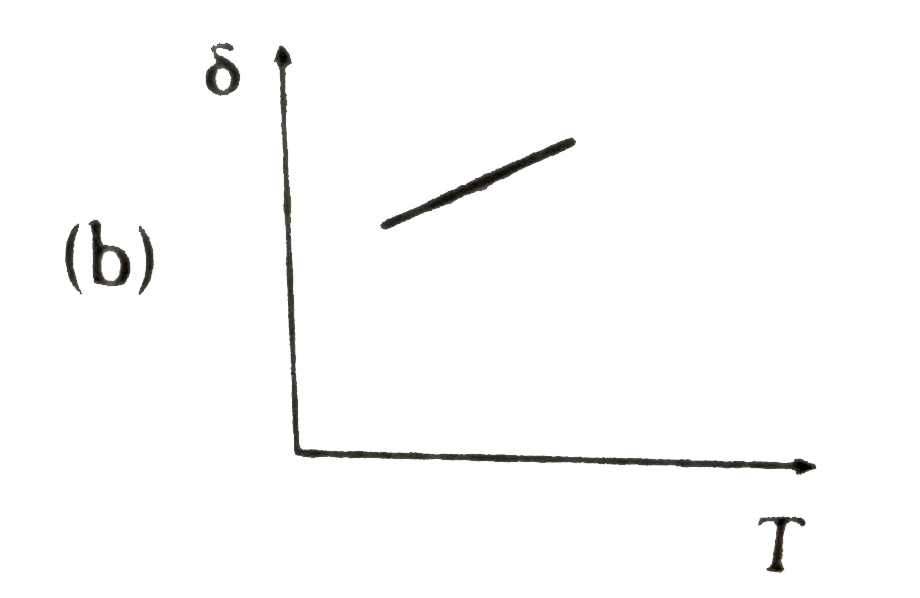
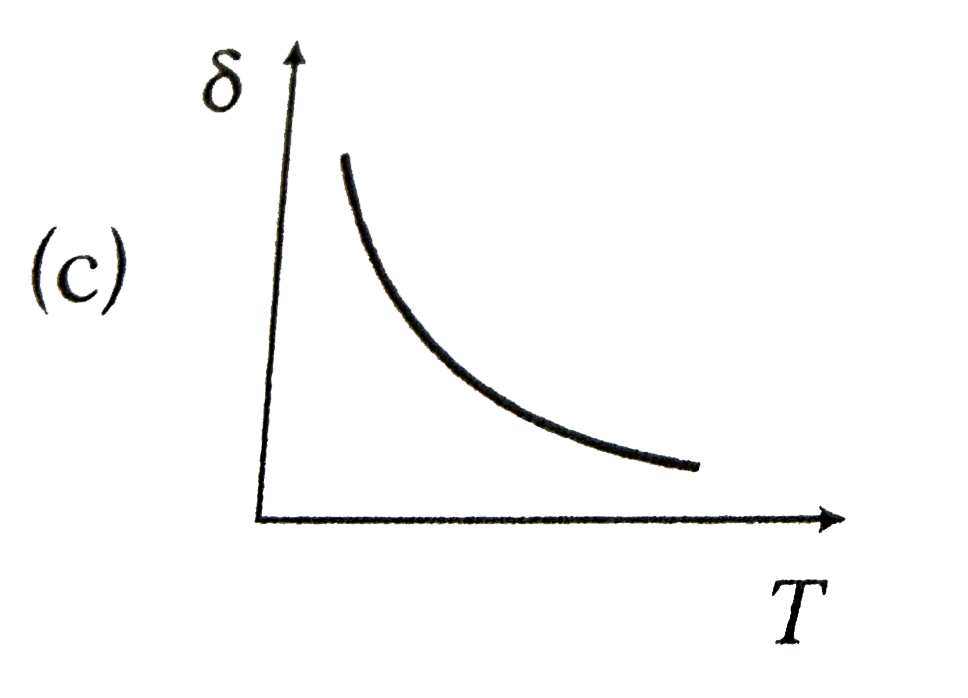
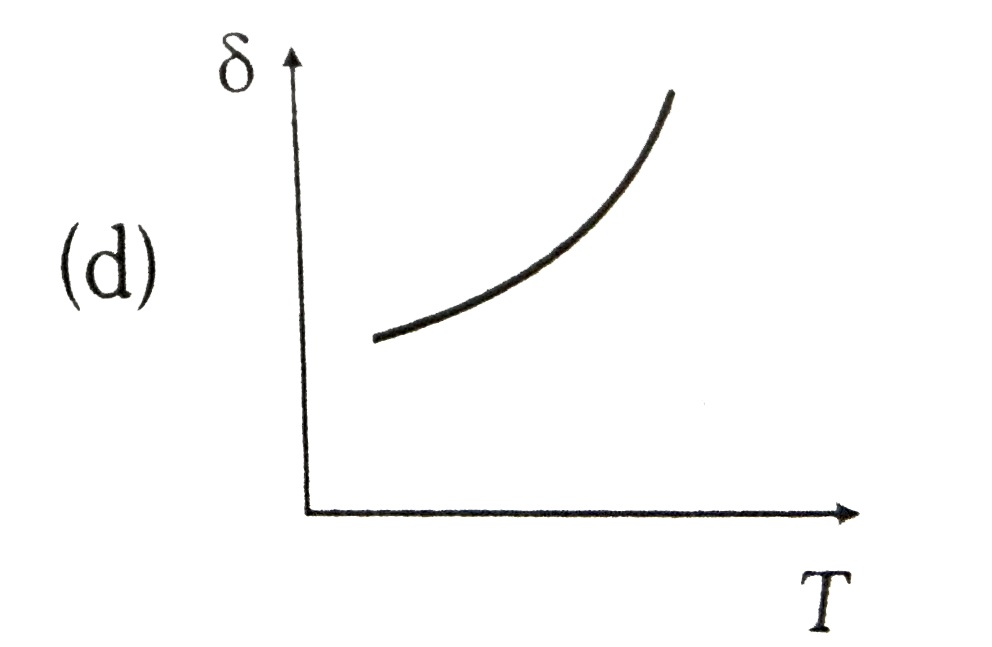
As \( T \) increases, \( \delta \) decreases, indicating an inverse relationship. Therefore, \( \delta \) varies with temperature as:
\( \delta \propto \frac{1}{T} \)

Step 9: Graphical Representation
The relationship \( \delta T = \text{constant} \) indicates that if we plot \( \delta \) against \( T \), we will get a rectangular hyperbola.

Conclusion
Thus, the quantity \( \delta = \frac{\Delta V}{V \Delta T} \) varies with temperature \( T \) as \( \delta \propto \frac{1}{T} \), and the correct option is that the graph of \( \delta \) versus \( T \) is a rectangular hyperbola.