A 20volts AC is applied to a circuit consisting of a resistance and a coil with negligible resistance. If the voltage across the resistance is 12V, the voltage across the coil is
16 V
10 V
8 V
6 V
The correct Answer is:A
To solve the problem, we need to find the voltage across the coil (inductor) in the given AC circuit. We know the total applied voltage and the voltage across the resistance. Let's break down the solution step by step.
Step 1: Identify the given values
- Total applied AC voltage (V) = 20 volts
- Voltage across the resistance (Vr) = 12 volts
Step 2: Use the relationship in an AC circuit
In an AC circuit with a resistor (R) and an inductor (L), the total voltage (V) can be related to the voltages across the resistor (Vr) and the inductor (Vl) using the Pythagorean theorem. This is because the voltages are not in phase.
The relationship is given by:
V2=Vr2+Vl2
Step 3: Substitute the known values
We can substitute the known values into the equation:
202=122+Vl2
Step 4: Calculate the squares
Calculating the squares gives us:
400=144+Vl2
Step 5: Rearrange the equation to solve for Vl^2
Now, we can rearrange the equation to isolate Vl2:
Vl2=400−144
Vl2=256
Step 6: Take the square root to find Vl
Now, we take the square root of both sides to find Vl:
Vl=√256
Vl=16 volts
Conclusion
The voltage across the coil (inductor) is 16 volts.
---
- Total applied AC voltage (V) = 20 volts
- Voltage across the resistance (Vr) = 12 volts
In an AC circuit with a resistor (R) and an inductor (L), the total voltage (V) can be related to the voltages across the resistor (Vr) and the inductor (Vl) using the Pythagorean theorem. This is because the voltages are not in phase.
V2=Vr2+Vl2
We can substitute the known values into the equation:
202=122+Vl2
Calculating the squares gives us:
400=144+Vl2
Now, we can rearrange the equation to isolate Vl2:
Vl2=400−144
Vl2=256
Now, we take the square root of both sides to find Vl:
Vl=√256
Vl=16 volts
The voltage across the coil (inductor) is 16 volts.
Similar Questions
In an a.c. circuit consisting of resistance R and inductance L, the voltage across R is 60 volt and that across L is 80 volt.The total voltage across the combination is
View SolutionAn ac source of 50 V (r.m.s value) is connected across a series R - C circuit. If the r.m.s voltage across the resistor is 40 V, then the r.m.s voltage across the capacitor is
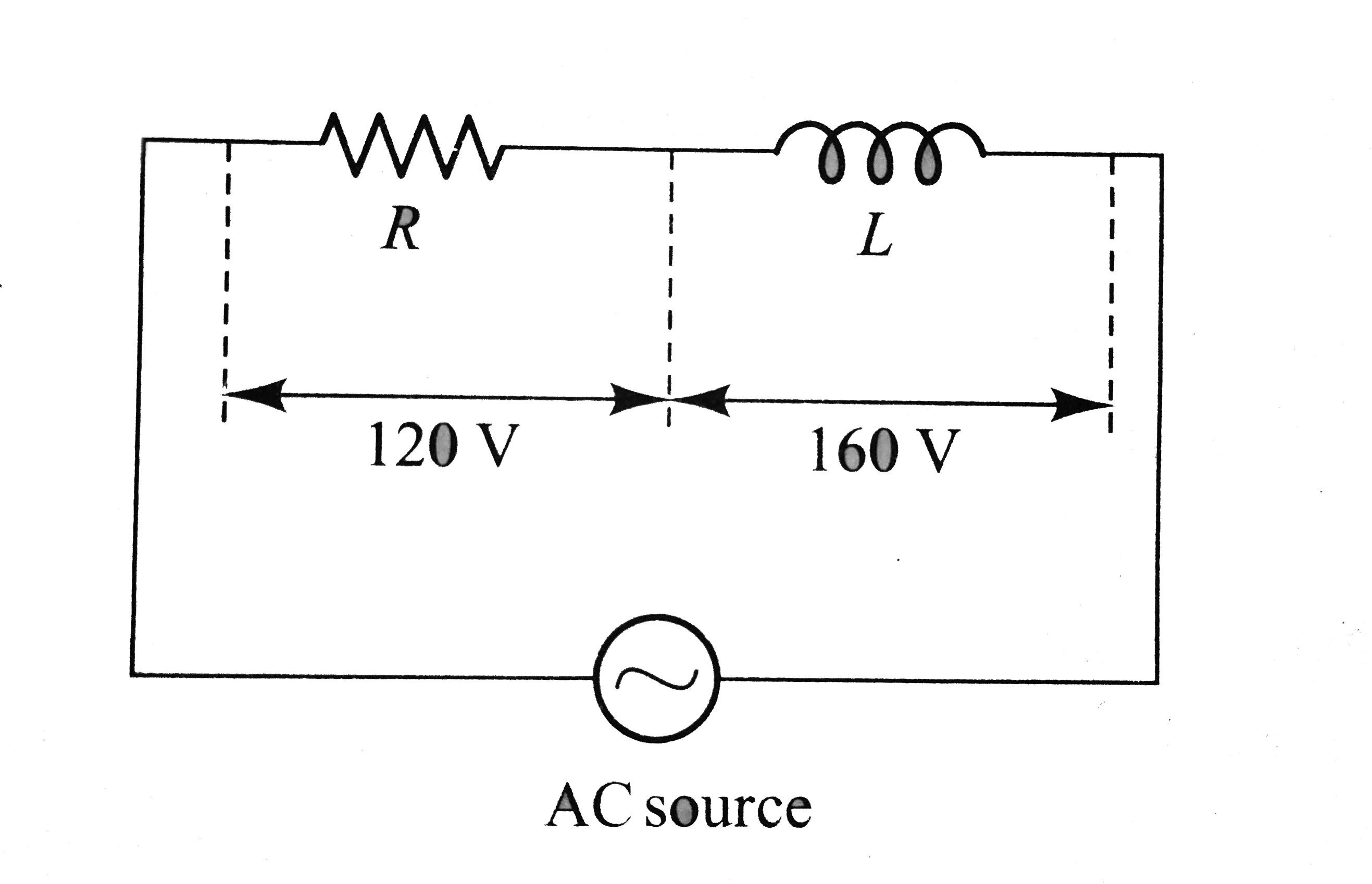
View SolutionThe circuit given in fig. has a resistanceless choke coil L and a resistance R. The voltage across R and L are also given in the figure. The virtual value of the applied voltage is
View SolutionA coil of negligible resistance is connected inseries with 90Ω resistor acorss a 120 V -60 Hz line. A voltmeter reads 36 V across the resistance. Find voltage across the coil and inductance of the coil.
View SolutionAn alternating voltage is connected in series with a resistance R and inductance L if the potential drop across the resistance is 200V and across the inductance is 150V, then the applied voltage is
View SolutionA 50HzAC source of 20V is connected across R and C as shown in figureure.
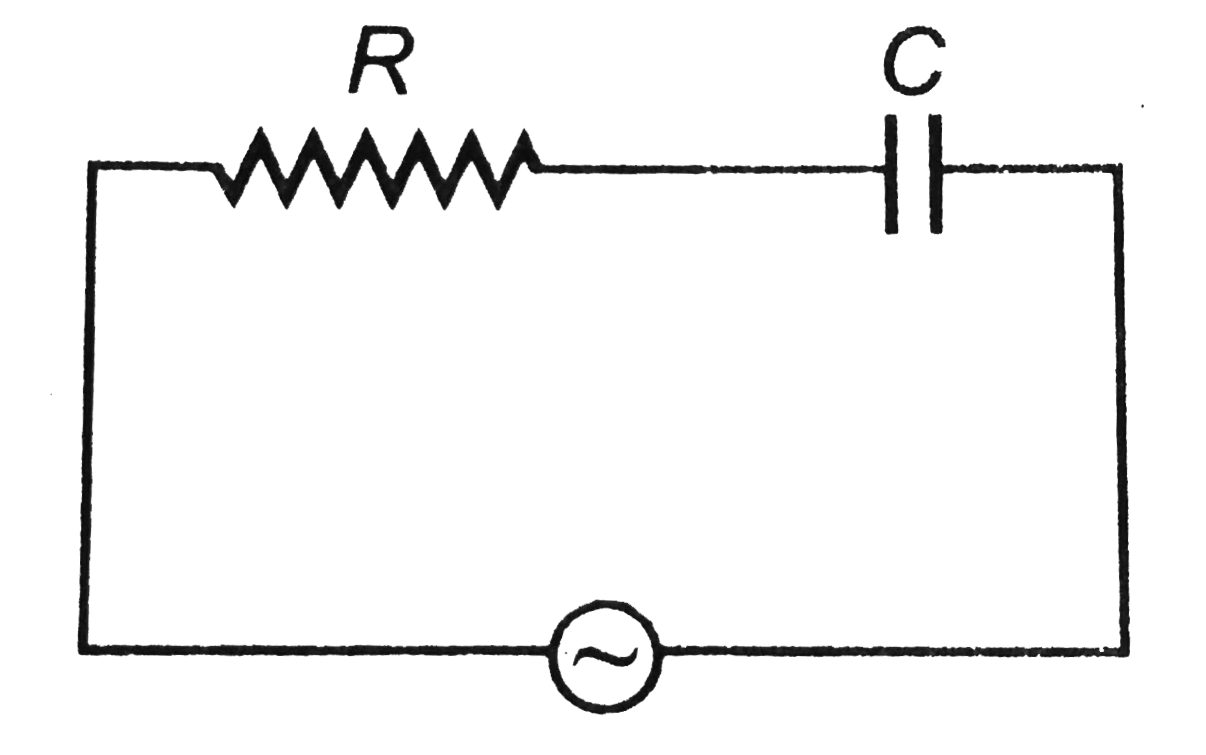
The voltage across R is 12V. The voltage across C is
View SolutionA 50HzAC source of 20V is connected across R and C as shown in figure.
The voltage across R is 12V. The voltage across C is

View SolutionTwo resistances of 100 ohm and 200 ohm are connected in series with a battery of emf 4 volt and negligible internal resistance. A voltmeter of resistance 200 ohm is used to measure voltage across the two resistances separately. Calculate the voltage indicated.
View SolutionA series LCR circuit, has equal resistance capacitive reactance. What is the phase angle between voltage across generator and resistor ?
View SolutionThe cell has an emf of 2V and the internal resistance of this cell is 0.1Ω, it is connected to resistance of 3.9Ω, the voltage across the cell will be
View Solution