Find the force of interaction between two current carrying coils, the distance between the centres of which is much greater than their linear dimensions (Fig. 28.16). 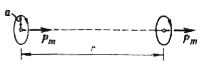
The correct Answer is:F=−6μ0p2m4πr4
It is easily seen that the moving coils hanging freely will arrange themselves so that their planes will be perpendicular to their common axis. The force of interaction is F=pmdBdx Noting that
dBdx=6μ0pmx4π(a2+x2)5/2
(see Problem 27.11) and that according to the condition of the problem x≈r≥≥a, we have dBdx=6μ0pm4πr4
The force of interaction is F=pmdBdx=−6μ0p2m4πr4
It is quite similar to the force of interaction between electric dipoles.
समान प्रश्न
Magnetic force between two current carrying wires
View SolutionThe parameters of two small identical current-carrying coils are as follows: the radius of winding is 20 mm, the number of turns is 10^3, the current is 0.5 A. the distance between the coils is 300 mm. What is the force of interaction between the coils?
View SolutionFind the force of interaction between two current carrying coils, the distance between the centres of which is much greater than their linear dimensions (Fig. 28.16).
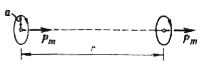
View SolutionThe parameters of two small identical current-carrying coils are as follows: the radius of winding is 20 mm, the number of turns is 10^3, the current is 0.5 A. the distance between the coils is 300 mm. What is the force of interaction between the coils?
View SolutionIf the distance between two parallel current carrying wire is doubled. What is the force between them?
View SolutionFind the force of interaction between the bodies as shown in fig. Blocks are in contact.

View SolutionTwo current carrying coil having radius 'r' are seperated by distance 'd' as shown in diagram. If rlt ltd . Then find the force between two ring. Current in both the ring is clockwise and equal to i.
View Solution
