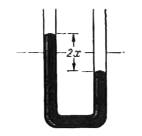
Mercury fills a glass tube Fig. so that the total column is 20 cm long. The tube is then rocked, so that the mercury begins to oscillate. Find the frequency and the period of the vibrations.
The correct Answer is:1.6Hz;0.63s.
AS may be seen from Fig the force which restores the body to its equilibrium positions is F=ρgΔV=2ρgSx. Since this force is proportional to the displacement, the natural frequency of the vibrations can be found from the formula ω0=√k/m. Here k=F/x=2ρgS,m=ρSl.
Hence ω0=√2g/l
समान प्रश्न
An 80 cm long glass tube opened from both sides is immersed in half mercury. If the top end of the tube is closed, it is taken out of the mercury. A column of 20 cm long mercury is formed inside the tube. Atmospheric pressure (mercury) will be in cm
View SolutionThe meniscus of mercury in a glass tube is :
View SolutionThe meniscus of mercury in a glass tube is :
View SolutionIn a vertical U - tube a column of mercury oscillates simple harmonically. If the tube contains 1kg of mercury and 1cm of mercury column weighs 20 g , then the period of oscillation is
View SolutionA glass tube 80 cm long and open ends is half immersed in mercury. Then the top of the tube is closed and it is taken out of the mercury. A column of murcury 20 cm long then remains in the tube. The atmospheric (in cm of Hg) is (assume temperature to be constant )
View SolutionMercury fills a glass tube Fig. so that the total column is 20 cm long. The tube is then rocked, so that the mercury begins to oscillate. Find the frequency and the period of the vibrations.
View SolutionThe meniscus of mercury in a glass tube is:
View Solution
