Question

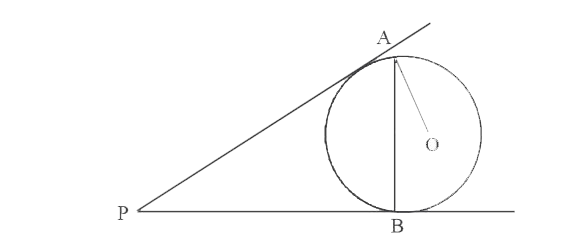
The tangents at two points A and B on the circle with centre O intersect at P. If in quadrilateral PAOB, $\angle AOB:\angle APB=5:1$, then measure of $\angle APB$ is :

Options

A. `30^(@)`
B. `60^(@)`
C. `45^(@)`
D. `15^(@)`

Answer

A

Explanation

To solve the problem step by step, we will follow the reasoning provided in the video transcript.

1. Understanding the Problem:
We have a circle with center O, and two tangents PA and PB drawn from an external point P. The angles we need to consider are ∠AOB and ∠APB, and we know the ratio of these angles is 5:1.

2. Setting Up the Angles:
Let ∠APB = k. According to the given ratio, ∠AOB = 5k.

3. Using the Properties of Tangents:
Since PA and PB are tangents to the circle at points A and B respectively, we know that:
- ∠PAO = 90° (the angle between the radius OA and the tangent PA)
- ∠PBO = 90° (the angle between the radius OB and the tangent PB)

4. Sum of Angles in Quadrilateral PAOB:
The sum of the angles in a quadrilateral is 360°. Therefore, we can write the equation:
\( \angle PAO + \angle PBO + \angle APB + \angle AOB = 360° \)
Substituting the known values, we have:
\( 90° + 90° + k + 5k = 360° \)

5. Simplifying the Equation:
Combine like terms:
\( 180° + 6k = 360° \)

6. Isolating k:
Subtract 180° from both sides:
\( 6k = 360° - 180° \)
\( 6k = 180° \)

7. Solving for k:
Divide both sides by 6:
\( k = \frac{180°}{6} = 30° \)

8. Finding ∠APB:
Since ∠APB = k, we find:
\( \angle APB = 30° \)

Conclusion:
The measure of ∠APB is 30 degrees.