ABCD is a parallelogram AB is divided at P and CD at Q so that AP:PB = 3:2 and CQ:QD = 4:1 if PQ meets AC at R then AR =
27 AC
37AC
47AC
57AC
The correct Answer is:B
To solve the problem, we will follow these steps:
Step 1: Understand the given ratios
We are given that in parallelogram ABCD, the segment AB is divided at point P such that the ratio AP:PB = 3:2. Similarly, the segment CD is divided at point Q such that the ratio CQ:QD = 4:1.
Step 2: Assign variables based on the ratios
Let:
- AP = 3x
- PB = 2x
- CQ = 4y
- QD = y
Step 3: Calculate the lengths of AB and CD
Since AB = AP + PB, we have:
AB = 3x + 2x = 5x
Similarly, for CD:
CD = CQ + QD = 4y + y = 5y
Step 4: Establish the relationship between x and y
Since ABCD is a parallelogram, opposite sides are equal. Therefore:
AB = CD
5x = 5y
This implies that x = y.
Step 5: Substitute y with x
Now, we can express CQ and QD in terms of x:
- CQ = 4x
- QD = x
Step 6: Identify similar triangles
When line PQ intersects AC at point R, triangles ARP and CRQ are formed. We can say that these triangles are similar.
Step 7: Set up the proportion based on the similarity of triangles
From the similarity of triangles ARP and CRQ, we can write:
APCQ=ARCR
Substituting the values we have:
3x4x=ARCR
This simplifies to:
34=ARCR
Step 8: Express CR in terms of AR
Let AR = 3k and CR = 4k for some k. Then:
AC=AR+CR=3k+4k=7k
Step 9: Find AR in terms of AC
Now we can express AR as a fraction of AC:
ARAC=3k7k=37
Thus, we have:
AR=37×AC
Conclusion
The length of AR is 37 of the length of AC.
We are given that in parallelogram ABCD, the segment AB is divided at point P such that the ratio AP:PB = 3:2. Similarly, the segment CD is divided at point Q such that the ratio CQ:QD = 4:1.
Let:
- AP = 3x
- PB = 2x
- CQ = 4y
- QD = y
Since AB = AP + PB, we have:
AB = 3x + 2x = 5x
CD = CQ + QD = 4y + y = 5y
Since ABCD is a parallelogram, opposite sides are equal. Therefore:
AB = CD
5x = 5y
This implies that x = y.
Now, we can express CQ and QD in terms of x:
- CQ = 4x
- QD = x
When line PQ intersects AC at point R, triangles ARP and CRQ are formed. We can say that these triangles are similar.
From the similarity of triangles ARP and CRQ, we can write:
APCQ=ARCR
Substituting the values we have:
3x4x=ARCR
This simplifies to:
34=ARCR
Let AR = 3k and CR = 4k for some k. Then:
AC=AR+CR=3k+4k=7k
Now we can express AR as a fraction of AC:
ARAC=3k7k=37
Thus, we have:
AR=37×AC
The length of AR is 37 of the length of AC.
Topper's Solved these Questions
- BOOK - ADVANCED MATHS BY ABHINAY MATHS ENGLISHCHAPTER - QUADRILATERALEXERCISE - EXERCISE48videos
QUADRILATERAL
- BOOK - ADVANCED MATHS BY ABHINAY MATHS ENGLISHCHAPTER - PROFIT & LOSSEXERCISE - Questions200videos
PROFIT & LOSS
- BOOK - ADVANCED MATHS BY ABHINAY MATHS ENGLISHCHAPTER - RATIO & PROPORTIONEXERCISE - QUESTIONS113videos
RATIO & PROPORTION
Similar Questions
In the figure, ABCD is a parallelogram and BC is produced to a point Q such that AD = CQ. If AQ intersect DC at P, show that ar(ΔBPC) = ar(ΔDPQ).
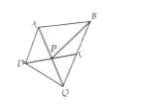 View Solution
View SolutionIn the given figure, ABCD is a parallelogram. Point P is at BC such that PB : PC = 1 : 2. The extended DP and extended AB meets at point Q. If the area of BPQ is 20 unit2 then find the area of ΔDCP.
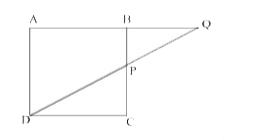 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A transversal cuts side AB and AD and diagonal AC of parallelogram ABCD in points P,Q and R respectively such that AP: PB =1 :2 and AQ: QD=2:3 . Find the ratio AR : RC
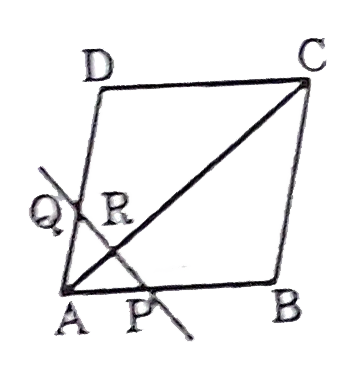 A72B37C27D29
A72B37C27D29 - Question 2 - Select One
ABCD is a parallelogram. P is a point on AD such that AP=13AD and Q is a point on BC such that CQ=13BP . Prove that AQCP is a parallelogram.
ArectangleBsquareCparallelogramDrhombus - Question 3 - Select One
ABCD is a parallelogram. If P be a point on CD such that AP=AD , then the measure of ∠PAB+∠BCD is:
A180∘B225∘C240∘D135∘
In the given figure, ABCD is a parallelogram. Point P is at BC such that PB : PC = 1 : 2. The extended DP and extended AB meets at point Q. If the area of BPQ is 20 unit2 then find the area of ΔDCP.
 View Solution
View SolutionABCD is a parallelogram, P is the mid point of AB. BD and CP intersects at Q such that CQ:QP=3:1. If ar(ΔPBQ)=10cm2, find the area of parallelogram ABCD.
View SolutionABCD is a / gram. P and Q are two points on AB and CD such that AP : PB = 2:3 , DQ = QC = 4 : 1 Find the ratio of area of Δ DPQ and '// ABCD
View SolutionThe side AB of a parallelogram ABCD is produced to any point P. A line through A and parallel to CP meets CB produced at Q and then parallelogram PBQR is completed as shown in Figure. Show that ar(∣∣gmABCD)=ar(∣∣gmPBQR)
View SolutionABCD is a parallelogram. P is a point on AD such that AP=13AD and Q is a point on BC such that CQ=13BP . Prove that AQCP is a parallelogram.
View Solution

