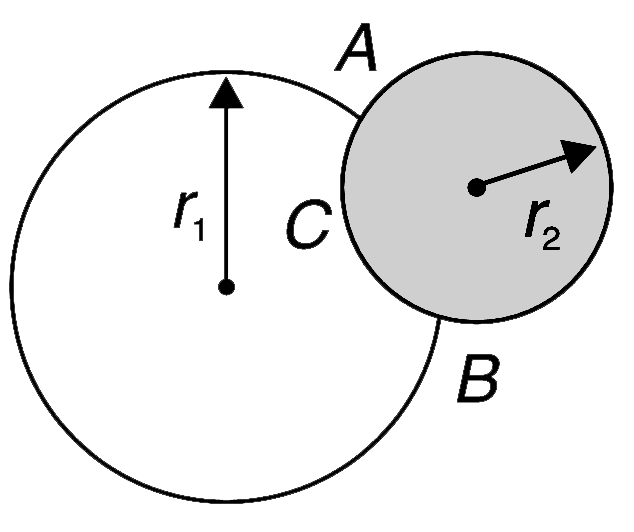
Two soap bubbles with radii of curvature R1andR2 where R2<R1 are brought into contact as shown in Fig. 21.10. What is the radius of curvature of the film between them? What is the contact angle of the films?
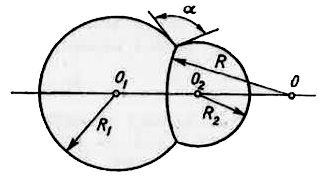
The pressures from the left and from the right are equal, i.e.
Δp1+Δp=Δp2=or4σR1+4σR=4σR2
Here Δ=4σ/R, for the film has two surfaces--the external and the internal. Hence
1R=1R1−1R2
Since at the point of contact of the three films we have a system of three forces of equal magnitude in equilibrium in a plane, the angle
between the forces is found from the condition that they form a closed triangle.
Similar Questions
Knowledge Check
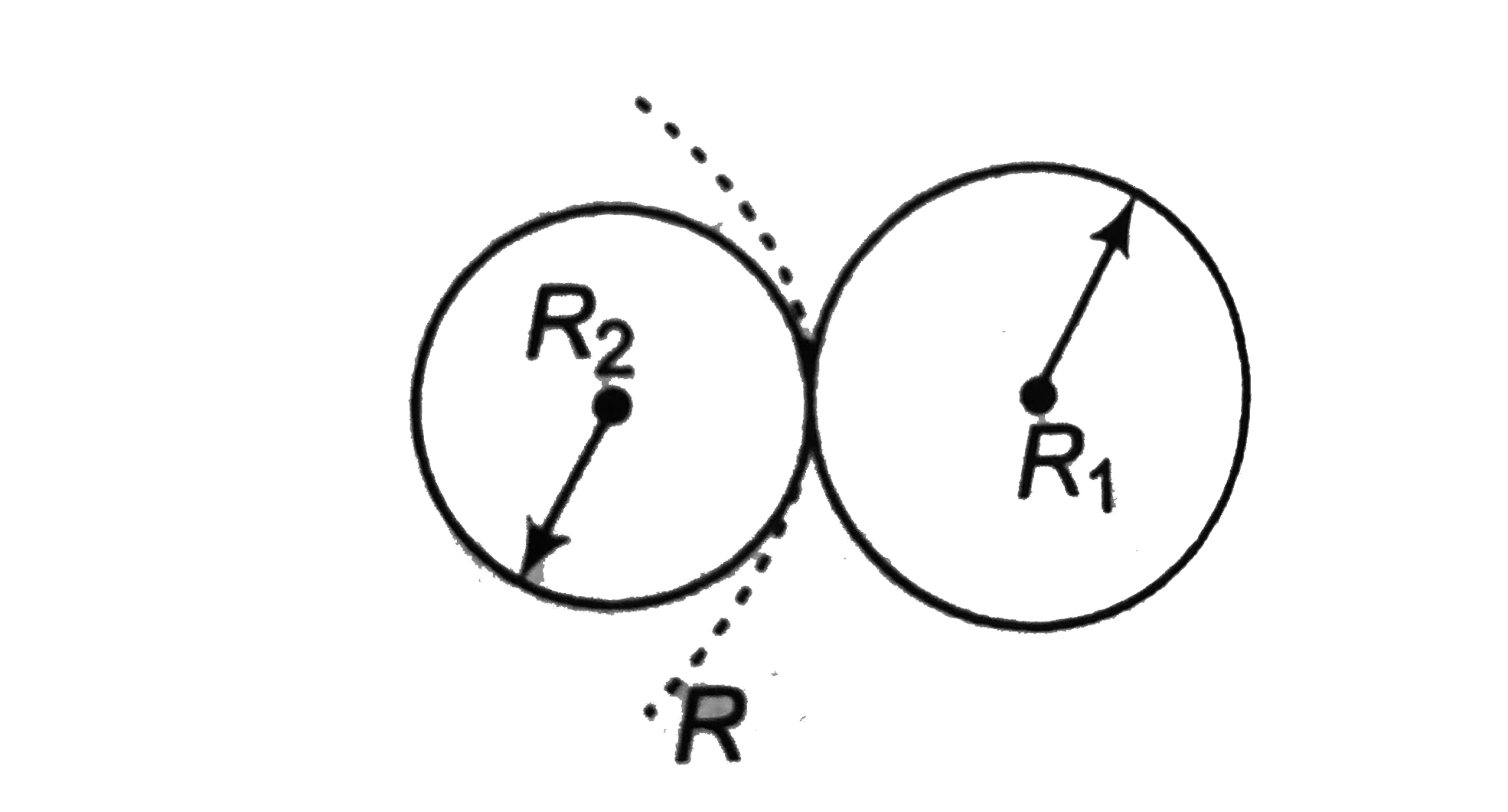
Two soap bubble of different radii R1 and R2(<R1) coalesce to form an interface of radius R as shown in figure. The correct value of R is
 .AR=R1−R2BR=R1−R22C1R=1R2−1R1D1R=1R2+1R1
.AR=R1−R2BR=R1−R22C1R=1R2−1R1D1R=1R2+1R1Prove that if two bubbles of radii r1 and r2(r1<r2) come in contact with each other then the radius of curvature of the common surface r=r1r2r2−r1
Ar2−r1Br2−r1r1r2Cr1r2r2−r1Dr2+r1If two soap bubbles of equal radii r coalesce then the radius of curvature of interface between two bubbles will be
ArB0CinifinityD1/2r
Two soap bubble of radius 2r and 3r are in contact with each other. If the radius of curvature of the interface between the bubble in nr then find the value of n:
View SolutionProve that if two bubbles of radii r1 and r2(r1<r2) come in contact with each other then the radius of curvature of the common surface r=r1r2r2−r1
View SolutionTwo soap bubbles coalesce.It noticed that, whilst joined together, the radii of the two bubbles are a and b where a>b.Then the radius of curvature of interface between the two bubbles will be
View SolutionTwo soap bubbles of radii r1 and r2 equal to 4 cm and 5 cm are touching each other over a common surface S1S2 (shown in figure). Its radius will be
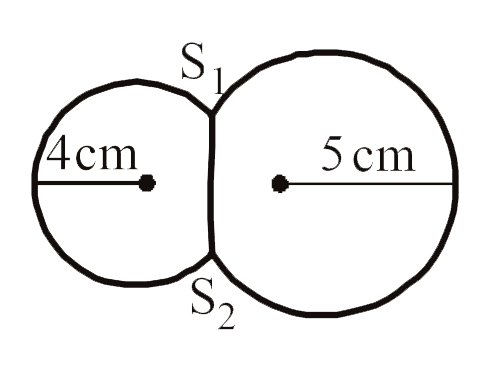
View SolutionTwo soap bubbles of radii r1 and r2 equal to 4 cm and 5 cm are touching each other over a common surface S1S2 (shown in figure). Its radius will be
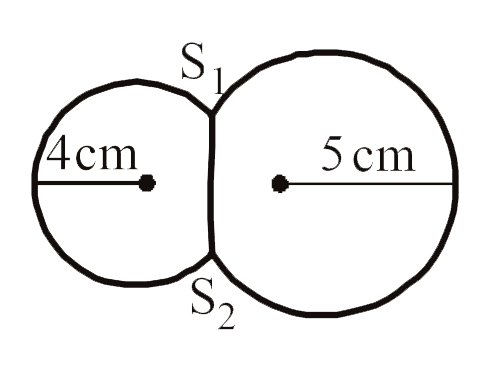
View Solution